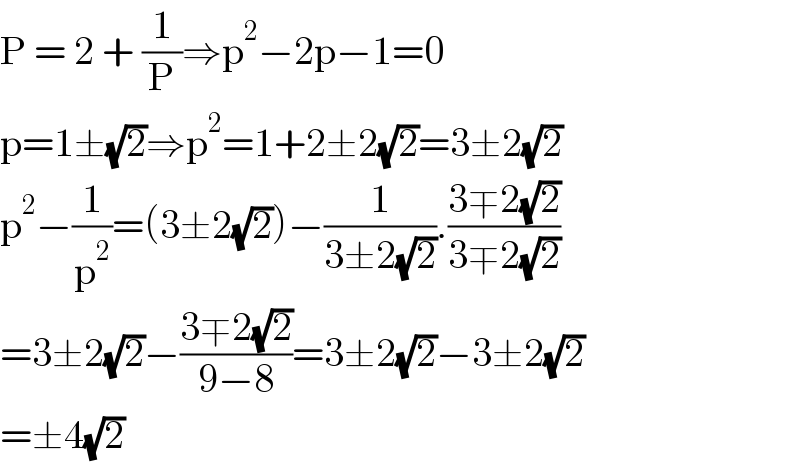Menu Close

If-P-2-1-P-then-what-is-the-answer-of-P-2-1-P-2-

Tinku Tara 0 Comments
Pin




Question Number 134135 by Agnibhoo last updated on 28/Feb/21
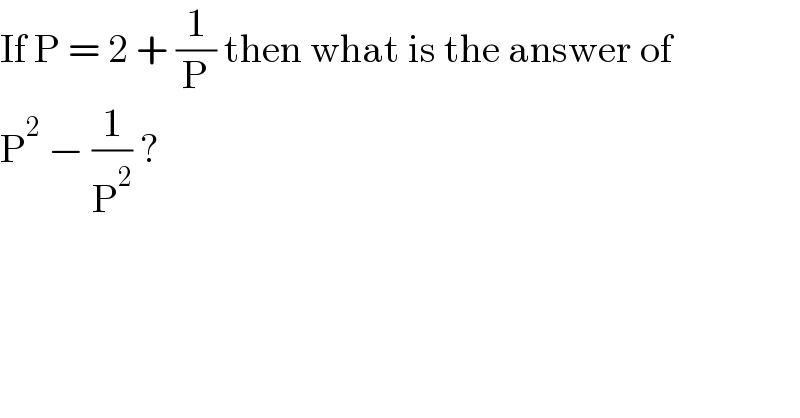
If P = 2 + (1/P) then what is the answer of P^2 − (1/P^2 ) ?
$$\mathrm{If}\:\mathrm{P}\:=\:\mathrm{2}\:+\:\frac{\mathrm{1}}{\mathrm{P}}\:\mathrm{then}\:\mathrm{what}\:\mathrm{is}\:\mathrm{the}\:\mathrm{answer}\:\mathrm{of} \\ $$$$\mathrm{P}^{\mathrm{2}} \:−\:\frac{\mathrm{1}}{\mathrm{P}^{\mathrm{2}} }\:?\:\: \\ $$
Answered by Ñï= last updated on 28/Feb/21
p^2 −(1/p^2 ) =(p−(1/p))(p+(1/p)) =(p−(1/p))[(p−(1/p))^2 +4]^(1/2) =2×(2^2 +4)^(1/2) =4(√2)
$${p}^{\mathrm{2}} −\frac{\mathrm{1}}{{p}^{\mathrm{2}} } \\ $$$$=\left({p}−\frac{\mathrm{1}}{{p}}\right)\left({p}+\frac{\mathrm{1}}{{p}}\right) \\ $$$$=\left({p}−\frac{\mathrm{1}}{{p}}\right)\left[\left({p}−\frac{\mathrm{1}}{{p}}\right)^{\mathrm{2}} +\mathrm{4}\right]^{\mathrm{1}/\mathrm{2}} \\ $$$$=\mathrm{2}×\left(\mathrm{2}^{\mathrm{2}} +\mathrm{4}\right)^{\mathrm{1}/\mathrm{2}} \\ $$$$=\mathrm{4}\sqrt{\mathrm{2}} \\ $$
Answered by bobhans last updated on 28/Feb/21
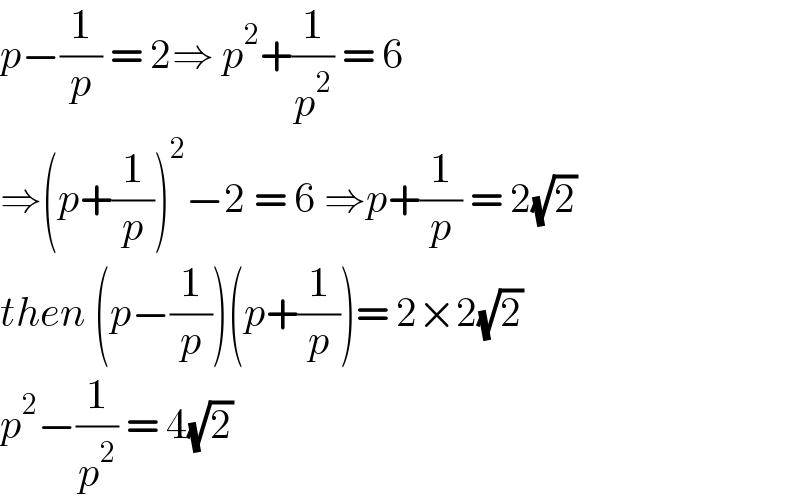
p−(1/p) = 2⇒ p^2 +(1/p^2 ) = 6 ⇒(p+(1/p))^2 −2 = 6 ⇒p+(1/p) = 2(√2) then (p−(1/p))(p+(1/p))= 2×2(√2) p^2 −(1/p^2 ) = 4(√2)
$${p}−\frac{\mathrm{1}}{{p}}\:=\:\mathrm{2}\Rightarrow\:{p}^{\mathrm{2}} +\frac{\mathrm{1}}{{p}^{\mathrm{2}} }\:=\:\mathrm{6} \\ $$$$\Rightarrow\left({p}+\frac{\mathrm{1}}{{p}}\right)^{\mathrm{2}} −\mathrm{2}\:=\:\mathrm{6}\:\Rightarrow{p}+\frac{\mathrm{1}}{{p}}\:=\:\mathrm{2}\sqrt{\mathrm{2}} \\ $$$${then}\:\left({p}−\frac{\mathrm{1}}{{p}}\right)\left({p}+\frac{\mathrm{1}}{{p}}\right)=\:\mathrm{2}×\mathrm{2}\sqrt{\mathrm{2}} \\ $$$${p}^{\mathrm{2}} −\frac{\mathrm{1}}{{p}^{\mathrm{2}} }\:=\:\mathrm{4}\sqrt{\mathrm{2}}\: \\ $$
Answered by mr W last updated on 28/Feb/21
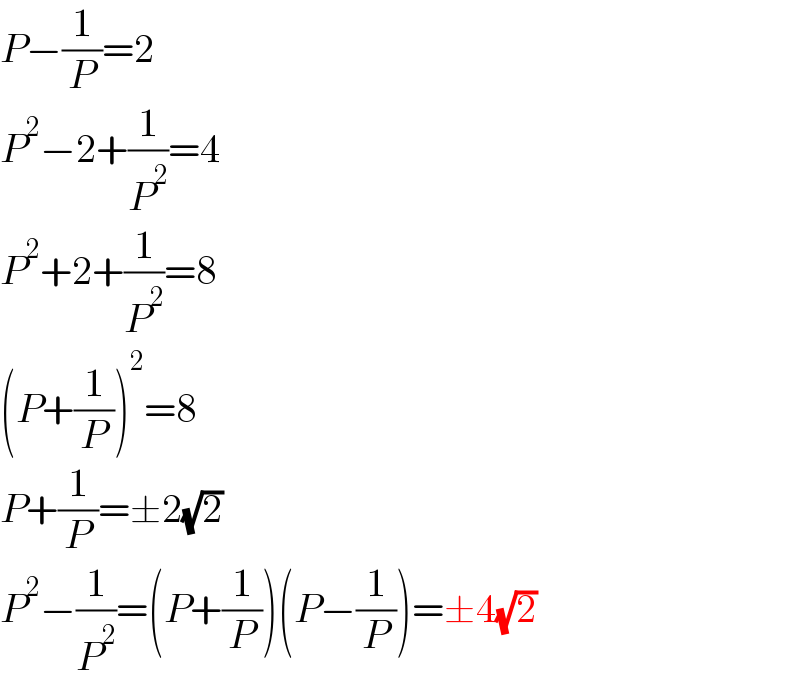
P−(1/P)=2 P^2 −2+(1/P^2 )=4 P^2 +2+(1/P^2 )=8 (P+(1/P))^2 =8 P+(1/P)=±2(√2) P^2 −(1/P^2 )=(P+(1/P))(P−(1/P))=±4(√2)
$${P}−\frac{\mathrm{1}}{{P}}=\mathrm{2} \\ $$$${P}^{\mathrm{2}} −\mathrm{2}+\frac{\mathrm{1}}{{P}^{\mathrm{2}} }=\mathrm{4} \\ $$$${P}^{\mathrm{2}} +\mathrm{2}+\frac{\mathrm{1}}{{P}^{\mathrm{2}} }=\mathrm{8} \\ $$$$\left({P}+\frac{\mathrm{1}}{{P}}\right)^{\mathrm{2}} =\mathrm{8} \\ $$$${P}+\frac{\mathrm{1}}{{P}}=\pm\mathrm{2}\sqrt{\mathrm{2}} \\ $$$${P}^{\mathrm{2}} −\frac{\mathrm{1}}{{P}^{\mathrm{2}} }=\left({P}+\frac{\mathrm{1}}{{P}}\right)\left({P}−\frac{\mathrm{1}}{{P}}\right)=\pm\mathrm{4}\sqrt{\mathrm{2}} \\ $$
Answered by Rasheed.Sindhi last updated on 28/Feb/21
P = 2 + (1/P)⇒p^2 −2p−1=0 p=1±(√2)⇒p^2 =1+2±2(√2)=3±2(√2) p^2 −(1/p^2 )=(3±2(√2))−(1/(3±2(√2))).((3∓2(√2))/(3∓2(√2))) =3±2(√2)−((3∓2(√2))/(9−8))=3±2(√2)−3±2(√2) =±4(√2)
$$\mathrm{P}\:=\:\mathrm{2}\:+\:\frac{\mathrm{1}}{\mathrm{P}}\Rightarrow\mathrm{p}^{\mathrm{2}} −\mathrm{2p}−\mathrm{1}=\mathrm{0} \\ $$$$\mathrm{p}=\mathrm{1}\pm\sqrt{\mathrm{2}}\Rightarrow\mathrm{p}^{\mathrm{2}} =\mathrm{1}+\mathrm{2}\pm\mathrm{2}\sqrt{\mathrm{2}}=\mathrm{3}\pm\mathrm{2}\sqrt{\mathrm{2}} \\ $$$$\mathrm{p}^{\mathrm{2}} −\frac{\mathrm{1}}{\mathrm{p}^{\mathrm{2}} }=\left(\mathrm{3}\pm\mathrm{2}\sqrt{\mathrm{2}}\right)−\frac{\mathrm{1}}{\mathrm{3}\pm\mathrm{2}\sqrt{\mathrm{2}}}.\frac{\mathrm{3}\mp\mathrm{2}\sqrt{\mathrm{2}}}{\mathrm{3}\mp\mathrm{2}\sqrt{\mathrm{2}}} \\ $$$$=\mathrm{3}\pm\mathrm{2}\sqrt{\mathrm{2}}−\frac{\mathrm{3}\mp\mathrm{2}\sqrt{\mathrm{2}}}{\mathrm{9}−\mathrm{8}}=\mathrm{3}\pm\mathrm{2}\sqrt{\mathrm{2}}−\mathrm{3}\pm\mathrm{2}\sqrt{\mathrm{2}} \\ $$$$=\pm\mathrm{4}\sqrt{\mathrm{2}} \\ $$

Pin

Leave a Reply

Your email address will not be published. Required fields are marked *