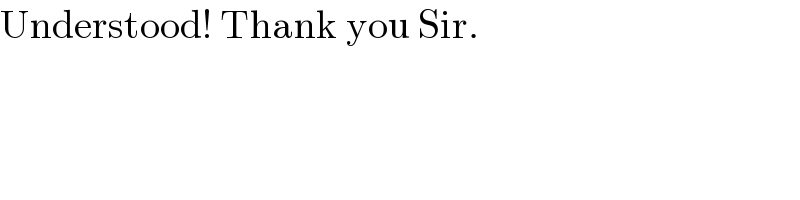If-z-i-2-and-z-0-5-3i-then-the-maximum-value-of-z-0-iz-is-A-7-B-7-C-5-D-9-

Question Number 139394 by EnterUsername last updated on 26/Apr/21

If ∣ z - i ∣⩽ 2 and z_{0} = 5 + 3 i, then the maximum value

of ∣ z_{0} + i z ∣ is

(A) 7 (B) \sqrt{7} (C) 5 (D) 9

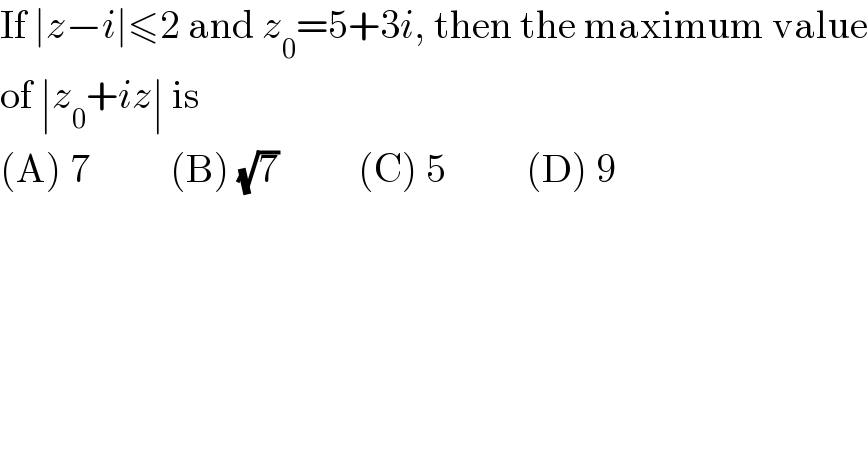
Answered by mr W last updated on 26/Apr/21

z = x + y i

∣ z - i ∣= \sqrt{x^{2} + {(y - 1)}^{2}} ⩽ 2

x^{2} + {(y - 1)}^{2} ⩽ 4

F =∣ z_{0} + i z ∣=∣ 5 - y + (3 + x) i ∣

= \sqrt{{(5 - y)}^{2} + {(3 + x)}^{2}}

= \sqrt{{(x + 3)}^{2} + {(y - 5)}^{2}}

F_{m i n} = \sqrt{{(- 3)}^{2} + {(5 - 1)}^{2}} - 2 = 3

F_{m a x} = \sqrt{{(- 3)}^{2} + {(5 - 1)}^{2}} + 2 = 7

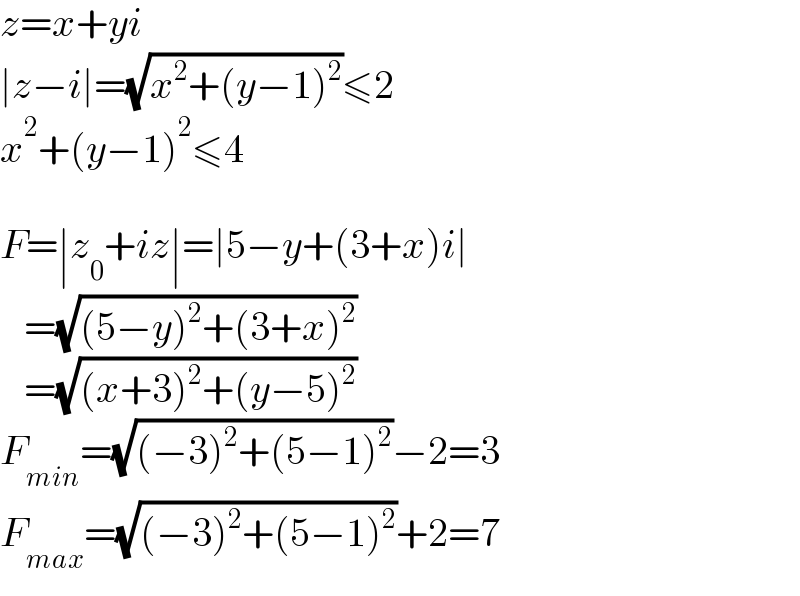
Commented by EnterUsername last updated on 26/Apr/21

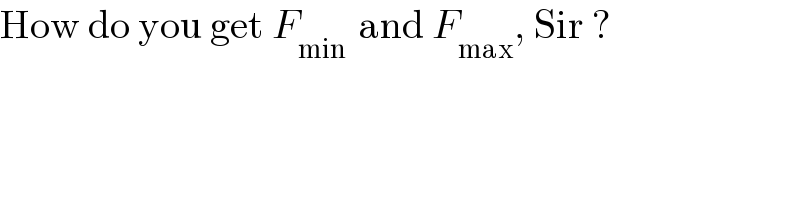
How do you get F_{\min} and F_{\max}, Sir ?

Commented by mr W last updated on 26/Apr/21

F i s t h e d i s t a n c e f r o m p o i n t (x, y) t o

p o i n t (- 3, 5) . p o i n t (x, y) s h o u l d b e

o n t h e c i r c l e x^{2} + {(y - 1)}^{2} ⩽ 2^{2}, a c i r c l e

a t c e n t e r (0, 1) a n d w i t h r a d i u s 2 .

s o t h e m i n i m u m d i s t a n c e F i s t h e

d i s t a n c e f r o m (- 3, 5) t o (0, 1) m i n u s

r a d i u s 2 a n d t h e m a x i m u m d i s t a n c e

F i s t h e d i s t a n c e f r o m (- 3, 5) t o (0, 1)

p l u s r a d i u s 2 .

Commented by EnterUsername last updated on 26/Apr/21

Understood! Thank you Sir .