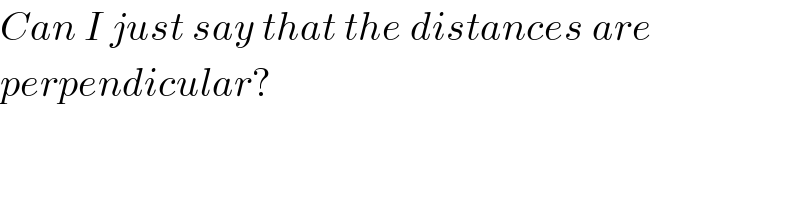Question Number 76455 by Maclaurin Stickker last updated on 27/Dec/19

Commented by MJS last updated on 27/Dec/19

Commented by Maclaurin Stickker last updated on 27/Dec/19

Answered by mr W last updated on 27/Dec/19
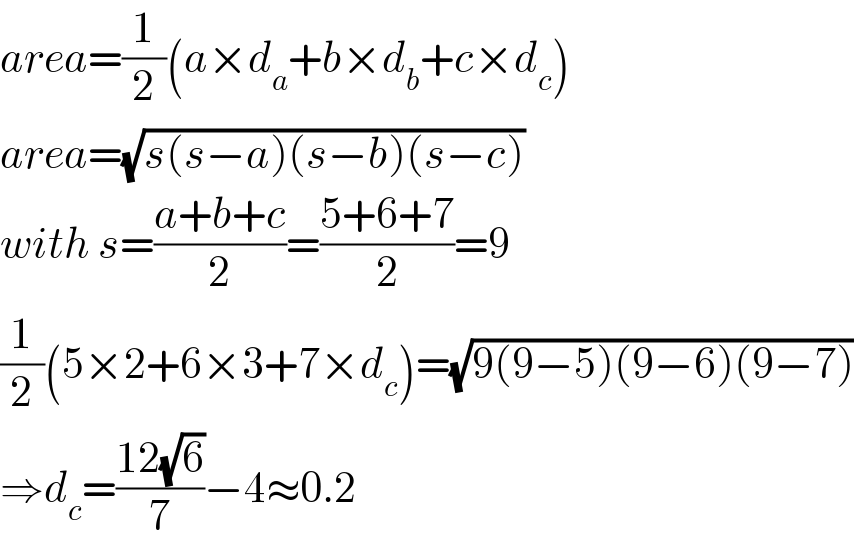
Commented by mr W last updated on 27/Dec/19
Commented by Maclaurin Stickker last updated on 27/Dec/19
Commented by mr W last updated on 27/Dec/19

Commented by mr W last updated on 27/Dec/19
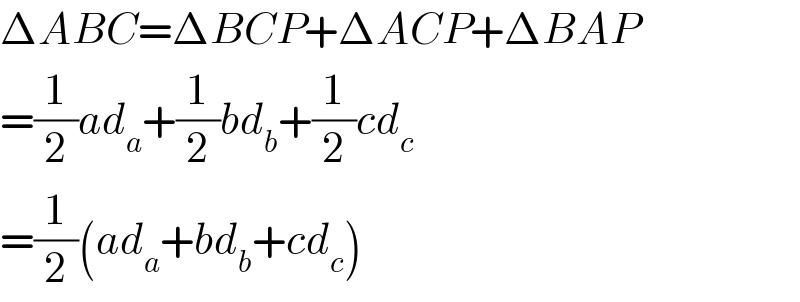
Commented by Maclaurin Stickker last updated on 27/Dec/19