Question Number 12881 by kunalshukla95040 last updated on 05/May/17

$$\frac{{lim}}{{x}\rightarrow\mathrm{0}}\frac{\sqrt{\mathrm{1}−\mathrm{cos}\:{x}}}{{x}} \\ $$$${is}\:{equals}\:{to}. \\ $$
Answered by nume1114 last updated on 05/May/17
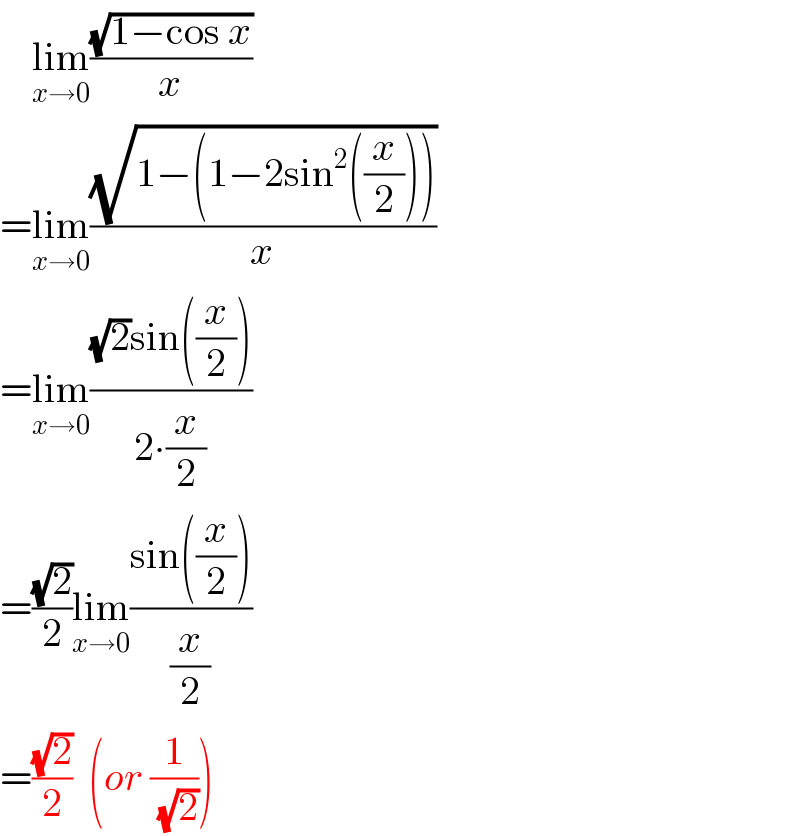
$$\:\:\:\:\underset{{x}\rightarrow\mathrm{0}} {\mathrm{lim}}\frac{\sqrt{\mathrm{1}−\mathrm{cos}\:{x}}}{{x}} \\ $$$$=\underset{{x}\rightarrow\mathrm{0}} {\mathrm{lim}}\frac{\sqrt{\mathrm{1}−\left(\mathrm{1}−\mathrm{2sin}^{\mathrm{2}} \left(\frac{{x}}{\mathrm{2}}\right)\right)}}{{x}} \\ $$$$=\underset{{x}\rightarrow\mathrm{0}} {\mathrm{lim}}\frac{\sqrt{\mathrm{2}}\mathrm{sin}\left(\frac{{x}}{\mathrm{2}}\right)}{\mathrm{2}\centerdot\frac{{x}}{\mathrm{2}}} \\ $$$$=\frac{\sqrt{\mathrm{2}}}{\mathrm{2}}\underset{{x}\rightarrow\mathrm{0}} {\mathrm{lim}}\frac{\mathrm{sin}\left(\frac{{x}}{\mathrm{2}}\right)}{\frac{{x}}{\mathrm{2}}} \\ $$$$=\frac{\sqrt{\mathrm{2}}}{\mathrm{2}}\:\:\left({or}\:\frac{\mathrm{1}}{\:\sqrt{\mathrm{2}}}\right) \\ $$
