Question Number 66640 by hmamarques1994@gmail.com last updated on 18/Aug/19

Commented by kaivan.ahmadi last updated on 18/Aug/19
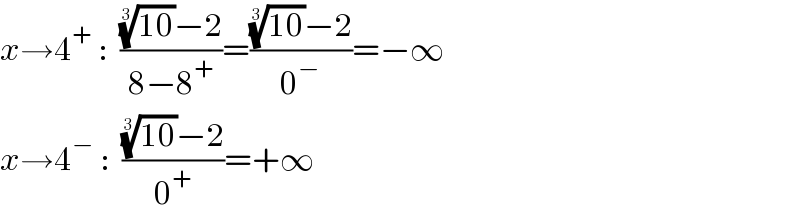
Commented by kaivan.ahmadi last updated on 18/Aug/19
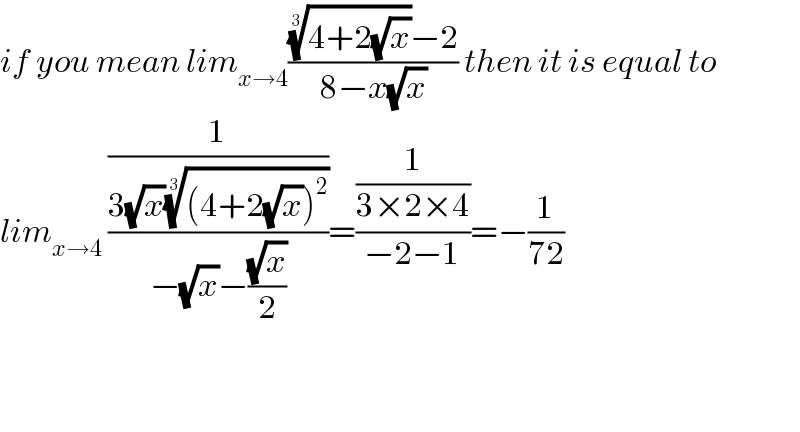
Commented by mathmax by abdo last updated on 18/Aug/19

Commented by hmamarques1994@gmail.com last updated on 18/Aug/19

Commented by Sayantan chakraborty last updated on 18/Aug/19

Commented by mathmax by abdo last updated on 18/Aug/19

