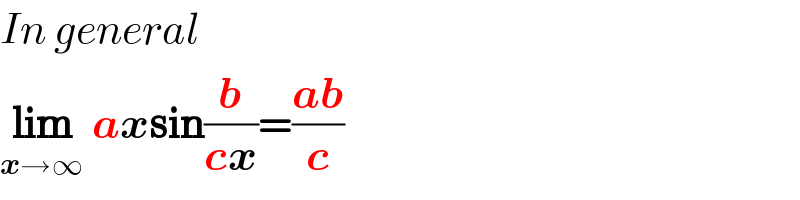lim-x-xsin-pi-x-

Question Number 69809 by RAKESH MANDA last updated on 28/Sep/19

\lim_{x \to \infty} x \sin \frac{π}{x}

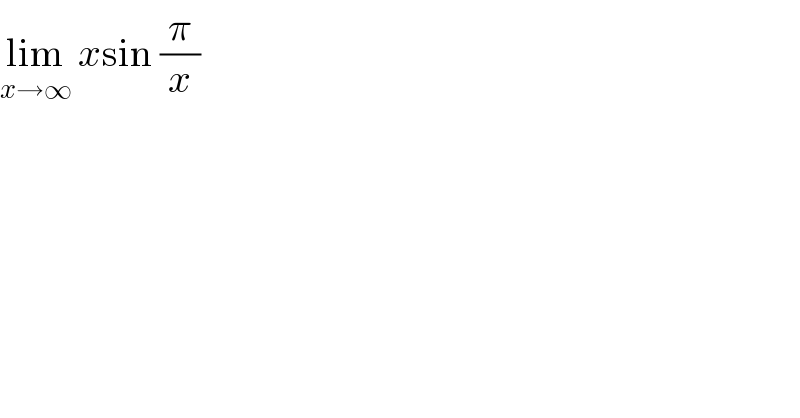
Commented by mr W last updated on 28/Sep/19

\lim_{x \to \infty} x \sin \frac{π}{x}

= π \lim_{x \to \infty} \frac{\sin \frac{π}{x}}{\frac{π}{x}}

= π \lim_{t \to 0} \frac{\sin t}{t}

= π \times 1

= π

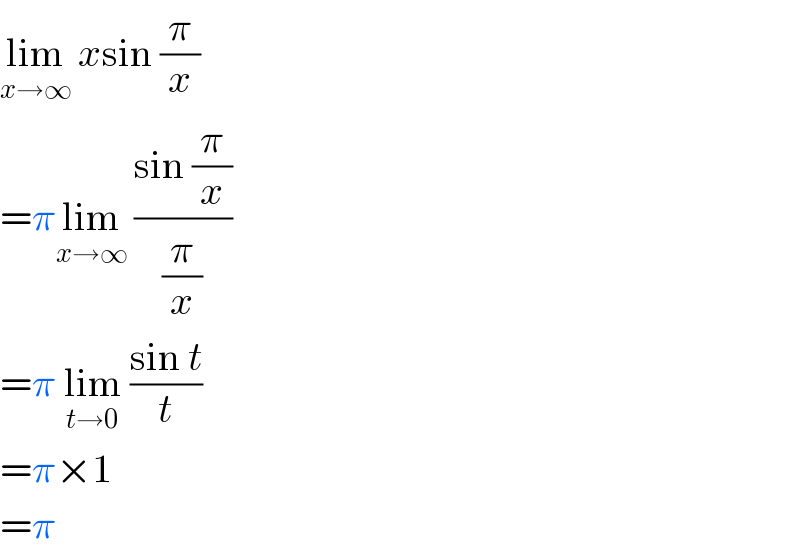
Commented by peter frank last updated on 28/Sep/19

t h a n k y o u

Answered by $@ty@m123 last updated on 28/Sep/19

L e t x = \frac{1}{y}

s o t h a t a s x \to \infty, y \to 0

∴ \lim_{x \to \infty} x \sin \frac{π}{x}

= \lim_{y \to 0} \frac{\sin π y}{y}

= \lim_{y \to 0} \frac{\sin π y}{π y} \times π

= 1 \times π

= π

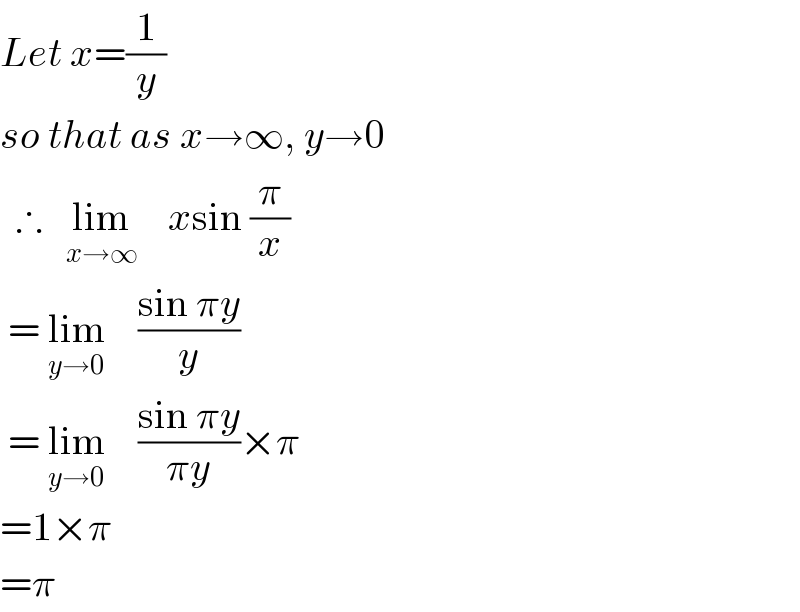
Answered by malwaan last updated on 28/Sep/19

\lim_{x \to \infty} \frac{\sin \frac{π}{x}}{\frac{π}{x}} \times π

= π \times \lim_{t \to 0} \frac{\sin t}{t} = π

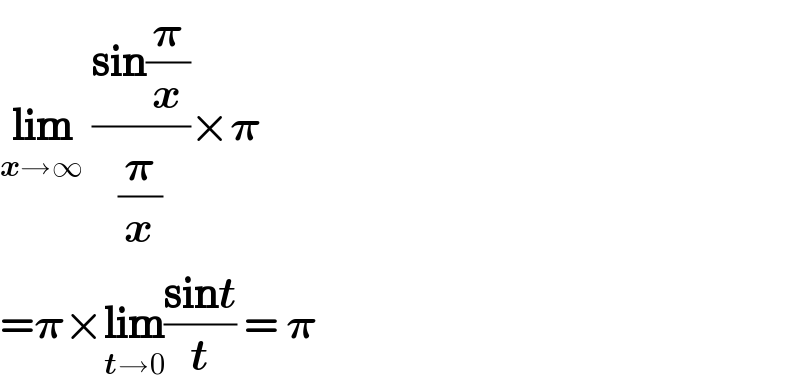
Commented by malwaan last updated on 28/Sep/19

I n g e n e r a l

\lim_{x \to \infty} a x \sin \frac{b}{c x} = \frac{a b}{c}

Facebook Tweet Pin