Nice-Calculus-find-the-value-of-1-ln-1-x-x-2-dx-

Question Number 137953 by mnjuly1970 last updated on 08/Apr/21

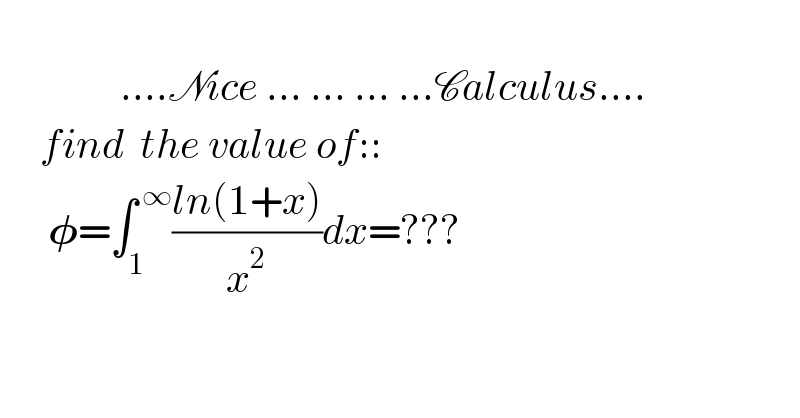
....Nice ... ... ... ...Calculus.... find the value of:: 𝛗=∫_1 ^( ∞) ((ln(1+x))/x^2 )dx=???

$$\:\:\:\:\:\:\:\:\:\:\:\: \\ $$$$\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:….\mathscr{N}{ice}\:…\:…\:…\:…\mathscr{C}{alculus}…. \\ $$$$\:\:\:\:\:{find}\:\:{the}\:{value}\:{of}:: \\ $$$$\:\:\:\:\:\:\boldsymbol{\phi}=\int_{\mathrm{1}} ^{\:\infty} \frac{{ln}\left(\mathrm{1}+{x}\right)}{{x}^{\mathrm{2}} }{dx}=??? \\ $$

Answered by Ñï= last updated on 08/Apr/21

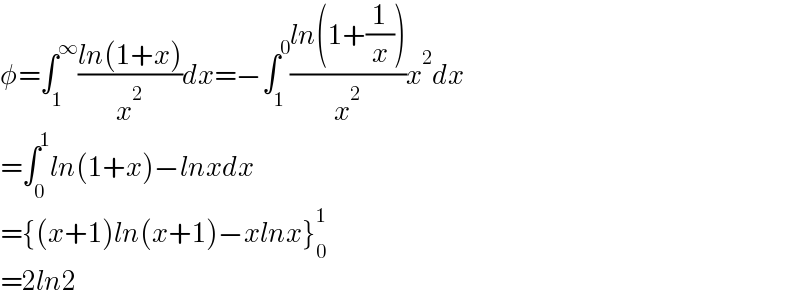
φ=∫_1 ^∞ ((ln(1+x))/x^2 )dx=−∫_1 ^0 ((ln(1+(1/x)))/x^2 )x^2 dx =∫_0 ^1 ln(1+x)−lnxdx ={(x+1)ln(x+1)−xlnx}_0 ^1 =2ln2

$$\phi=\int_{\mathrm{1}} ^{\infty} \frac{{ln}\left(\mathrm{1}+{x}\right)}{{x}^{\mathrm{2}} }{dx}=−\int_{\mathrm{1}} ^{\mathrm{0}} \frac{{ln}\left(\mathrm{1}+\frac{\mathrm{1}}{{x}}\right)}{{x}^{\mathrm{2}} }{x}^{\mathrm{2}} {dx} \\ $$$$=\int_{\mathrm{0}} ^{\mathrm{1}} {ln}\left(\mathrm{1}+{x}\right)−{lnxdx} \\ $$$$=\left\{\left({x}+\mathrm{1}\right){ln}\left({x}+\mathrm{1}\right)−{xlnx}\right\}_{\mathrm{0}} ^{\mathrm{1}} \\ $$$$=\mathrm{2}{ln}\mathrm{2} \\ $$

Commented by mnjuly1970 last updated on 08/Apr/21

$$\:\:{very}\:{nice}\:{solution}…{thank}\:{you} \\ $$$${mr}\:… \\ $$

Answered by mnjuly1970 last updated on 08/Apr/21

f(a)=∫_1 ^( ∞) ((ln(1+ax))/x^2 )dx f ′(a)=∫_1 ^( ∞) (1/(x(1+ax)))dx =∫_1 ^( ∞) ((1/x) −(a/(1+ax)))dx=[ln((x/(1+ax)))]_1 ^∞ =−ln(a)+ln(1+a) ∴ f(a)=a−aln(a)+(1+a)ln(1+a)−(a)+C f(0)=0=C 𝛗=f (1)=1+2ln(2)−1=ln(4) 𝛗=ln(4)...✓

$$\:\:\:\:{f}\left({a}\right)=\int_{\mathrm{1}} ^{\:\infty} \frac{{ln}\left(\mathrm{1}+{ax}\right)}{{x}^{\mathrm{2}} }{dx} \\ $$$$\:\:\:\:\:\:\:\:\:{f}\:'\left({a}\right)=\int_{\mathrm{1}} ^{\:\infty} \frac{\mathrm{1}}{{x}\left(\mathrm{1}+{ax}\right)}{dx} \\ $$$$\:\:\:\:\:\:=\int_{\mathrm{1}} ^{\:\infty} \left(\frac{\mathrm{1}}{{x}}\:−\frac{{a}}{\mathrm{1}+{ax}}\right){dx}=\left[{ln}\left(\frac{{x}}{\mathrm{1}+{ax}}\right)\right]_{\mathrm{1}} ^{\infty} \\ $$$$=−{ln}\left({a}\right)+{ln}\left(\mathrm{1}+{a}\right) \\ $$$$\:\therefore\:{f}\left({a}\right)={a}−{aln}\left({a}\right)+\left(\mathrm{1}+{a}\right){ln}\left(\mathrm{1}+{a}\right)−\left({a}\right)+{C} \\ $$$$\:\:\:{f}\left(\mathrm{0}\right)=\mathrm{0}={C} \\ $$$$\:\:\:\boldsymbol{\phi}={f}\:\left(\mathrm{1}\right)=\mathrm{1}+\mathrm{2}{ln}\left(\mathrm{2}\right)−\mathrm{1}={ln}\left(\mathrm{4}\right) \\ $$$$\:\:\:\:\boldsymbol{\phi}={ln}\left(\mathrm{4}\right)…\checkmark \\ $$

Facebook Tweet Pin

Nice-Calculus-find-the-value-of-1-ln-1-x-x-2-dx-

Leave a Reply Cancel reply