Question Number 8808 by tawakalitu last updated on 28/Oct/16
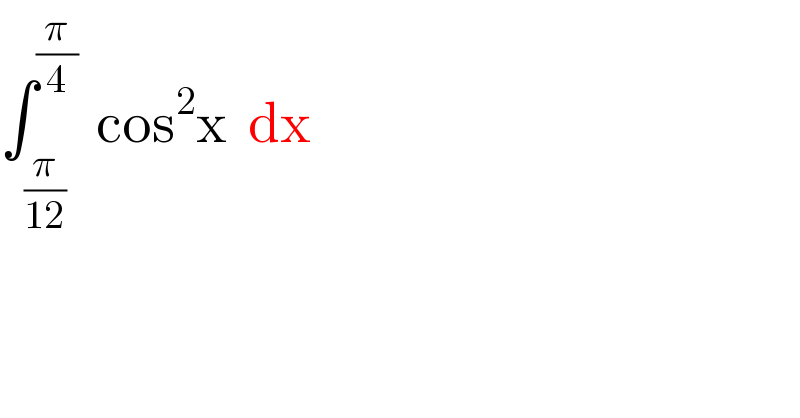
$$\int_{\frac{\pi}{\mathrm{12}}\:} ^{\frac{\pi}{\mathrm{4}}} \:\mathrm{cos}^{\mathrm{2}} \mathrm{x}\:\:\mathrm{dx} \\ $$
Commented by ridwan balatif last updated on 29/Oct/16
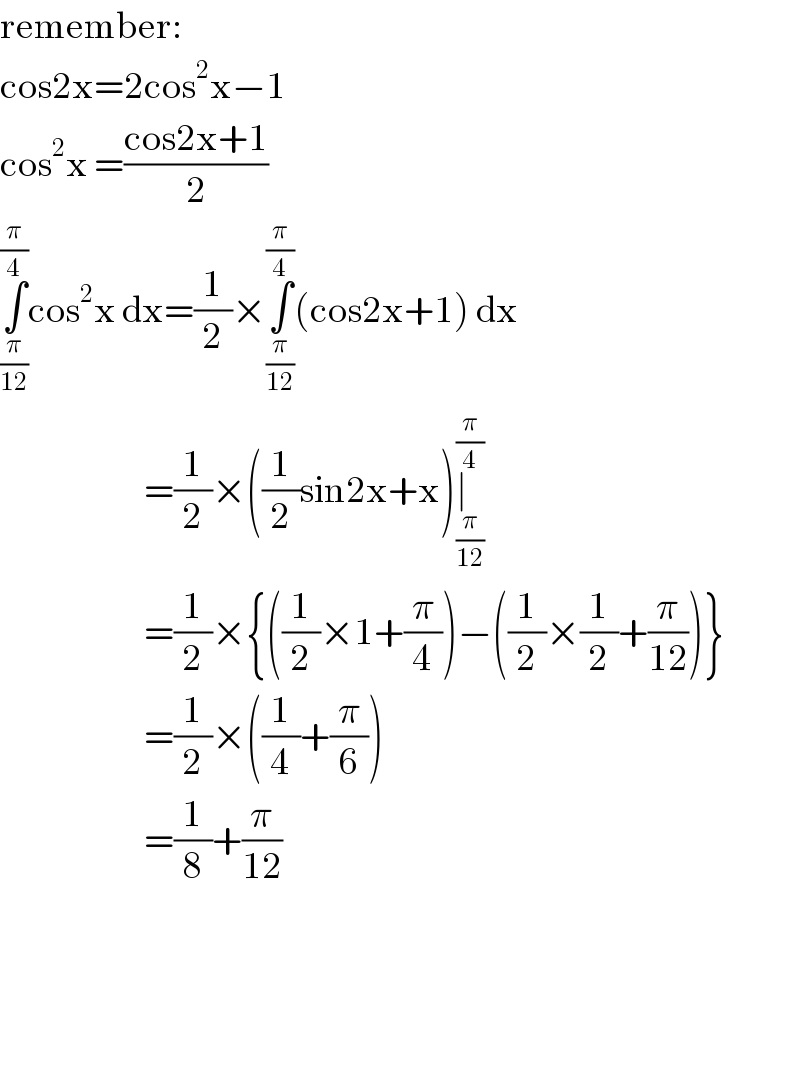
$$\mathrm{remember}:\: \\ $$$$\mathrm{cos2x}=\mathrm{2cos}^{\mathrm{2}} \mathrm{x}−\mathrm{1} \\ $$$$\mathrm{cos}^{\mathrm{2}} \mathrm{x}\:=\frac{\mathrm{cos2x}+\mathrm{1}}{\mathrm{2}} \\ $$$$\underset{\frac{\pi}{\mathrm{12}}} {\overset{\frac{\pi}{\mathrm{4}}} {\int}}\mathrm{cos}^{\mathrm{2}} \mathrm{x}\:\mathrm{dx}=\frac{\mathrm{1}}{\mathrm{2}}×\underset{\frac{\pi}{\mathrm{12}}} {\overset{\frac{\pi}{\mathrm{4}}} {\int}}\left(\mathrm{cos2x}+\mathrm{1}\right)\:\mathrm{dx} \\ $$$$\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:=\frac{\mathrm{1}}{\mathrm{2}}×\left(\frac{\mathrm{1}}{\mathrm{2}}\mathrm{sin2x}+\mathrm{x}\right)\underset{\frac{\pi}{\mathrm{12}}} {\overset{\frac{\pi}{\mathrm{4}}} {\mid}} \\ $$$$\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:=\frac{\mathrm{1}}{\mathrm{2}}×\left\{\left(\frac{\mathrm{1}}{\mathrm{2}}×\mathrm{1}+\frac{\pi}{\mathrm{4}}\right)−\left(\frac{\mathrm{1}}{\mathrm{2}}×\frac{\mathrm{1}}{\mathrm{2}}+\frac{\pi}{\mathrm{12}}\right)\right\} \\ $$$$\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:=\frac{\mathrm{1}}{\mathrm{2}}×\left(\frac{\mathrm{1}}{\mathrm{4}}+\frac{\pi}{\mathrm{6}}\right) \\ $$$$\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:=\frac{\mathrm{1}}{\mathrm{8}}+\frac{\pi}{\mathrm{12}} \\ $$$$ \\ $$$$ \\ $$$$ \\ $$
Commented by tawakalitu last updated on 29/Oct/16

$$\mathrm{Thanks}\:\mathrm{for}\:\mathrm{your}\:\mathrm{help}\:\mathrm{sir}. \\ $$
