Menu Close

Prove-that-m-1-n-1-m-2-m-1-n-C-m-m-m-1-n-1-m-m-

Tinku Tara 0 Comments
Pin




Question Number 4103 by prakash jain last updated on 28/Dec/15
Prove that Σ_(m=1) ^n (((−1)^m ∙(2^m −1) ∙^n C_m )/m) =Σ_(m=1) ^n (((−1)^m )/m)
$$\mathrm{Prove}\:\mathrm{that} \\ $$$$\underset{{m}=\mathrm{1}} {\overset{{n}} {\sum}}\frac{\left(−\mathrm{1}\right)^{{m}} \:\centerdot\left(\mathrm{2}^{{m}} −\mathrm{1}\right)\:\centerdot\:^{{n}} {C}_{{m}} }{{m}}\:\:=\underset{{m}=\mathrm{1}} {\overset{{n}} {\sum}}\frac{\left(−\mathrm{1}\right)^{{m}} }{{m}} \\ $$
Commented by Yozzii last updated on 29/Dec/15
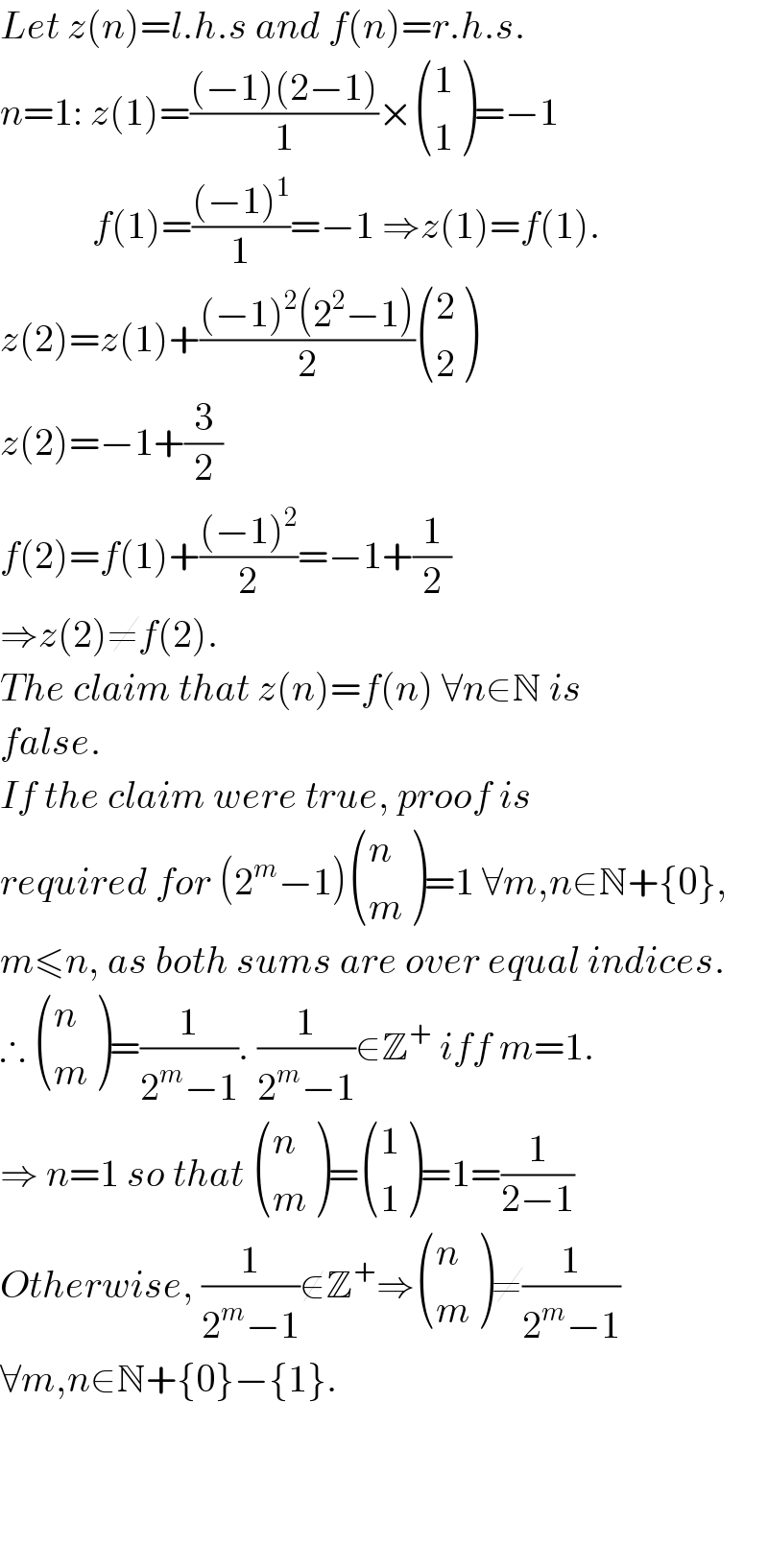
Let z(n)=l.h.s and f(n)=r.h.s. n=1: z(1)=(((−1)(2−1))/1)× ((1),(1) )=−1 f(1)=(((−1)^1 )/1)=−1 ⇒z(1)=f(1). z(2)=z(1)+(((−1)^2 (2^2 −1))/2) ((2),(2) ) z(2)=−1+(3/2) f(2)=f(1)+(((−1)^2 )/2)=−1+(1/2) ⇒z(2)≠f(2). The claim that z(n)=f(n) ∀n∈N is false. If the claim were true, proof is required for (2^m −1) ((n),(m) )=1 ∀m,n∈N+{0}, m≤n, as both sums are over equal indices. ∴ ((n),(m) )=(1/(2^m −1)). (1/(2^m −1))∈Z^+ iff m=1. ⇒ n=1 so that ((n),(m) )= ((1),(1) )=1=(1/(2−1)) Otherwise, (1/(2^m −1))∉Z^+ ⇒ ((n),(m) )≠(1/(2^m −1)) ∀m,n∈N+{0}−{1}.
$${Let}\:{z}\left({n}\right)={l}.{h}.{s}\:{and}\:{f}\left({n}\right)={r}.{h}.{s}. \\ $$$${n}=\mathrm{1}:\:{z}\left(\mathrm{1}\right)=\frac{\left(−\mathrm{1}\right)\left(\mathrm{2}−\mathrm{1}\right)}{\mathrm{1}}×\begin{pmatrix}{\mathrm{1}}\\{\mathrm{1}}\end{pmatrix}=−\mathrm{1} \\ $$$$\:\:\:\:\:\:\:\:\:\:\:\:{f}\left(\mathrm{1}\right)=\frac{\left(−\mathrm{1}\right)^{\mathrm{1}} }{\mathrm{1}}=−\mathrm{1}\:\Rightarrow{z}\left(\mathrm{1}\right)={f}\left(\mathrm{1}\right). \\ $$$${z}\left(\mathrm{2}\right)={z}\left(\mathrm{1}\right)+\frac{\left(−\mathrm{1}\right)^{\mathrm{2}} \left(\mathrm{2}^{\mathrm{2}} −\mathrm{1}\right)}{\mathrm{2}}\begin{pmatrix}{\mathrm{2}}\\{\mathrm{2}}\end{pmatrix} \\ $$$${z}\left(\mathrm{2}\right)=−\mathrm{1}+\frac{\mathrm{3}}{\mathrm{2}} \\ $$$${f}\left(\mathrm{2}\right)={f}\left(\mathrm{1}\right)+\frac{\left(−\mathrm{1}\right)^{\mathrm{2}} }{\mathrm{2}}=−\mathrm{1}+\frac{\mathrm{1}}{\mathrm{2}} \\ $$$$\Rightarrow{z}\left(\mathrm{2}\right)\neq{f}\left(\mathrm{2}\right). \\ $$$${The}\:{claim}\:{that}\:{z}\left({n}\right)={f}\left({n}\right)\:\forall{n}\in\mathbb{N}\:{is} \\ $$$${false}.\: \\ $$$${If}\:{the}\:{claim}\:{were}\:{true},\:{proof}\:{is}\: \\ $$$${required}\:{for}\:\left(\mathrm{2}^{{m}} −\mathrm{1}\right)\begin{pmatrix}{{n}}\\{{m}}\end{pmatrix}=\mathrm{1}\:\forall{m},{n}\in\mathbb{N}+\left\{\mathrm{0}\right\}, \\ $$$${m}\leqslant{n},\:{as}\:{both}\:{sums}\:{are}\:{over}\:{equal}\:{indices}. \\ $$$$\therefore\:\begin{pmatrix}{{n}}\\{{m}}\end{pmatrix}=\frac{\mathrm{1}}{\mathrm{2}^{{m}} −\mathrm{1}}.\:\frac{\mathrm{1}}{\mathrm{2}^{{m}} −\mathrm{1}}\in\mathbb{Z}^{+} \:{iff}\:{m}=\mathrm{1}. \\ $$$$\Rightarrow\:{n}=\mathrm{1}\:{so}\:{that}\:\begin{pmatrix}{{n}}\\{{m}}\end{pmatrix}=\begin{pmatrix}{\mathrm{1}}\\{\mathrm{1}}\end{pmatrix}=\mathrm{1}=\frac{\mathrm{1}}{\mathrm{2}−\mathrm{1}} \\ $$$${Otherwise},\:\frac{\mathrm{1}}{\mathrm{2}^{{m}} −\mathrm{1}}\notin\mathbb{Z}^{+} \Rightarrow\begin{pmatrix}{{n}}\\{{m}}\end{pmatrix}\neq\frac{\mathrm{1}}{\mathrm{2}^{{m}} −\mathrm{1}} \\ $$$$\forall{m},{n}\in\mathbb{N}+\left\{\mathrm{0}\right\}−\left\{\mathrm{1}\right\}. \\ $$$$ \\ $$$$ \\ $$$$ \\ $$
Commented by prakash jain last updated on 29/Dec/15
I should have made these basic checks before asking for proof. Yozzi had shown in earlier question Q2088. ∫_0 ^(π/4) tan^(2n+1) θdθ=(−1)^n ((1/2)ln2+Σ_(m=1) ^n (((−1)^m )/(2m))) In the same time I did a different integral. ∫_0 ^( π/4) tan^(2n) θtan θdθ =∫_0 ^( π/4) (sec^2 θ−1)^n tan θdθ =(−1)^n ∫_0 ^( π/4) (1−sec^2 θ)^n tan θdθ =(−1)^n ∫_0 ^( π/4) [1+Σ_(m=1) ^n (−1)^m ^n C_m sec^(2m) θ]tan θdθ =(−1)^n ∫_0 ^( π/4) tan θdθ+∫_0 ^( π/4) Σ_(m=1) ^n [(−1)^m ^n C_m sec^(2m−1) θ∙sec θtan θ]dθ =(−1)^n [ln sec θ+Σ_(m=1) ^n (((−1)^m ^n C_m sec^(2m) θ)/(2m))]_0 ^(π/4) =(−1)^n (ln (√2)+Σ_(m=1) ^n (((−1)^m ^n C_m (2^m −1))/(2m))) Now if I compare the two results it looks like. Σ_(m=1) ^n (((−1)^m ^n C_m (2^m −1))/(2m))=Σ_(m=1) ^n (((−1)^m )/(2m)) which is not correct. So where is the error?
$$\mathrm{I}\:\mathrm{should}\:\mathrm{have}\:\mathrm{made}\:\mathrm{these}\:\mathrm{basic}\:\mathrm{checks}\:\mathrm{before} \\ $$$$\mathrm{asking}\:\mathrm{for}\:\mathrm{proof}.\:\mathrm{Yozzi}\:\mathrm{had}\:\mathrm{shown}\:\mathrm{in}\:\mathrm{earlier} \\ $$$$\mathrm{question}\:\mathrm{Q2088}. \\ $$$$\int_{\mathrm{0}} ^{\pi/\mathrm{4}} {tan}^{\mathrm{2}{n}+\mathrm{1}} \theta{d}\theta=\left(−\mathrm{1}\right)^{{n}} \left(\frac{\mathrm{1}}{\mathrm{2}}{ln}\mathrm{2}+\underset{{m}=\mathrm{1}} {\overset{{n}} {\sum}}\frac{\left(−\mathrm{1}\right)^{{m}} }{\mathrm{2}{m}}\right) \\ $$$$\mathrm{In}\:\mathrm{the}\:\mathrm{same}\:\mathrm{time}\:\mathrm{I}\:\mathrm{did}\:\mathrm{a}\:\mathrm{different}\:\mathrm{integral}. \\ $$$$\int_{\mathrm{0}} ^{\:\pi/\mathrm{4}} \mathrm{tan}\:^{\mathrm{2}{n}} \theta\mathrm{tan}\:\theta{d}\theta \\ $$$$=\int_{\mathrm{0}} ^{\:\pi/\mathrm{4}} \left(\mathrm{sec}^{\mathrm{2}} \theta−\mathrm{1}\right)^{{n}} \mathrm{tan}\:\theta{d}\theta \\ $$$$=\left(−\mathrm{1}\right)^{{n}} \int_{\mathrm{0}} ^{\:\pi/\mathrm{4}} \left(\mathrm{1}−\mathrm{sec}^{\mathrm{2}} \theta\right)^{{n}} \mathrm{tan}\:\theta{d}\theta \\ $$$$=\left(−\mathrm{1}\right)^{{n}} \int_{\mathrm{0}} ^{\:\pi/\mathrm{4}} \left[\mathrm{1}+\underset{{m}=\mathrm{1}} {\overset{{n}} {\sum}}\left(−\mathrm{1}\right)^{{m}} \:^{{n}} {C}_{{m}} \mathrm{sec}^{\mathrm{2}{m}} \theta\right]\mathrm{tan}\:\theta\mathrm{d}\theta \\ $$$$=\left(−\mathrm{1}\right)^{{n}} \int_{\mathrm{0}} ^{\:\pi/\mathrm{4}} \mathrm{tan}\:\theta\mathrm{d}\theta+\int_{\mathrm{0}} ^{\:\pi/\mathrm{4}} \underset{{m}=\mathrm{1}} {\overset{{n}} {\sum}}\left[\left(−\mathrm{1}\right)^{{m}} \:^{{n}} {C}_{{m}} \mathrm{sec}^{\mathrm{2}{m}−\mathrm{1}} \theta\centerdot\mathrm{sec}\:\theta\mathrm{tan}\:\theta\right]\mathrm{d}\theta \\ $$$$=\left(−\mathrm{1}\right)^{{n}} \left[\mathrm{ln}\:\mathrm{sec}\:\theta+\underset{{m}=\mathrm{1}} {\overset{{n}} {\sum}}\frac{\left(−\mathrm{1}\right)^{{m}} \:^{{n}} {C}_{{m}} \mathrm{sec}\:^{\mathrm{2}{m}} \theta}{\mathrm{2}{m}}\right]_{\mathrm{0}} ^{\pi/\mathrm{4}} \\ $$$$=\left(−\mathrm{1}\right)^{{n}} \left(\mathrm{ln}\:\sqrt{\mathrm{2}}+\underset{{m}=\mathrm{1}} {\overset{{n}} {\sum}}\frac{\left(−\mathrm{1}\right)^{{m}} \:^{{n}} {C}_{{m}} \left(\mathrm{2}^{{m}} −\mathrm{1}\right)}{\mathrm{2}{m}}\right) \\ $$$$\mathrm{Now}\:\mathrm{if}\:\mathrm{I}\:\mathrm{compare}\:\mathrm{the}\:\mathrm{two}\:\mathrm{results}\:\mathrm{it}\:\mathrm{looks}\:\mathrm{like}. \\ $$$$\underset{{m}=\mathrm{1}} {\overset{{n}} {\sum}}\frac{\left(−\mathrm{1}\right)^{{m}} \:^{{n}} {C}_{{m}} \left(\mathrm{2}^{{m}} −\mathrm{1}\right)}{\mathrm{2}{m}}=\underset{{m}=\mathrm{1}} {\overset{{n}} {\sum}}\frac{\left(−\mathrm{1}\right)^{{m}} }{\mathrm{2}{m}} \\ $$$$\mathrm{which}\:\mathrm{is}\:\mathrm{not}\:\mathrm{correct}. \\ $$$$\mathrm{So}\:\mathrm{where}\:\mathrm{is}\:\mathrm{the}\:\mathrm{error}?\: \\ $$
Commented by Yozzii last updated on 29/Dec/15
I recall the question and our discussion on it. I′ll check the working soon.
$${I}\:{recall}\:{the}\:{question}\:{and}\:{our}\: \\ $$$${discussion}\:{on}\:{it}.\:{I}'{ll}\:{check}\:{the}\: \\ $$$${working}\:{soon}. \\ $$
Commented by prakash jain last updated on 29/Dec/15
Thanks. There must be some error in the integral above. I will also recheck.
$$\mathrm{Thanks}.\:\mathrm{There}\:\mathrm{must}\:\mathrm{be}\:\mathrm{some}\:\mathrm{error}\:\mathrm{in}\:\mathrm{the} \\ $$$$\mathrm{integral}\:\mathrm{above}.\:\mathrm{I}\:\mathrm{will}\:\mathrm{also}\:\mathrm{recheck}. \\ $$
Commented by Yozzii last updated on 29/Dec/15
I thought that the identity worked inductively but apparently it does not. In other words, you cannot add the nth term to the partial sum of n−1 terms to give the nth partial sum in z(n). For n=2: z(2)=(((−1)^1 (2^1 −1) ((2),(1) ))/1)+(((−1)^2 (2^2 −1) ((2),(2) ))/2) z(2)=−2+(3/2)=((−1)/2)=f(2)=((−1)/1)+(1/2) If done inductively, as detailed above, z(2)=z(1)+(3/2)=(((−1)^1 (2−1) ((1),(1) ))/1)+(3/2)=(1/2)≠f(2) However, the sum Σ_(m=1) ^n (((−1)^m )/m) works inductively. Sorry for my error in my first comment.
$$ \\ $$$${I}\:{thought}\:{that}\:{the}\:{identity}\:{worked} \\ $$$${inductively}\:{but}\:{apparently}\:{it}\:{does}\:{not}. \\ $$$${In}\:{other}\:{words},\:{you}\:{cannot}\:{add}\:{the}\:{nth} \\ $$$${term}\:{to}\:{the}\:{partial}\:{sum}\:{of}\:{n}−\mathrm{1}\:{terms} \\ $$$${to}\:{give}\:{the}\:{nth}\:{partial}\:{sum}\:{in}\:{z}\left({n}\right). \\ $$$${For}\:{n}=\mathrm{2}: \\ $$$${z}\left(\mathrm{2}\right)=\frac{\left(−\mathrm{1}\right)^{\mathrm{1}} \left(\mathrm{2}^{\mathrm{1}} −\mathrm{1}\right)\begin{pmatrix}{\mathrm{2}}\\{\mathrm{1}}\end{pmatrix}}{\mathrm{1}}+\frac{\left(−\mathrm{1}\right)^{\mathrm{2}} \left(\mathrm{2}^{\mathrm{2}} −\mathrm{1}\right)\begin{pmatrix}{\mathrm{2}}\\{\mathrm{2}}\end{pmatrix}}{\mathrm{2}} \\ $$$${z}\left(\mathrm{2}\right)=−\mathrm{2}+\frac{\mathrm{3}}{\mathrm{2}}=\frac{−\mathrm{1}}{\mathrm{2}}={f}\left(\mathrm{2}\right)=\frac{−\mathrm{1}}{\mathrm{1}}+\frac{\mathrm{1}}{\mathrm{2}} \\ $$$$ \\ $$$${If}\:{done}\:{inductively},\:{as}\:{detailed}\:{above}, \\ $$$${z}\left(\mathrm{2}\right)={z}\left(\mathrm{1}\right)+\frac{\mathrm{3}}{\mathrm{2}}=\frac{\left(−\mathrm{1}\right)^{\mathrm{1}} \left(\mathrm{2}−\mathrm{1}\right)\begin{pmatrix}{\mathrm{1}}\\{\mathrm{1}}\end{pmatrix}}{\mathrm{1}}+\frac{\mathrm{3}}{\mathrm{2}}=\frac{\mathrm{1}}{\mathrm{2}}\neq{f}\left(\mathrm{2}\right) \\ $$$${However},\:{the}\:{sum}\:\underset{{m}=\mathrm{1}} {\overset{{n}} {\sum}}\frac{\left(−\mathrm{1}\right)^{{m}} }{{m}}\:{works} \\ $$$${inductively}. \\ $$$$ \\ $$$${Sorry}\:{for}\:{my}\:{error}\:{in}\:{my}\:{first}\:{comment}. \\ $$$$ \\ $$$$ \\ $$
Commented by prakash jain last updated on 29/Dec/15
Ok. So the assertion in question is not disproved. I also did some computer test for very large n and both LHS and RHS converged to −ln 2. Is there a way to prove the statement in question?
$$\mathrm{Ok}.\:\mathrm{So}\:\mathrm{the}\:\mathrm{assertion}\:\mathrm{in}\:\mathrm{question}\:\mathrm{is}\:\mathrm{not} \\ $$$$\mathrm{disproved}. \\ $$$$\mathrm{I}\:\mathrm{also}\:\mathrm{did}\:\mathrm{some}\:\mathrm{computer}\:\mathrm{test}\:\mathrm{for}\:\mathrm{very}\:\mathrm{large} \\ $$$${n}\:\mathrm{and}\:\mathrm{both}\:\mathrm{LHS}\:\mathrm{and}\:\mathrm{RHS}\:\mathrm{converged}\:\mathrm{to}\:−\mathrm{ln}\:\mathrm{2}. \\ $$$$\mathrm{Is}\:\mathrm{there}\:\mathrm{a}\:\mathrm{way}\:\mathrm{to}\:\mathrm{prove}\:\mathrm{the}\:\mathrm{statement}\:\mathrm{in} \\ $$$$\mathrm{question}? \\ $$
Commented by Yozzii last updated on 29/Dec/15
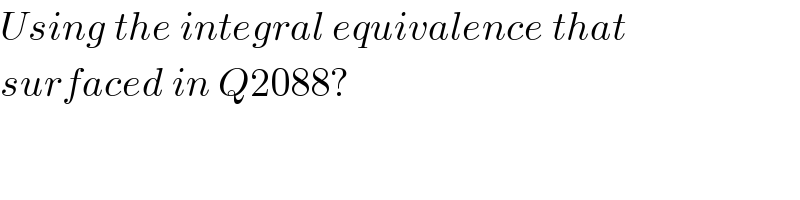
Using the integral equivalence that surfaced in Q2088?
$${Using}\:{the}\:{integral}\:{equivalence}\:{that} \\ $$$${surfaced}\:{in}\:{Q}\mathrm{2088}? \\ $$
Commented by prakash jain last updated on 29/Dec/15
That is an option but without using the integral. Suppose the question is asked as I asked now, it is hard to imagine the integral to prove. I have made a few attempts but could not get a simple proof. May by induction?
$$\mathrm{That}\:\mathrm{is}\:\mathrm{an}\:\mathrm{option}\:\mathrm{but}\:\mathrm{without}\:\mathrm{using}\:\mathrm{the} \\ $$$$\mathrm{integral}.\:\mathrm{Suppose}\:\mathrm{the}\:\mathrm{question}\:\mathrm{is}\:\mathrm{asked}\:\mathrm{as}\:\mathrm{I} \\ $$$$\mathrm{asked}\:\mathrm{now},\:\:\mathrm{it}\:\mathrm{is}\:\mathrm{hard}\:\mathrm{to}\:\mathrm{imagine}\:\mathrm{the}\:\mathrm{integral} \\ $$$$\mathrm{to}\:\mathrm{prove}. \\ $$$$\mathrm{I}\:\mathrm{have}\:\mathrm{made}\:\mathrm{a}\:\mathrm{few}\:\mathrm{attempts}\:\mathrm{but}\:\mathrm{could}\:\mathrm{not} \\ $$$$\mathrm{get}\:\mathrm{a}\:\mathrm{simple}\:\mathrm{proof}.\:\mathrm{May}\:\mathrm{by}\:\mathrm{induction}? \\ $$
Commented by Yozzii last updated on 29/Dec/15
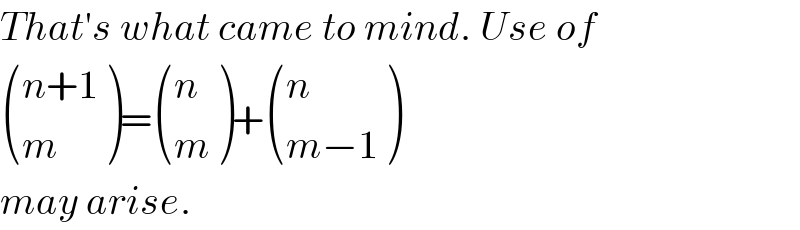
That′s what came to mind. Use of (((n+1)),(m) )= ((n),(m) )+ ((n),((m−1)) ) may arise.
$${That}'{s}\:{what}\:{came}\:{to}\:{mind}.\:{Use}\:{of} \\ $$$$\begin{pmatrix}{{n}+\mathrm{1}}\\{{m}}\end{pmatrix}=\begin{pmatrix}{{n}}\\{{m}}\end{pmatrix}+\begin{pmatrix}{{n}}\\{{m}−\mathrm{1}}\end{pmatrix} \\ $$$${may}\:{arise}.\: \\ $$

Pin

Leave a Reply

Your email address will not be published. Required fields are marked *