Question Number 76657 by Tony Lin last updated on 29/Dec/19
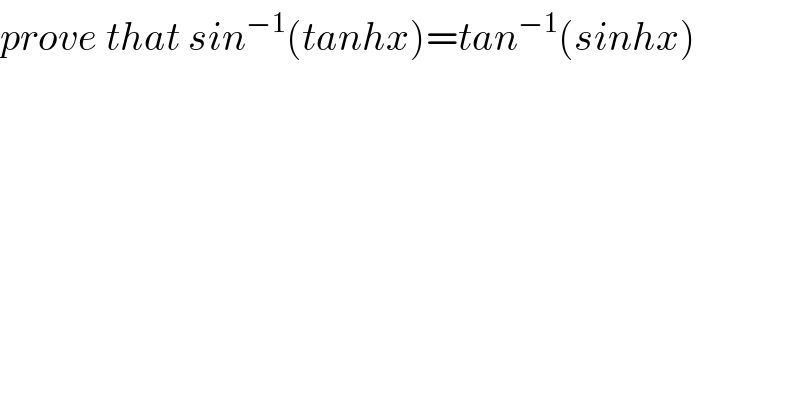
$${prove}\:{that}\:{sin}^{−\mathrm{1}} \left({tanhx}\right)={tan}^{−\mathrm{1}} \left({sinhx}\right) \\ $$
Answered by john santu last updated on 29/Dec/19

$$\left(\mathrm{1}\right)\:\mathrm{tan}\:{hx}\:=\:\mathrm{sin}\:{y} \\ $$$$\mathrm{sin}\:{y}\:=\frac{{e}^{{x}} −{e}^{−{x}} }{{e}^{{x}} +{e}^{−{x}} }\:\rightarrow\:{e}^{{x}} −{e}^{−{x}} =\left({e}^{{x}} +{e}^{−{x}} \right)\mathrm{sin}\:{y} \\ $$$$\left(\mathrm{2}\right)\:\mathrm{tan}^{−\mathrm{1}} \left(\mathrm{sin}\:{hx}\right)=\:{t} \\ $$$$\mathrm{sin}\:{hx}\:=\mathrm{tan}\:{t}\:\rightarrow\:\frac{{e}^{{x}} −{e}^{−{x}} }{\mathrm{2}}=\mathrm{tan}\:{t} \\ $$$${e}^{{x}} −{e}^{−{x}} =\mathrm{2tan}\:{t}\:\rightarrow\:\left({e}^{{x}} +{e}^{−{x}} \right)\mathrm{sin}\:{y}\:=\mathrm{2tan}\:{t} \\ $$$$\frac{{e}^{{x}} +{e}^{−{x}} }{\mathrm{2}}×\mathrm{sin}\:{y}\:=\:\mathrm{tan}\:{t} \\ $$$$\mathrm{cosh}\:{x}×\mathrm{tanh}\:{x}=\mathrm{sinh}\:{x}\: \\ $$$$\mathrm{tanh}\:{x}\:=\:\mathrm{tanh}\:{x}\:. \\ $$
Commented by mr W last updated on 29/Dec/19
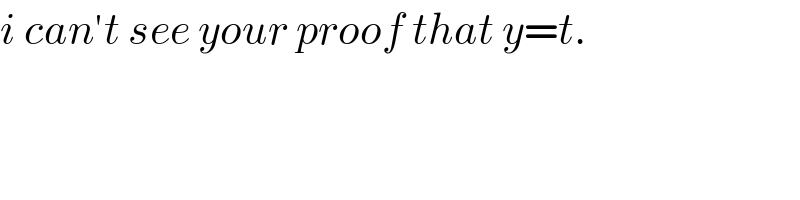
$${i}\:{can}'{t}\:{see}\:{your}\:{proof}\:{that}\:{y}={t}. \\ $$
Commented by john santu last updated on 29/Dec/19
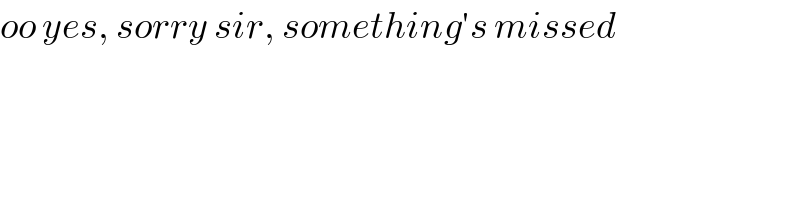
$${oo}\:{yes},\:{sorry}\:{sir},\:{something}'{s}\:{missed} \\ $$
Answered by mr W last updated on 29/Dec/19

$${let}\:{LHS}={sin}^{−\mathrm{1}} \left({tanhx}\right)={u} \\ $$$$\Rightarrow\mathrm{tanh}\:{x}=\mathrm{sin}\:{u} \\ $$$$\Rightarrow\frac{\mathrm{sinh}\:{x}}{\mathrm{cosh}\:{x}}=\mathrm{sin}\:{u} \\ $$$$\Rightarrow\frac{\mathrm{sinh}^{\mathrm{2}} \:{x}}{\mathrm{cosh}^{\mathrm{2}} \:{x}}=\mathrm{sin}^{\mathrm{2}} \:{u} \\ $$$$\Rightarrow\frac{\mathrm{sinh}^{\mathrm{2}} \:{x}}{\mathrm{1}+\mathrm{sinh}^{\mathrm{2}} \:{x}}=\mathrm{sin}^{\mathrm{2}} \:{u} \\ $$$$\Rightarrow\mathrm{sinh}^{\mathrm{2}} \:{x}=\frac{\mathrm{sin}^{\mathrm{2}} \:{u}}{\mathrm{1}−\mathrm{sin}^{\mathrm{2}} \:{u}}=\frac{\mathrm{sin}^{\mathrm{2}} \:{u}}{\mathrm{cos}^{\mathrm{2}} \:{u}}=\mathrm{tan}^{\mathrm{2}} \:{u} \\ $$$$\Rightarrow\mathrm{sinh}\:{x}=\mathrm{tan}\:{u} \\ $$$$\Rightarrow\mathrm{tan}^{−\mathrm{1}} \left(\mathrm{sinh}\:{x}\right)={u} \\ $$$$\Rightarrow\mathrm{tan}^{−\mathrm{1}} \left(\mathrm{sinh}\:{x}\right)={sin}^{−\mathrm{1}} \left({tanhx}\right) \\ $$
Commented by Tony Lin last updated on 29/Dec/19

$${thank}\:{you}\:{both} \\ $$
Answered by mind is power last updated on 29/Dec/19
![Hello happy too back withe you Since th(x) is bijection ]−∞,+∞[−{0}→]−1,1[ ⇒∀u∈]−1,+1[,∃!x∈R^∗ ∣u=th(x) sin^− (th(x))=sin^− (u) tan^− (sh(x))=tan^− (sh(th^− (u))) th(u)=((sh(u))/(ch(u))) ch^2 −sh^2 =1⇒ sh^2 (u)=((th^2 (u))/(1−th^2 (u))) ⇒sh^2 (th^− (u))=(u^2 /(1−u^2 )) sh(th^− (u))=(u/( (√(1−u^2 )))) tan^− ((u/( (√(1−u^2 )))))=?sin^− (u),u∈[−1,1],t→sint bijection [−(π/2),(π/2)]→[−1,1] u=sin(α)⇒(u/( (√(1−u^2 ))))=tan(α) tan^− (tan(α))=α sin^− (sinα)=α,since α is unique since we have bijection ⇒injection ⇒tan^− ((u/( (√(1−u^2 )))))=sin^− (u) end of proof](https://www.tinkutara.com/question/Q76705.png)
$$\mathrm{Hello}\:\mathrm{happy}\:\mathrm{too}\:\mathrm{back}\:\mathrm{withe}\:\mathrm{you}\: \\ $$$$\mathrm{Since} \\ $$$$\left.\mathrm{th}\left(\mathrm{x}\right)\:\mathrm{is}\:\mathrm{bijection}\:\right]−\infty,+\infty\left[−\left\{\mathrm{0}\right\}\rightarrow\right]−\mathrm{1},\mathrm{1}\left[\right. \\ $$$$\left.\Rightarrow\forall\mathrm{u}\in\right]−\mathrm{1},+\mathrm{1}\left[,\exists!\mathrm{x}\in\mathbb{R}^{\ast} \:\:\mid\mathrm{u}=\mathrm{th}\left(\mathrm{x}\right)\right. \\ $$$$\mathrm{sin}^{−} \left(\mathrm{th}\left(\mathrm{x}\right)\right)=\mathrm{sin}^{−} \left(\mathrm{u}\right) \\ $$$$\mathrm{tan}^{−} \left(\mathrm{sh}\left(\mathrm{x}\right)\right)=\mathrm{tan}^{−} \left(\mathrm{sh}\left(\mathrm{th}^{−} \left(\mathrm{u}\right)\right)\right) \\ $$$$\mathrm{th}\left(\mathrm{u}\right)=\frac{\mathrm{sh}\left(\mathrm{u}\right)}{\mathrm{ch}\left(\mathrm{u}\right)} \\ $$$$\mathrm{ch}^{\mathrm{2}} −\mathrm{sh}^{\mathrm{2}} =\mathrm{1}\Rightarrow \\ $$$$\mathrm{sh}^{\mathrm{2}} \left(\mathrm{u}\right)=\frac{\mathrm{th}^{\mathrm{2}} \left(\mathrm{u}\right)}{\mathrm{1}−\mathrm{th}^{\mathrm{2}} \left(\mathrm{u}\right)} \\ $$$$\Rightarrow\mathrm{sh}^{\mathrm{2}} \left(\mathrm{th}^{−} \left(\mathrm{u}\right)\right)=\frac{\mathrm{u}^{\mathrm{2}} }{\mathrm{1}−\mathrm{u}^{\mathrm{2}} } \\ $$$$\mathrm{sh}\left(\mathrm{th}^{−} \left(\mathrm{u}\right)\right)=\frac{\mathrm{u}}{\:\sqrt{\mathrm{1}−\mathrm{u}^{\mathrm{2}} }} \\ $$$$\mathrm{tan}^{−} \left(\frac{\mathrm{u}}{\:\sqrt{\mathrm{1}−\mathrm{u}^{\mathrm{2}} }}\right)=?\mathrm{sin}^{−} \left(\mathrm{u}\right),\mathrm{u}\in\left[−\mathrm{1},\mathrm{1}\right],\mathrm{t}\rightarrow\mathrm{sint}\:\mathrm{bijection}\:\left[−\frac{\pi}{\mathrm{2}},\frac{\pi}{\mathrm{2}}\right]\rightarrow\left[−\mathrm{1},\mathrm{1}\right] \\ $$$$ \\ $$$$\mathrm{u}=\mathrm{sin}\left(\alpha\right)\Rightarrow\frac{\mathrm{u}}{\:\sqrt{\mathrm{1}−\mathrm{u}^{\mathrm{2}} }}=\mathrm{tan}\left(\alpha\right) \\ $$$$\mathrm{tan}^{−} \left(\mathrm{tan}\left(\alpha\right)\right)=\alpha \\ $$$$\mathrm{sin}^{−} \left(\mathrm{sin}\alpha\right)=\alpha,\mathrm{since}\:\alpha\:\mathrm{is}\:\mathrm{unique}\:\mathrm{since}\:\mathrm{we}\:\mathrm{have}\:\mathrm{bijection}\:\Rightarrow\mathrm{injection} \\ $$$$\Rightarrow\mathrm{tan}^{−} \left(\frac{\mathrm{u}}{\:\sqrt{\mathrm{1}−\mathrm{u}^{\mathrm{2}} }}\right)=\mathrm{sin}^{−} \left(\mathrm{u}\right) \\ $$$$\mathrm{end}\:\mathrm{of}\:\mathrm{proof} \\ $$
Commented by Tony Lin last updated on 31/Dec/19

$${thanks}\:{sir} \\ $$
