prove-that-the-equation-b-2-4ac-x-2-4-a-c-x-4-0-is-always-real-

Question Number 69778 by Rio Michael last updated on 27/Sep/19

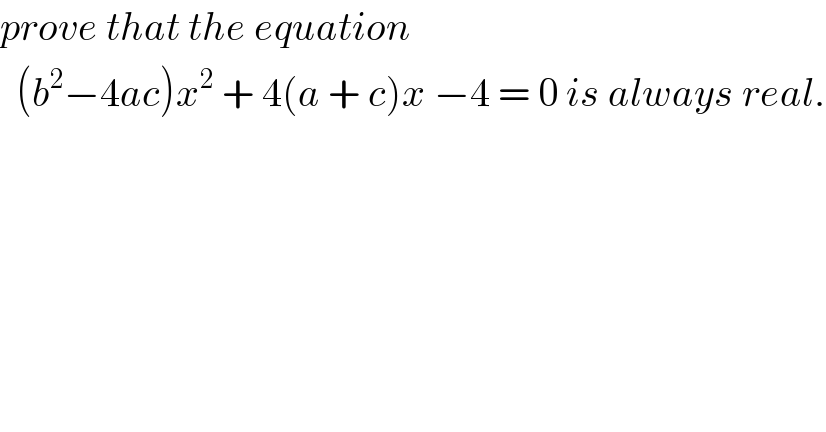
p r o v e t h a t t h e e q u a t i o n

(b^{2} - 4 a c) x^{2} + 4 (a + c) x - 4 = 0 i s a l w a y s r e a l .

Commented by prakash jain last updated on 28/Sep/19

Hi Rasheed

So glad to see that you are still here .

Commented by prakash jain last updated on 27/Sep/19

Δ = {(4 (a + c))}^{2} - 4 \times (- 4) (b^{2} - 4 a c)

= 16 a^{2} + 16 c^{2} + 32 a c + 16 b^{2} - 64 a c

= 16 a^{2} + 16 c^{2} - 32 a c + 16 b^{2}

= 16 {(a - c)}^{2} + 16 b^{2}

{(a - c)}^{2} is always ⩾ 0

b^{2} is always ⩾ 0

hence

Δ ⩾ 0

\Rightarrow roots are always real

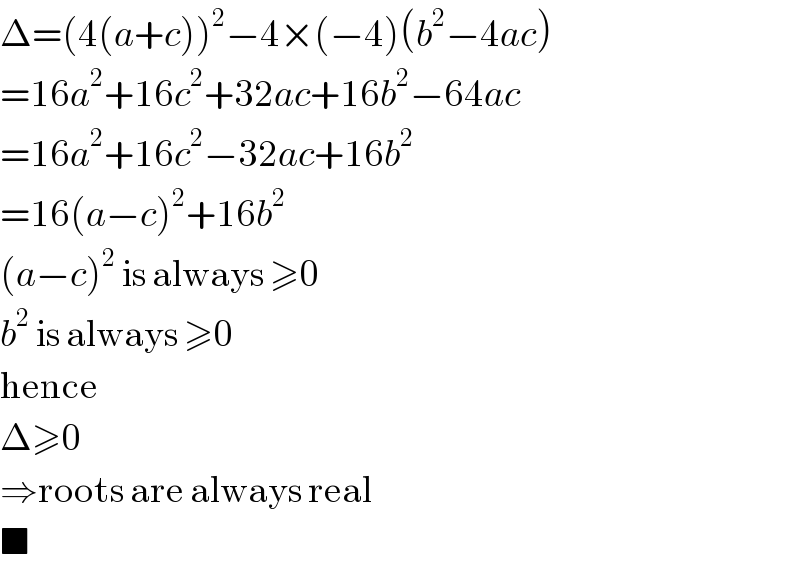
Commented by Abdo msup. last updated on 28/Sep/19

h e l l o p r a k a s h y o u a r e a b s e n t f o r a l o n g t i m e \dots

Commented by Rasheed.Sindhi last updated on 28/Sep/19

W e l c o m e s i r p r a k a s h . H a p p y t o s e e

y o u i n t h e f o r u m! T h e f o r u m n e e d s y o u .

Commented by Rio Michael last updated on 28/Sep/19

t h a n k s s i r

Answered by mind is power last updated on 27/Sep/19

a = b = c = 0 f a l s e

Commented by Rio Michael last updated on 27/Sep/19

w h a t d o y o u m e a n s i r ?

Commented by mind is power last updated on 27/Sep/19

y o u r q u a t i o n m e a n {a x}^{2} + b x + c = 0 . . E l e t y r o o t o f E

\Rightarrow (b^{2} - 4 a c) y^{2} + 4 (a + c) y - 4 = 0 ?

Commented by JDamian last updated on 28/Sep/19

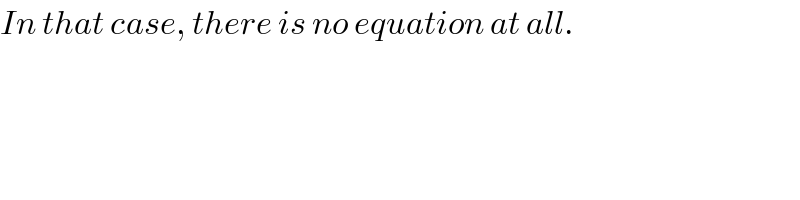
I n t h a t c a s e, t h e r e i s n o e q u a t i o n a t a l l .

Answered by MJS last updated on 27/Sep/19

usually when dealing with

{a x}^{2} + b x + c = 0

it is clearly implied that a \neq 0

\Rightarrow

x^{2} + \frac{4 (a + c)}{b^{2} - 4 a c} x - \frac{4}{b^{2} - 4 a c} = 0; a, b, c \in R \land b^{2} - 4 a c \neq 0

x^{2} + p x + q = 0 \Leftrightarrow x = - \frac{p}{2} \pm \sqrt{\frac{p^{2}}{4} - q}

this is real for D = \frac{p^{2}}{4} - q ⩾ 0

\Rightarrow D = 4 \frac{a^{2} - 2 a c + b^{2} + c^{2}}{{(b^{2} - 4 a c)}^{2}} = 4 \frac{{(a - c)}^{2} + b^{2}}{{(b^{2} - 4 a c)}^{2}} > 0 \Rightarrow

\Rightarrow x \in R

Commented by Rio Michael last updated on 27/Sep/19

t h a n k y o u s i r