Question Number 10062 by ridwan balatif last updated on 22/Jan/17

Commented by ridwan balatif last updated on 22/Jan/17

$$\mathrm{find}\:\mathrm{the}\:\mathrm{remainder}\:\mathrm{of}\:\mathrm{the}\:\mathrm{division}\:\mathrm{20}^{\mathrm{2017}} +\mathrm{1}^{\mathrm{2017}} +\mathrm{17}^{\mathrm{2017}} +\mathrm{72}^{\mathrm{2017}} \:\mathrm{by}\:\mathrm{2017} \\ $$
Answered by nume1114 last updated on 23/Jan/17
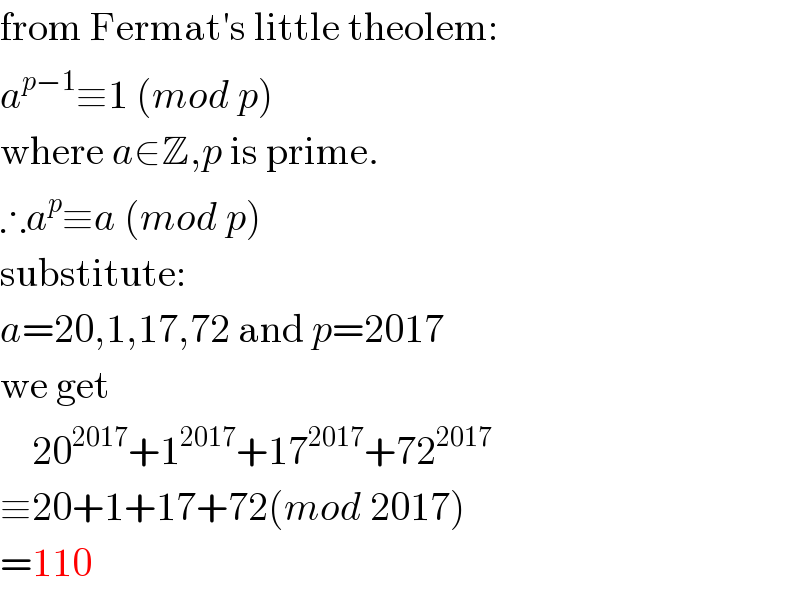
$$\mathrm{from}\:\mathrm{Fermat}'\mathrm{s}\:\mathrm{little}\:\mathrm{theolem}: \\ $$$${a}^{{p}−\mathrm{1}} \equiv\mathrm{1}\:\left({mod}\:{p}\right) \\ $$$$\mathrm{where}\:{a}\in\mathbb{Z},{p}\:\mathrm{is}\:\mathrm{prime}. \\ $$$$\therefore{a}^{{p}} \equiv{a}\:\left({mod}\:{p}\right) \\ $$$$\mathrm{substitute}: \\ $$$${a}=\mathrm{20},\mathrm{1},\mathrm{17},\mathrm{72}\:\mathrm{and}\:{p}=\mathrm{2017} \\ $$$$\mathrm{we}\:\mathrm{get} \\ $$$$\:\:\:\:\mathrm{20}^{\mathrm{2017}} +\mathrm{1}^{\mathrm{2017}} +\mathrm{17}^{\mathrm{2017}} +\mathrm{72}^{\mathrm{2017}} \\ $$$$\equiv\mathrm{20}+\mathrm{1}+\mathrm{17}+\mathrm{72}\left({mod}\:\mathrm{2017}\right) \\ $$$$=\mathrm{110} \\ $$
