Question Number 10451 by ketto last updated on 09/Feb/17

Answered by amir last updated on 10/Feb/17
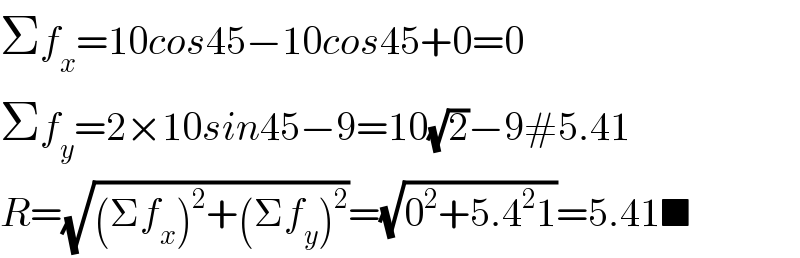
$$\Sigma{f}_{{x}} =\mathrm{10}{cos}\mathrm{45}−\mathrm{10}{cos}\mathrm{45}+\mathrm{0}=\mathrm{0} \\ $$$$\Sigma{f}_{{y}} =\mathrm{2}×\mathrm{10}{sin}\mathrm{45}−\mathrm{9}=\mathrm{10}\sqrt{\mathrm{2}}−\mathrm{9}#\mathrm{5}.\mathrm{41} \\ $$$${R}=\sqrt{\left(\Sigma{f}_{{x}} \right)^{\mathrm{2}} +\left(\Sigma{f}_{{y}} \right)^{\mathrm{2}} }=\sqrt{\mathrm{0}^{\mathrm{2}} +\mathrm{5}.\mathrm{4}^{\mathrm{2}} \mathrm{1}}=\mathrm{5}.\mathrm{41}\blacksquare \\ $$
Answered by ridwan balatif last updated on 10/Feb/17

