Question Number 11695 by b.e.h.i.8.3.4.1.7@gmail.com last updated on 30/Mar/17

Commented by mrW1 last updated on 30/Mar/17
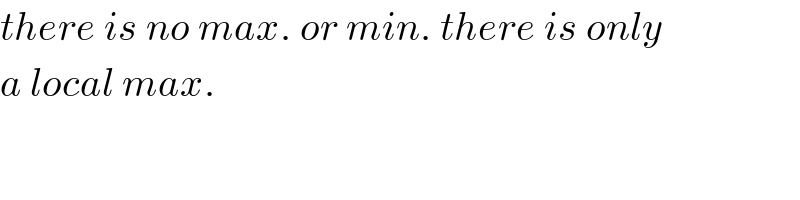
$${there}\:{is}\:{no}\:{max}.\:{or}\:{min}.\:{there}\:{is}\:{only} \\ $$$${a}\:{local}\:{max}. \\ $$
Commented by mrW1 last updated on 30/Mar/17
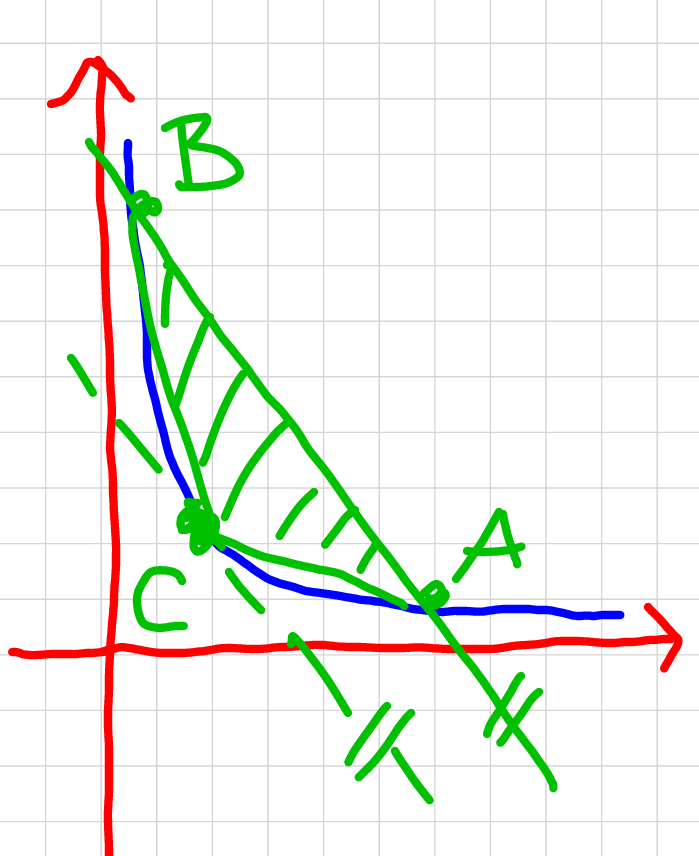
Commented by ajfour last updated on 30/Mar/17
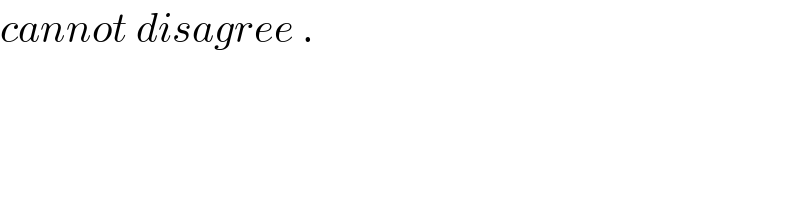
$${cannot}\:{disagree}\:. \\ $$
Commented by linkelly0615 last updated on 30/Mar/17

$${well}….. \\ $$$${hmmm}… \\ $$
Answered by b.e.h.i.8.3.4.1.7@gmail.com last updated on 30/Mar/17
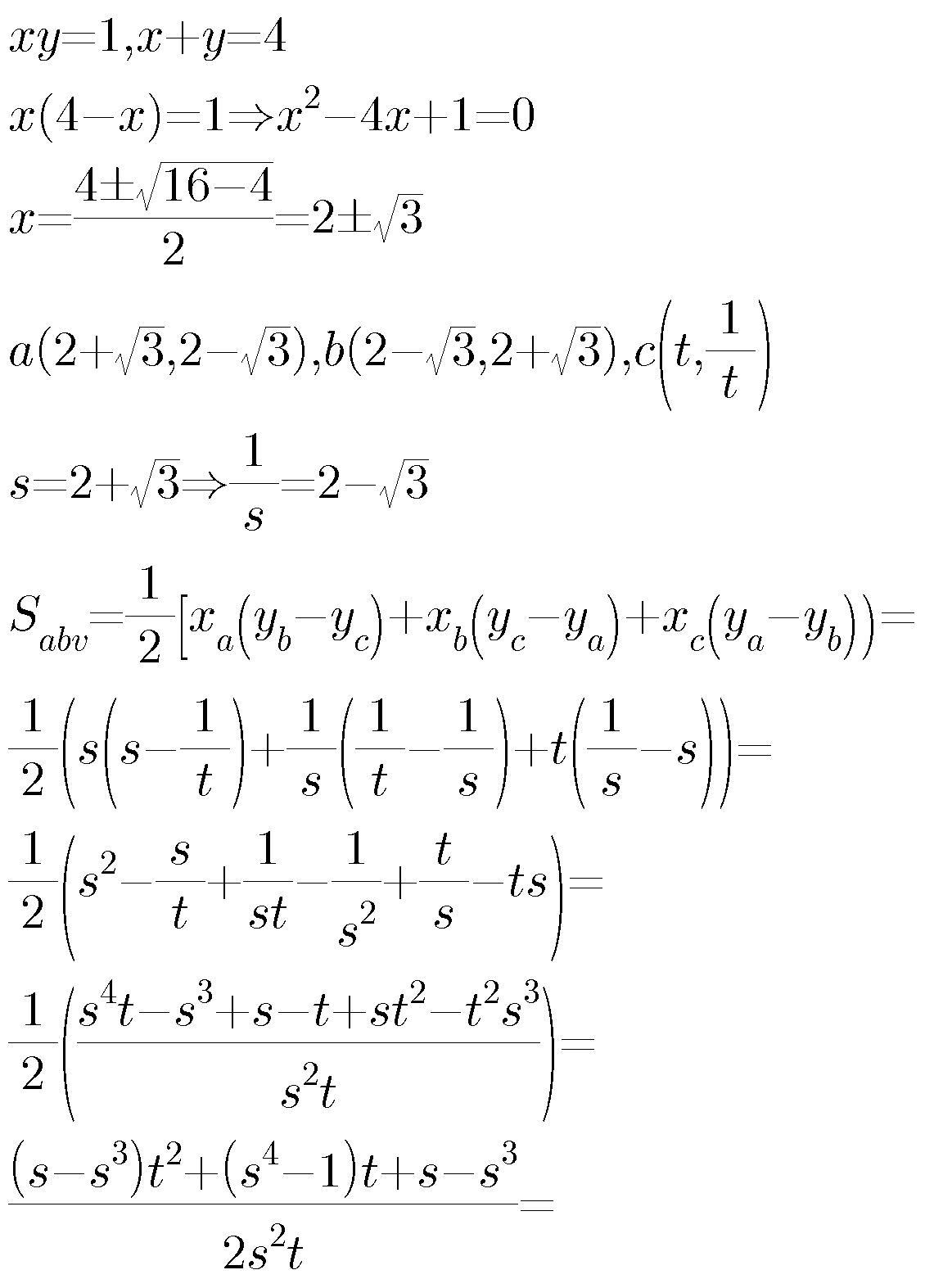
Answered by b.e.h.i.8.3.4.1.7@gmail.com last updated on 30/Mar/17
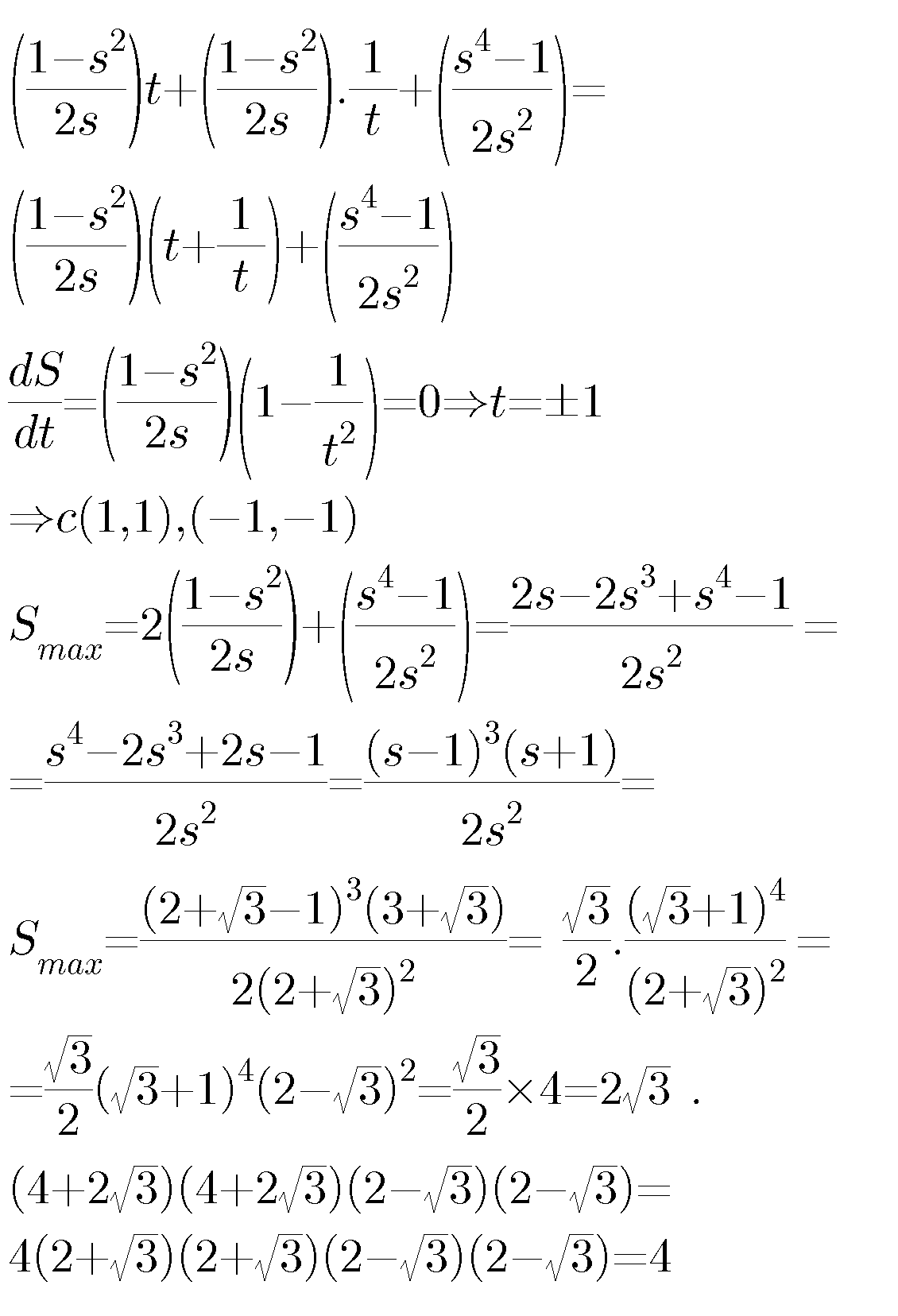
Commented by mrW1 last updated on 30/Mar/17
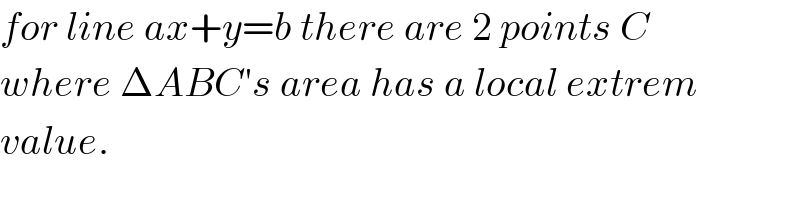
$${for}\:{line}\:{ax}+{y}={b}\:{there}\:{are}\:\mathrm{2}\:{points}\:{C} \\ $$$${where}\:\Delta{ABC}'{s}\:{area}\:{has}\:{a}\:{local}\:{extrem} \\ $$$${value}. \\ $$
