Question Number 11868 by Nayon last updated on 03/Apr/17

Answered by sandy_suhendra last updated on 03/Apr/17

Commented by sandy_suhendra last updated on 03/Apr/17
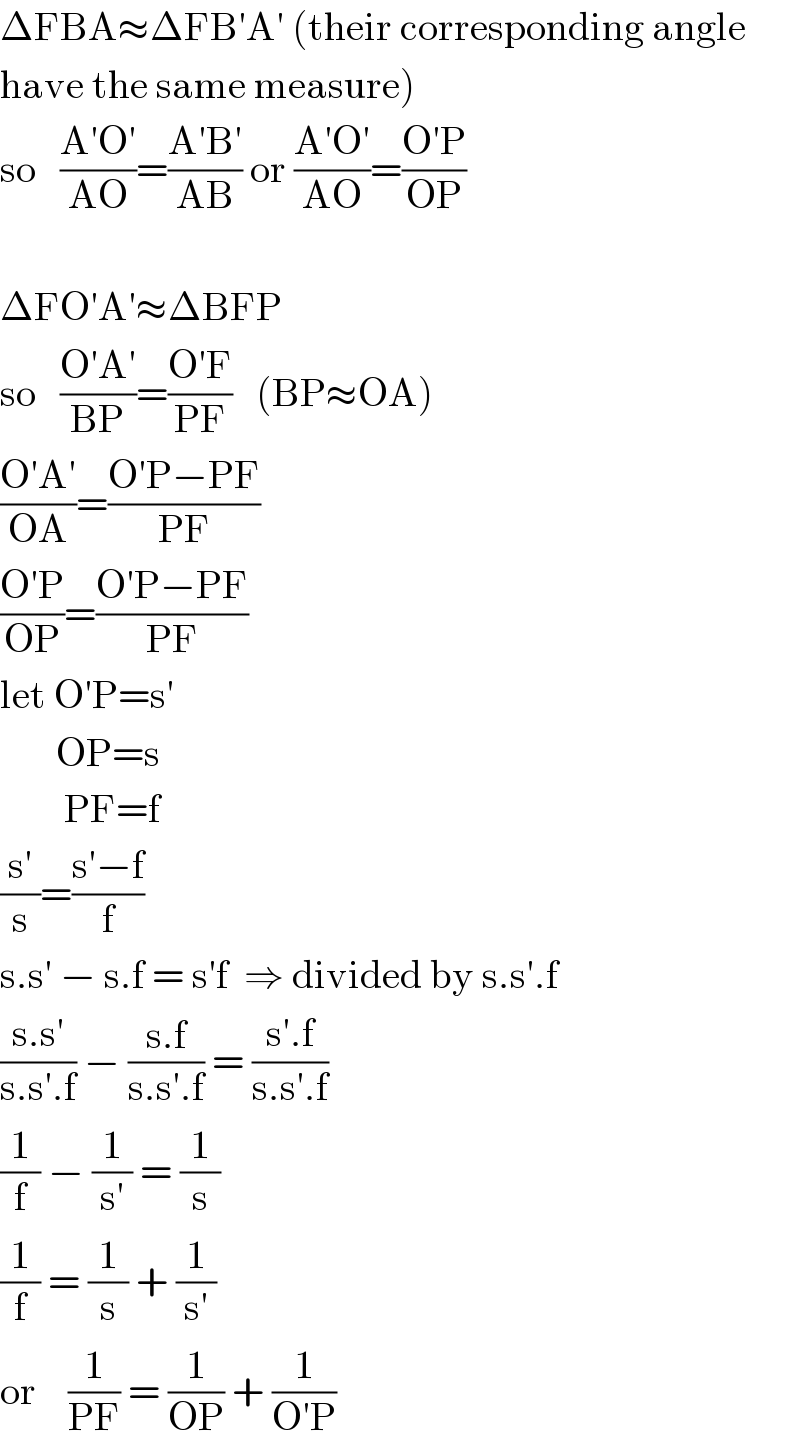
$$\Delta\mathrm{FBA}\approx\Delta\mathrm{FB}'\mathrm{A}'\:\left(\mathrm{their}\:\mathrm{corresponding}\:\mathrm{angle}\:\:\:\:\:\right. \\ $$$$\left.\mathrm{have}\:\mathrm{the}\:\mathrm{same}\:\mathrm{measure}\right) \\ $$$$\mathrm{so}\:\:\:\frac{\mathrm{A}'\mathrm{O}'}{\mathrm{AO}}=\frac{\mathrm{A}'\mathrm{B}'}{\mathrm{AB}}\:\mathrm{or}\:\frac{\mathrm{A}'\mathrm{O}'}{\mathrm{AO}}=\frac{\mathrm{O}'\mathrm{P}}{\mathrm{OP}} \\ $$$$ \\ $$$$\Delta\mathrm{FO}'\mathrm{A}'\approx\Delta\mathrm{BFP} \\ $$$$\mathrm{so}\:\:\:\frac{\mathrm{O}'\mathrm{A}'}{\mathrm{BP}}=\frac{\mathrm{O}'\mathrm{F}}{\mathrm{PF}}\:\:\:\left(\mathrm{BP}\approx\mathrm{OA}\right) \\ $$$$\frac{\mathrm{O}'\mathrm{A}'}{\mathrm{OA}}=\frac{\mathrm{O}'\mathrm{P}−\mathrm{PF}}{\mathrm{PF}} \\ $$$$\frac{\mathrm{O}'\mathrm{P}}{\mathrm{OP}}=\frac{\mathrm{O}'\mathrm{P}−\mathrm{PF}}{\mathrm{PF}} \\ $$$$\mathrm{let}\:\mathrm{O}'\mathrm{P}=\mathrm{s}' \\ $$$$\:\:\:\:\:\:\:\mathrm{OP}=\mathrm{s} \\ $$$$\:\:\:\:\:\:\:\:\mathrm{PF}=\mathrm{f} \\ $$$$\frac{\mathrm{s}'}{\mathrm{s}}=\frac{\mathrm{s}'−\mathrm{f}}{\mathrm{f}} \\ $$$$\mathrm{s}.\mathrm{s}'\:−\:\mathrm{s}.\mathrm{f}\:=\:\mathrm{s}'\mathrm{f}\:\:\Rightarrow\:\mathrm{divided}\:\mathrm{by}\:\mathrm{s}.\mathrm{s}'.\mathrm{f} \\ $$$$\frac{\mathrm{s}.\mathrm{s}'}{\mathrm{s}.\mathrm{s}'.\mathrm{f}}\:−\:\frac{\mathrm{s}.\mathrm{f}}{\mathrm{s}.\mathrm{s}'.\mathrm{f}}\:=\:\frac{\mathrm{s}'.\mathrm{f}}{\mathrm{s}.\mathrm{s}'.\mathrm{f}} \\ $$$$\frac{\mathrm{1}}{\mathrm{f}}\:−\:\frac{\mathrm{1}}{\mathrm{s}'}\:=\:\frac{\mathrm{1}}{\mathrm{s}} \\ $$$$\frac{\mathrm{1}}{\mathrm{f}}\:=\:\frac{\mathrm{1}}{\mathrm{s}}\:+\:\frac{\mathrm{1}}{\mathrm{s}'} \\ $$$$\mathrm{or}\:\:\:\:\frac{\mathrm{1}}{\mathrm{PF}}\:=\:\frac{\mathrm{1}}{\mathrm{OP}}\:+\:\frac{\mathrm{1}}{\mathrm{O}'\mathrm{P}} \\ $$
Commented by Nayon last updated on 06/Apr/17

$${hy}\:{dood}\:{AB}\neq{OP}\: \\ $$
