Question Number 12126 by tawa last updated on 13/Apr/17

Answered by b.e.h.i.8.3.4.1.7@gmail.com last updated on 16/Apr/17
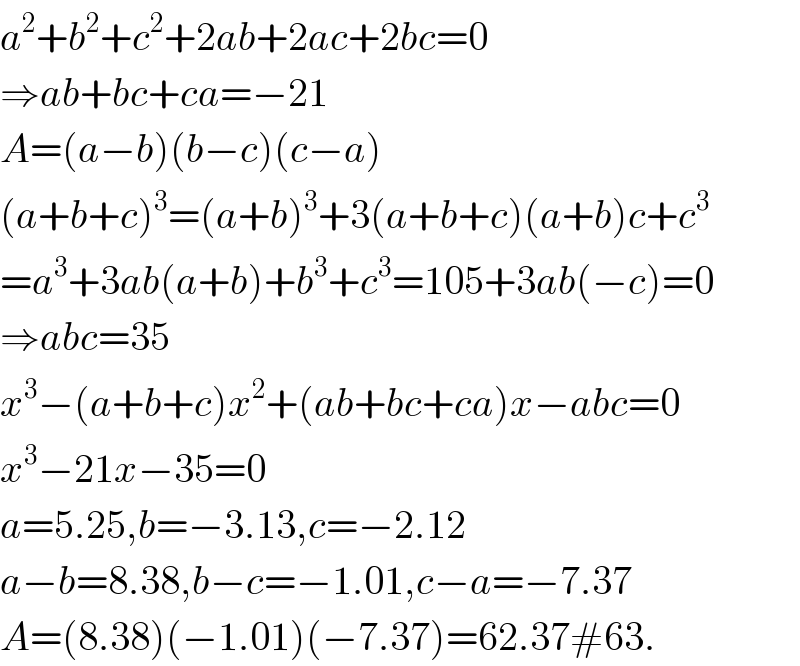
$${a}^{\mathrm{2}} +{b}^{\mathrm{2}} +{c}^{\mathrm{2}} +\mathrm{2}{ab}+\mathrm{2}{ac}+\mathrm{2}{bc}=\mathrm{0} \\ $$$$\Rightarrow{ab}+{bc}+{ca}=−\mathrm{21} \\ $$$${A}=\left({a}−{b}\right)\left({b}−{c}\right)\left({c}−{a}\right) \\ $$$$\left({a}+{b}+{c}\right)^{\mathrm{3}} =\left({a}+{b}\right)^{\mathrm{3}} +\mathrm{3}\left({a}+{b}+{c}\right)\left({a}+{b}\right){c}+{c}^{\mathrm{3}} \\ $$$$={a}^{\mathrm{3}} +\mathrm{3}{ab}\left({a}+{b}\right)+{b}^{\mathrm{3}} +{c}^{\mathrm{3}} =\mathrm{105}+\mathrm{3}{ab}\left(−{c}\right)=\mathrm{0} \\ $$$$\Rightarrow{abc}=\mathrm{35} \\ $$$${x}^{\mathrm{3}} −\left({a}+{b}+{c}\right){x}^{\mathrm{2}} +\left({ab}+{bc}+{ca}\right){x}−{abc}=\mathrm{0} \\ $$$${x}^{\mathrm{3}} −\mathrm{21}{x}−\mathrm{35}=\mathrm{0} \\ $$$${a}=\mathrm{5}.\mathrm{25},{b}=−\mathrm{3}.\mathrm{13},{c}=−\mathrm{2}.\mathrm{12} \\ $$$${a}−{b}=\mathrm{8}.\mathrm{38},{b}−{c}=−\mathrm{1}.\mathrm{01},{c}−{a}=−\mathrm{7}.\mathrm{37} \\ $$$${A}=\left(\mathrm{8}.\mathrm{38}\right)\left(−\mathrm{1}.\mathrm{01}\right)\left(−\mathrm{7}.\mathrm{37}\right)=\mathrm{62}.\mathrm{37}#\mathrm{63}. \\ $$
