Question Number 12148 by b.e.h.i.8.3.4.1.7@gmail.com last updated on 14/Apr/17

Commented by b.e.h.i.8.3.4.1.7@gmail.com last updated on 14/Apr/17
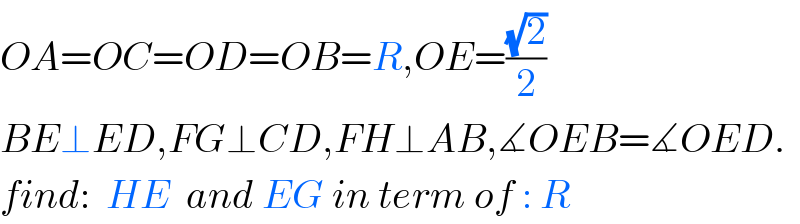
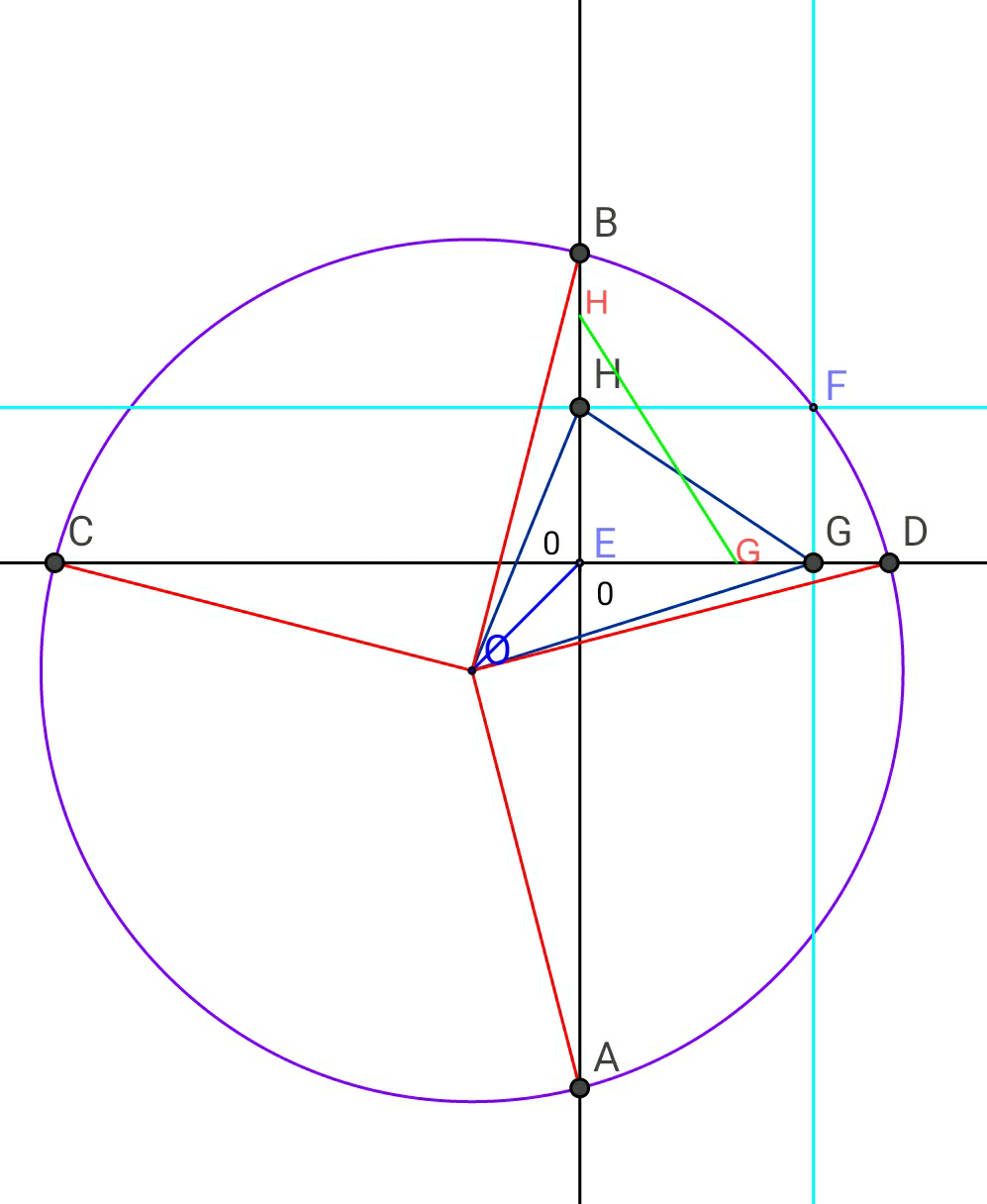
$${OA}={OC}={OD}={OB}={R},{OE}=\frac{\sqrt{\mathrm{2}}}{\mathrm{2}} \\ $$$${BE}\bot{ED},{FG}\bot{CD},{FH}\bot{AB},\measuredangle{OEB}=\measuredangle{OED}. \\ $$$${find}:\:\:{HE}\:\:{and}\:{EG}\:{in}\:{term}\:{of}\::\:{R} \\ $$
Commented by mrW1 last updated on 14/Apr/17

$${no}\:{unique}\:{solution} \\ $$
Commented by b.e.h.i.8.3.4.1.7@gmail.com last updated on 15/Apr/17

$${please}\:{help}\:{me}\:{by}\:{sending}\:{at}\:{last}\:{one} \\ $$$${solution}.{thank}\:{you}\:{so}\:{much}. \\ $$
Commented by mrW1 last updated on 15/Apr/17
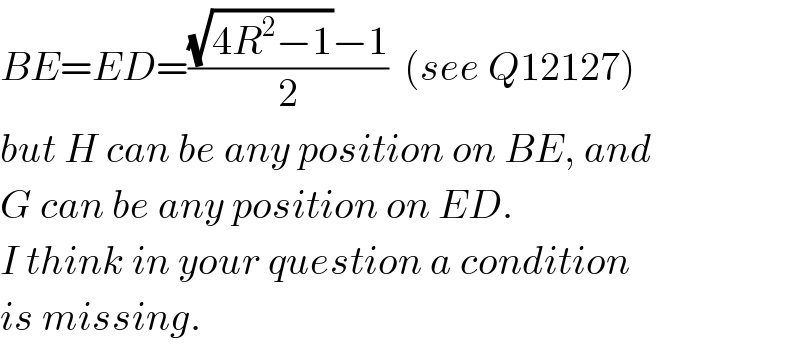
$${BE}={ED}=\frac{\sqrt{\mathrm{4}{R}^{\mathrm{2}} −\mathrm{1}}−\mathrm{1}}{\mathrm{2}}\:\:\left({see}\:{Q}\mathrm{12127}\right) \\ $$$${but}\:{H}\:{can}\:{be}\:{any}\:{position}\:{on}\:{BE},\:{and} \\ $$$${G}\:{can}\:{be}\:{any}\:{position}\:{on}\:{ED}. \\ $$$${I}\:{think}\:{in}\:{your}\:{question}\:{a}\:{condition} \\ $$$${is}\:{missing}. \\ $$
Commented by b.e.h.i.8.3.4.1.7@gmail.com last updated on 15/Apr/17
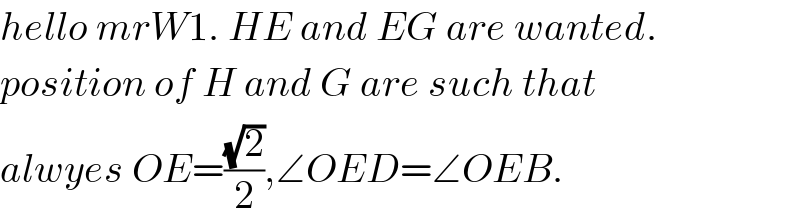
$${hello}\:{mrW}\mathrm{1}.\:{HE}\:{and}\:{EG}\:{are}\:{wanted}. \\ $$$${position}\:{of}\:{H}\:{and}\:{G}\:{are}\:{such}\:{that}\: \\ $$$${alwyes}\:{OE}=\frac{\sqrt{\mathrm{2}}}{\mathrm{2}},\angle{OED}=\angle{OEB}. \\ $$
Commented by mrW1 last updated on 15/Apr/17

$${you}\:{can}\:{only}\:{determine}\:{EB}\:{and}\:{ED}, \\ $$$${but}\:{not}\:{EH}\:{and}\:{EG}.\:{to}\:{determine}\: \\ $$$${EH}\:{and}\:{EG}\:{you}\:{need}\:{an}\:{additional} \\ $$$${condition},\:{e}.{g}.\:{F},\:{E}\:{and}\:{O}\:{are}\:{collinear}. \\ $$
Commented by b.e.h.i.8.3.4.1.7@gmail.com last updated on 15/Apr/17

$${F}\:{alweys}\:{is}\:{on}\:{circle}\:{O}.{O}\left(−\mathrm{0}.\mathrm{5},−\mathrm{0}.\mathrm{5}\right). \\ $$$${equation}\:{of}\:{circle}\:{can}\:{help}\:{us}. \\ $$$${if}\:{F},{E},{O}\:{be}\:{collinear},{we}\:{have}\:{special} \\ $$$${case}\:{that}\:{gives}\:{us}:{EB}={ED}. \\ $$$$ \\ $$
Commented by mrW1 last updated on 15/Apr/17
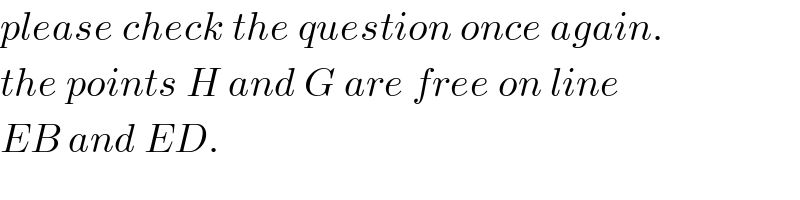
$${please}\:{check}\:{the}\:{question}\:{once}\:{again}. \\ $$$${the}\:{points}\:{H}\:{and}\:{G}\:{are}\:{free}\:{on}\:{line} \\ $$$${EB}\:{and}\:{ED}. \\ $$
Commented by mrW1 last updated on 15/Apr/17
Commented by mrW1 last updated on 15/Apr/17
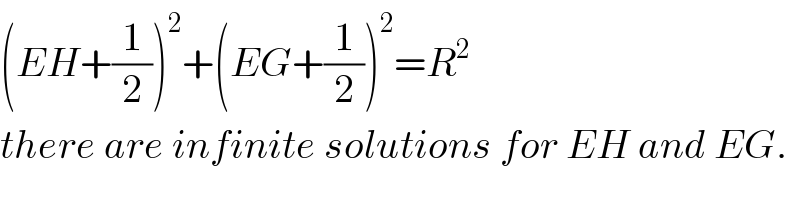
$$\left({EH}+\frac{\mathrm{1}}{\mathrm{2}}\right)^{\mathrm{2}} +\left({EG}+\frac{\mathrm{1}}{\mathrm{2}}\right)^{\mathrm{2}} ={R}^{\mathrm{2}} \\ $$$${there}\:{are}\:{infinite}\:{solutions}\:{for}\:{EH}\:{and}\:{EG}. \\ $$
Commented by chux last updated on 15/Apr/17

$$\mathrm{please}\:\mathrm{which}\:\mathrm{app}\:\mathrm{do}\:\mathrm{you}\:\mathrm{use}\:\mathrm{in}\: \\ $$$$\mathrm{drawing}\:\mathrm{this}?\:\mathrm{n}\:\mathrm{how}\:\mathrm{is}\:\mathrm{it}\:\mathrm{used}?. \\ $$$$\mathrm{thanks} \\ $$
Commented by b.e.h.i.8.3.4.1.7@gmail.com last updated on 15/Apr/17
$${hello}.\:{Geogebra}\:{app}. \\ $$
Commented by chux last updated on 15/Apr/17

$$\mathrm{thanks}\:\mathrm{boss} \\ $$
