Question Number 12682 by b.e.h.i.8.3.4.1.7@gmail.com last updated on 28/Apr/17

Answered by mrW1 last updated on 29/Apr/17
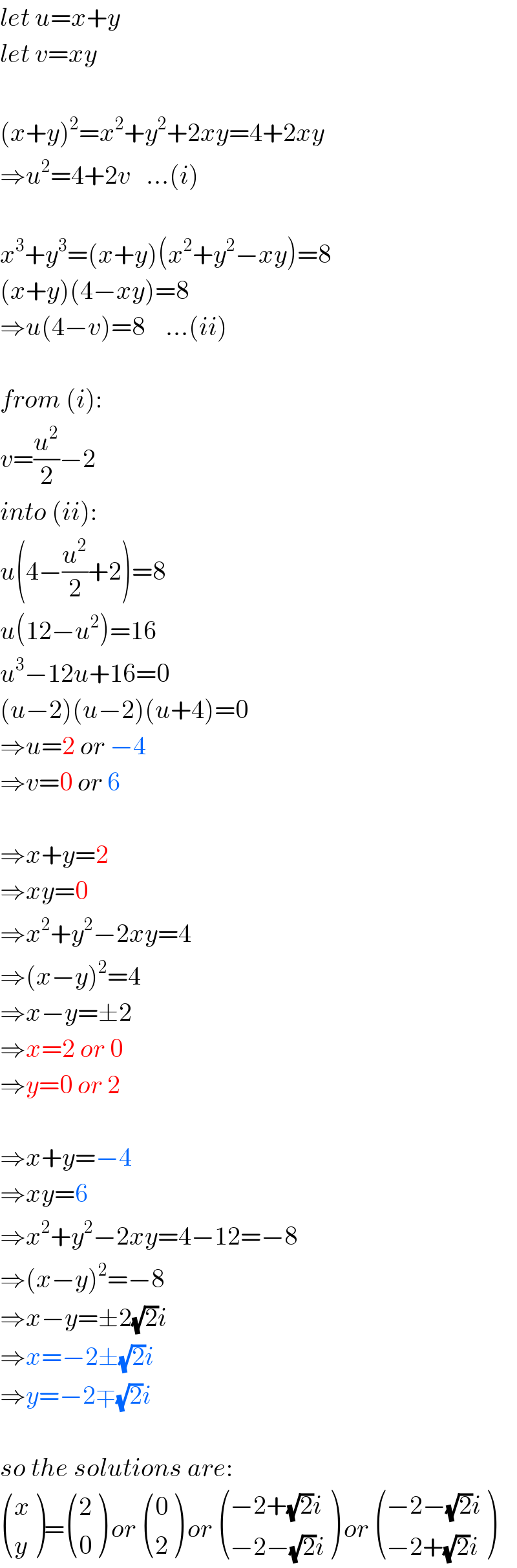
$${let}\:{u}={x}+{y} \\ $$$${let}\:{v}={xy} \\ $$$$ \\ $$$$\left({x}+{y}\right)^{\mathrm{2}} ={x}^{\mathrm{2}} +{y}^{\mathrm{2}} +\mathrm{2}{xy}=\mathrm{4}+\mathrm{2}{xy} \\ $$$$\Rightarrow{u}^{\mathrm{2}} =\mathrm{4}+\mathrm{2}{v}\:\:\:…\left({i}\right) \\ $$$$ \\ $$$${x}^{\mathrm{3}} +{y}^{\mathrm{3}} =\left({x}+{y}\right)\left({x}^{\mathrm{2}} +{y}^{\mathrm{2}} −{xy}\right)=\mathrm{8} \\ $$$$\left({x}+{y}\right)\left(\mathrm{4}−{xy}\right)=\mathrm{8} \\ $$$$\Rightarrow{u}\left(\mathrm{4}−{v}\right)=\mathrm{8}\:\:\:\:…\left({ii}\right) \\ $$$$ \\ $$$${from}\:\left({i}\right): \\ $$$${v}=\frac{{u}^{\mathrm{2}} }{\mathrm{2}}−\mathrm{2} \\ $$$${into}\:\left({ii}\right): \\ $$$${u}\left(\mathrm{4}−\frac{{u}^{\mathrm{2}} }{\mathrm{2}}+\mathrm{2}\right)=\mathrm{8} \\ $$$${u}\left(\mathrm{12}−{u}^{\mathrm{2}} \right)=\mathrm{16} \\ $$$${u}^{\mathrm{3}} −\mathrm{12}{u}+\mathrm{16}=\mathrm{0} \\ $$$$\left({u}−\mathrm{2}\right)\left({u}−\mathrm{2}\right)\left({u}+\mathrm{4}\right)=\mathrm{0} \\ $$$$\Rightarrow{u}=\mathrm{2}\:{or}\:−\mathrm{4} \\ $$$$\Rightarrow{v}=\mathrm{0}\:{or}\:\mathrm{6} \\ $$$$ \\ $$$$\Rightarrow{x}+{y}=\mathrm{2} \\ $$$$\Rightarrow{xy}=\mathrm{0} \\ $$$$\Rightarrow{x}^{\mathrm{2}} +{y}^{\mathrm{2}} −\mathrm{2}{xy}=\mathrm{4} \\ $$$$\Rightarrow\left({x}−{y}\right)^{\mathrm{2}} =\mathrm{4} \\ $$$$\Rightarrow{x}−{y}=\pm\mathrm{2} \\ $$$$\Rightarrow{x}=\mathrm{2}\:{or}\:\mathrm{0} \\ $$$$\Rightarrow{y}=\mathrm{0}\:{or}\:\mathrm{2} \\ $$$$ \\ $$$$\Rightarrow{x}+{y}=−\mathrm{4} \\ $$$$\Rightarrow{xy}=\mathrm{6} \\ $$$$\Rightarrow{x}^{\mathrm{2}} +{y}^{\mathrm{2}} −\mathrm{2}{xy}=\mathrm{4}−\mathrm{12}=−\mathrm{8} \\ $$$$\Rightarrow\left({x}−{y}\right)^{\mathrm{2}} =−\mathrm{8} \\ $$$$\Rightarrow{x}−{y}=\pm\mathrm{2}\sqrt{\mathrm{2}}{i} \\ $$$$\Rightarrow{x}=−\mathrm{2}\pm\sqrt{\mathrm{2}}{i} \\ $$$$\Rightarrow{y}=−\mathrm{2}\mp\sqrt{\mathrm{2}}{i} \\ $$$$ \\ $$$${so}\:{the}\:{solutions}\:{are}: \\ $$$$\begin{pmatrix}{{x}}\\{{y}}\end{pmatrix}=\begin{pmatrix}{\mathrm{2}}\\{\mathrm{0}}\end{pmatrix}\:{or}\:\begin{pmatrix}{\mathrm{0}}\\{\mathrm{2}}\end{pmatrix}\:{or}\:\begin{pmatrix}{−\mathrm{2}+\sqrt{\mathrm{2}}{i}}\\{−\mathrm{2}−\sqrt{\mathrm{2}}{i}}\end{pmatrix}\:{or}\:\begin{pmatrix}{−\mathrm{2}−\sqrt{\mathrm{2}}{i}}\\{−\mathrm{2}+\sqrt{\mathrm{2}}{i}}\end{pmatrix} \\ $$
Commented by b.e.h.i.8.3.4.1.7@gmail.com last updated on 29/Apr/17
$${thanks}\:{a}\:{lot}\:{dear}\:{mrW}\mathrm{1}.{you}\:{are}\:{no}.\mathrm{1}. \\ $$
