Question Number 12962 by tawa last updated on 07/May/17

Answered by sandy_suhendra last updated on 09/May/17

Commented by sandy_suhendra last updated on 09/May/17
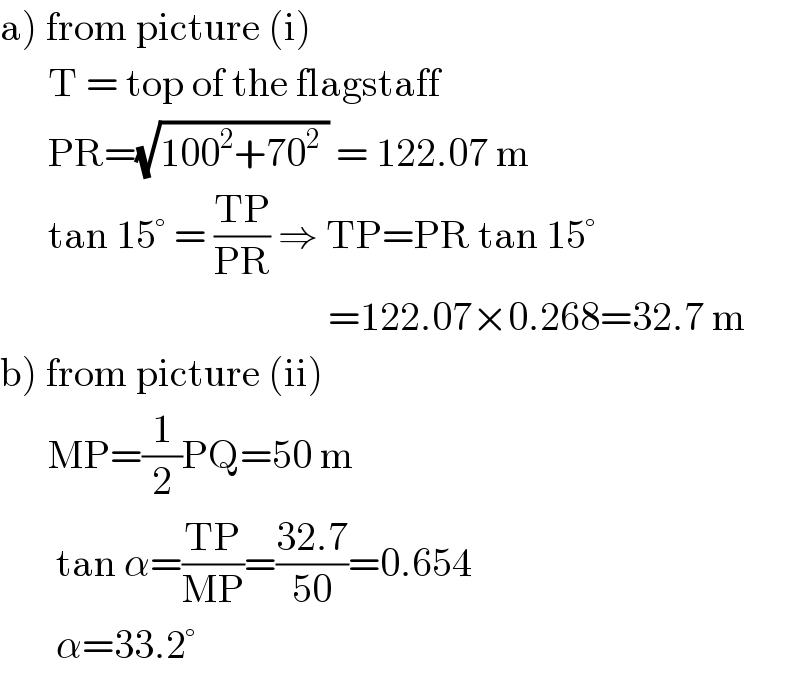
$$\left.\mathrm{a}\right)\:\mathrm{from}\:\mathrm{picture}\:\left(\mathrm{i}\right) \\ $$$$\:\:\:\:\:\:\mathrm{T}\:=\:\mathrm{top}\:\mathrm{of}\:\mathrm{the}\:\mathrm{flagstaff} \\ $$$$\:\:\:\:\:\:\mathrm{PR}=\sqrt{\mathrm{100}^{\mathrm{2}} +\mathrm{70}^{\mathrm{2}} \:}\:=\:\mathrm{122}.\mathrm{07}\:\mathrm{m} \\ $$$$\:\:\:\:\:\:\mathrm{tan}\:\mathrm{15}°\:=\:\frac{\mathrm{TP}}{\mathrm{PR}}\:\Rightarrow\:\mathrm{TP}=\mathrm{PR}\:\mathrm{tan}\:\mathrm{15}° \\ $$$$\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:=\mathrm{122}.\mathrm{07}×\mathrm{0}.\mathrm{268}=\mathrm{32}.\mathrm{7}\:\mathrm{m}\:\:\:\:\: \\ $$$$\left.\mathrm{b}\right)\:\mathrm{from}\:\mathrm{picture}\:\left(\mathrm{ii}\right) \\ $$$$\:\:\:\:\:\:\mathrm{MP}=\frac{\mathrm{1}}{\mathrm{2}}\mathrm{PQ}=\mathrm{50}\:\mathrm{m} \\ $$$$\:\:\:\:\:\:\:\mathrm{tan}\:\alpha=\frac{\mathrm{TP}}{\mathrm{MP}}=\frac{\mathrm{32}.\mathrm{7}}{\mathrm{50}}=\mathrm{0}.\mathrm{654} \\ $$$$\:\:\:\:\:\:\:\alpha=\mathrm{33}.\mathrm{2}° \\ $$
Commented by tawa tawa last updated on 10/May/17
$$\mathrm{wow},\:\mathrm{G}\boldsymbol{{od}}\:\boldsymbol{{bless}}\:\boldsymbol{{you}}\:\boldsymbol{{sir}}. \\ $$
