Question Number 131183 by john_santu last updated on 02/Feb/21
Answered by liberty last updated on 02/Feb/21
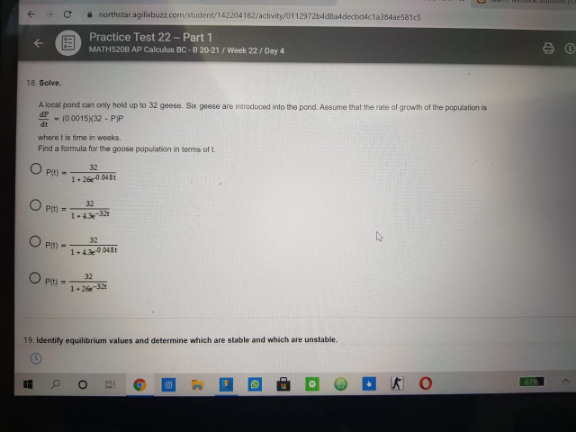
![(dP/((32−P)P)) = 0.0015 dt (1/(32)) ∫ [ (1/(32−P)) +(1/P) ]dP = ∫0.0015 dt (1/(32)) ln ∣(P/(32−P)) ∣ = 0.0015t + c ln ∣(P/(32−P))∣ = 0.48t+C ; ((P(t))/(32−P(t))) = λe^(0.048t) ⇔ ((32−P(t))/(P(t))) = (1/λ)e^(−0.048t) ⇒ ((32)/(P(t)))−1 =(e^(−0.048t) /λ) ; ((32)/(P(t))) = ((λ+e^(−0.048t) )/λ) ⇔P(t)= ((32λ)/(λ+e^(−0.048t) )) = ((32)/(1+(1/λ)e^(−0.048t) )) where P(0) = ((32)/(1+(1/λ))) = 6 ; 1+(1/λ)= ((16)/3) (1/λ) = ((13)/3)= 4.3^− , Therefore P(t)= ((32)/(1+4.3^− e^(−0.048t) ))](https://www.tinkutara.com/question/Q131184.png)
$$\:\frac{\mathrm{dP}}{\left(\mathrm{32}−\mathrm{P}\right)\mathrm{P}}\:=\:\mathrm{0}.\mathrm{0015}\:\mathrm{dt}\: \\ $$$$\frac{\mathrm{1}}{\mathrm{32}}\:\int\:\left[\:\frac{\mathrm{1}}{\mathrm{32}−\mathrm{P}}\:+\frac{\mathrm{1}}{\mathrm{P}}\:\right]\mathrm{dP}\:=\:\int\mathrm{0}.\mathrm{0015}\:\mathrm{dt} \\ $$$$\frac{\mathrm{1}}{\mathrm{32}}\:\mathrm{ln}\:\mid\frac{\mathrm{P}}{\mathrm{32}−\mathrm{P}}\:\mid\:=\:\mathrm{0}.\mathrm{0015t}\:+\:\mathrm{c}\: \\ $$$$\mathrm{ln}\:\mid\frac{\mathrm{P}}{\mathrm{32}−\mathrm{P}}\mid\:=\:\mathrm{0}.\mathrm{48t}+\mathrm{C}\:;\:\frac{\mathrm{P}\left(\mathrm{t}\right)}{\mathrm{32}−\mathrm{P}\left(\mathrm{t}\right)}\:=\:\lambda\mathrm{e}^{\mathrm{0}.\mathrm{048t}} \\ $$$$\Leftrightarrow\:\frac{\mathrm{32}−\mathrm{P}\left(\mathrm{t}\right)}{\mathrm{P}\left(\mathrm{t}\right)}\:=\:\frac{\mathrm{1}}{\lambda}\mathrm{e}^{−\mathrm{0}.\mathrm{048t}} \\ $$$$\Rightarrow\:\frac{\mathrm{32}}{\mathrm{P}\left(\mathrm{t}\right)}−\mathrm{1}\:=\frac{\mathrm{e}^{−\mathrm{0}.\mathrm{048t}} }{\lambda}\:;\:\frac{\mathrm{32}}{\mathrm{P}\left(\mathrm{t}\right)}\:=\:\frac{\lambda+\mathrm{e}^{−\mathrm{0}.\mathrm{048t}} }{\lambda} \\ $$$$\Leftrightarrow\mathrm{P}\left(\mathrm{t}\right)=\:\frac{\mathrm{32}\lambda}{\lambda+\mathrm{e}^{−\mathrm{0}.\mathrm{048t}} }\:=\:\frac{\mathrm{32}}{\mathrm{1}+\frac{\mathrm{1}}{\lambda}\mathrm{e}^{−\mathrm{0}.\mathrm{048t}} } \\ $$$$\mathrm{where}\:\mathrm{P}\left(\mathrm{0}\right)\:=\:\frac{\mathrm{32}}{\mathrm{1}+\frac{\mathrm{1}}{\lambda}}\:=\:\mathrm{6}\:;\:\mathrm{1}+\frac{\mathrm{1}}{\lambda}=\:\frac{\mathrm{16}}{\mathrm{3}}\: \\ $$$$\:\frac{\mathrm{1}}{\lambda}\:=\:\frac{\mathrm{13}}{\mathrm{3}}=\:\mathrm{4}.\overset{−} {\mathrm{3}}\:,\:\mathrm{Therefore}\:\mathrm{P}\left(\mathrm{t}\right)=\:\frac{\mathrm{32}}{\mathrm{1}+\mathrm{4}.\overset{−} {\mathrm{3}}\:\mathrm{e}^{−\mathrm{0}.\mathrm{048t}} } \\ $$$$ \\ $$
