Question Number 131320 by mohammad17 last updated on 03/Feb/21

Answered by mr W last updated on 04/Feb/21
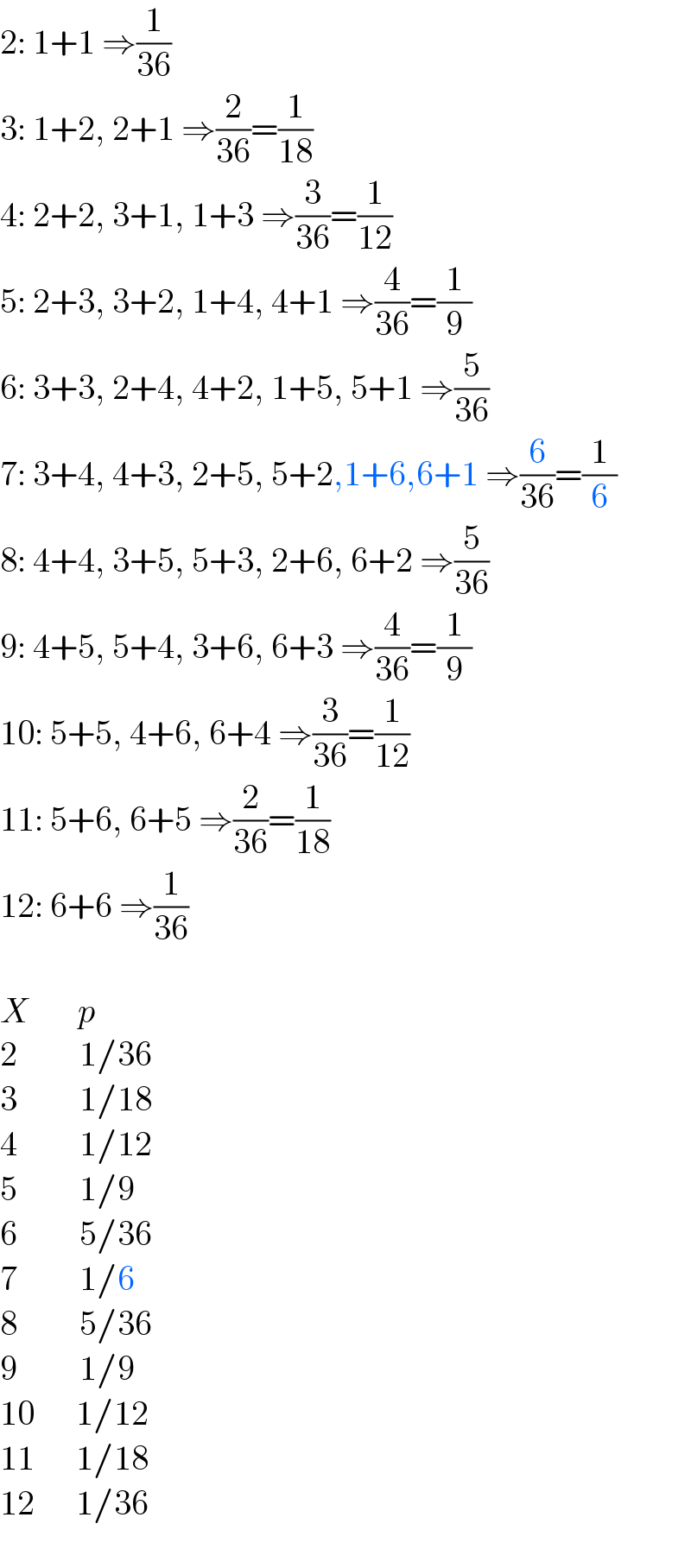
$$\mathrm{2}:\:\mathrm{1}+\mathrm{1}\:\Rightarrow\frac{\mathrm{1}}{\mathrm{36}} \\ $$$$\mathrm{3}:\:\mathrm{1}+\mathrm{2},\:\mathrm{2}+\mathrm{1}\:\Rightarrow\frac{\mathrm{2}}{\mathrm{36}}=\frac{\mathrm{1}}{\mathrm{18}} \\ $$$$\mathrm{4}:\:\mathrm{2}+\mathrm{2},\:\mathrm{3}+\mathrm{1},\:\mathrm{1}+\mathrm{3}\:\Rightarrow\frac{\mathrm{3}}{\mathrm{36}}=\frac{\mathrm{1}}{\mathrm{12}} \\ $$$$\mathrm{5}:\:\mathrm{2}+\mathrm{3},\:\mathrm{3}+\mathrm{2},\:\mathrm{1}+\mathrm{4},\:\mathrm{4}+\mathrm{1}\:\Rightarrow\frac{\mathrm{4}}{\mathrm{36}}=\frac{\mathrm{1}}{\mathrm{9}} \\ $$$$\mathrm{6}:\:\mathrm{3}+\mathrm{3},\:\mathrm{2}+\mathrm{4},\:\mathrm{4}+\mathrm{2},\:\mathrm{1}+\mathrm{5},\:\mathrm{5}+\mathrm{1}\:\Rightarrow\frac{\mathrm{5}}{\mathrm{36}} \\ $$$$\mathrm{7}:\:\mathrm{3}+\mathrm{4},\:\mathrm{4}+\mathrm{3},\:\mathrm{2}+\mathrm{5},\:\mathrm{5}+\mathrm{2},\mathrm{1}+\mathrm{6},\mathrm{6}+\mathrm{1}\:\Rightarrow\frac{\mathrm{6}}{\mathrm{36}}=\frac{\mathrm{1}}{\mathrm{6}} \\ $$$$\mathrm{8}:\:\mathrm{4}+\mathrm{4},\:\mathrm{3}+\mathrm{5},\:\mathrm{5}+\mathrm{3},\:\mathrm{2}+\mathrm{6},\:\mathrm{6}+\mathrm{2}\:\Rightarrow\frac{\mathrm{5}}{\mathrm{36}} \\ $$$$\mathrm{9}:\:\mathrm{4}+\mathrm{5},\:\mathrm{5}+\mathrm{4},\:\mathrm{3}+\mathrm{6},\:\mathrm{6}+\mathrm{3}\:\Rightarrow\frac{\mathrm{4}}{\mathrm{36}}=\frac{\mathrm{1}}{\mathrm{9}} \\ $$$$\mathrm{10}:\:\mathrm{5}+\mathrm{5},\:\mathrm{4}+\mathrm{6},\:\mathrm{6}+\mathrm{4}\:\Rightarrow\frac{\mathrm{3}}{\mathrm{36}}=\frac{\mathrm{1}}{\mathrm{12}} \\ $$$$\mathrm{11}:\:\mathrm{5}+\mathrm{6},\:\mathrm{6}+\mathrm{5}\:\Rightarrow\frac{\mathrm{2}}{\mathrm{36}}=\frac{\mathrm{1}}{\mathrm{18}} \\ $$$$\mathrm{12}:\:\mathrm{6}+\mathrm{6}\:\Rightarrow\frac{\mathrm{1}}{\mathrm{36}} \\ $$$$ \\ $$$${X}\:\:\:\:\:\:\:{p} \\ $$$$\mathrm{2}\:\:\:\:\:\:\:\:\:\mathrm{1}/\mathrm{36} \\ $$$$\mathrm{3}\:\:\:\:\:\:\:\:\:\mathrm{1}/\mathrm{18} \\ $$$$\mathrm{4}\:\:\:\:\:\:\:\:\:\mathrm{1}/\mathrm{12} \\ $$$$\mathrm{5}\:\:\:\:\:\:\:\:\:\mathrm{1}/\mathrm{9} \\ $$$$\mathrm{6}\:\:\:\:\:\:\:\:\:\mathrm{5}/\mathrm{36} \\ $$$$\mathrm{7}\:\:\:\:\:\:\:\:\:\mathrm{1}/\mathrm{6} \\ $$$$\mathrm{8}\:\:\:\:\:\:\:\:\:\mathrm{5}/\mathrm{36} \\ $$$$\mathrm{9}\:\:\:\:\:\:\:\:\:\mathrm{1}/\mathrm{9} \\ $$$$\mathrm{10}\:\:\:\:\:\:\mathrm{1}/\mathrm{12} \\ $$$$\mathrm{11}\:\:\:\:\:\:\mathrm{1}/\mathrm{18} \\ $$$$\mathrm{12}\:\:\:\:\:\:\mathrm{1}/\mathrm{36} \\ $$
Commented by liberty last updated on 11/Feb/21
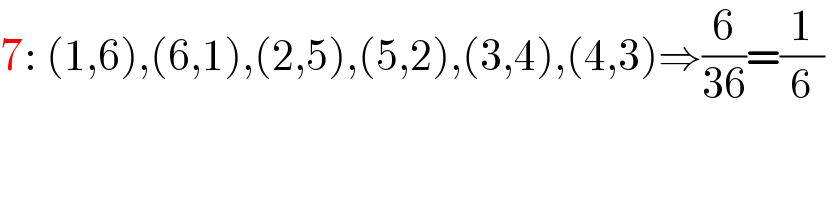
$$\mathrm{7}:\:\left(\mathrm{1},\mathrm{6}\right),\left(\mathrm{6},\mathrm{1}\right),\left(\mathrm{2},\mathrm{5}\right),\left(\mathrm{5},\mathrm{2}\right),\left(\mathrm{3},\mathrm{4}\right),\left(\mathrm{4},\mathrm{3}\right)\Rightarrow\frac{\mathrm{6}}{\mathrm{36}}=\frac{\mathrm{1}}{\mathrm{6}}\: \\ $$
Commented by mr W last updated on 04/Feb/21

$${yes},\:{thanks}! \\ $$
Answered by liberty last updated on 03/Feb/21
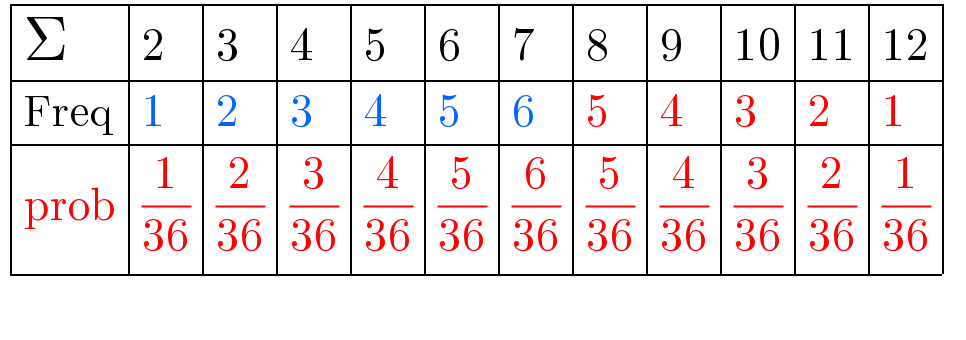
$$\begin{array}{|c|c|c|}{\Sigma}&\hline{\mathrm{2}}&\hline{\mathrm{3}}&\hline{\mathrm{4}}&\hline{\mathrm{5}}&\hline{\mathrm{6}}&\hline{\mathrm{7}}&\hline{\mathrm{8}}&\hline{\mathrm{9}}&\hline{\mathrm{10}}&\hline{\mathrm{11}}&\hline{\mathrm{12}}\\{\mathrm{Freq}}&\hline{\mathrm{1}}&\hline{\mathrm{2}}&\hline{\mathrm{3}}&\hline{\mathrm{4}}&\hline{\mathrm{5}}&\hline{\mathrm{6}}&\hline{\mathrm{5}}&\hline{\mathrm{4}}&\hline{\mathrm{3}}&\hline{\mathrm{2}}&\hline{\mathrm{1}}\\{\mathrm{prob}}&\hline{\frac{\mathrm{1}}{\mathrm{36}}}&\hline{\frac{\mathrm{2}}{\mathrm{36}}}&\hline{\frac{\mathrm{3}}{\mathrm{36}}}&\hline{\frac{\mathrm{4}}{\mathrm{36}}}&\hline{\frac{\mathrm{5}}{\mathrm{36}}}&\hline{\frac{\mathrm{6}}{\mathrm{36}}}&\hline{\frac{\mathrm{5}}{\mathrm{36}}}&\hline{\frac{\mathrm{4}}{\mathrm{36}}}&\hline{\frac{\mathrm{3}}{\mathrm{36}}}&\hline{\frac{\mathrm{2}}{\mathrm{36}}}&\hline{\frac{\mathrm{1}}{\mathrm{36}}}\\\hline\end{array} \\ $$$$ \\ $$
