Question Number 131624 by mr W last updated on 06/Feb/21

Commented by mr W last updated on 06/Feb/21
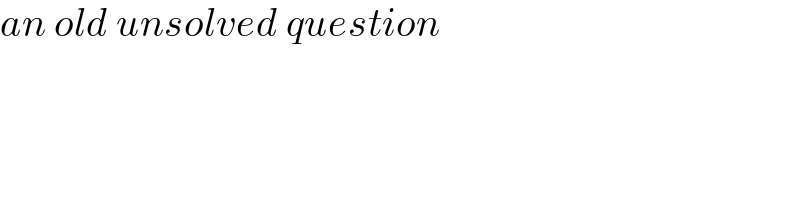
$${an}\:{old}\:{unsolved}\:{question} \\ $$
Commented by MJS_new last updated on 07/Feb/21

$$\mathrm{I}'\mathrm{ll}\:\mathrm{try}… \\ $$
Commented by MJS_new last updated on 08/Feb/21

$$\mathrm{I}\:\mathrm{get}\:\mathrm{blue}\:\mathrm{area}=\mathrm{4}{A} \\ $$$$\alpha\:\mathrm{is}\:\mathrm{the}\:\mathrm{rotation}\:\mathrm{angle}\:\mathrm{of}\:\mathrm{the}\:\mathrm{red}\:\mathrm{square} \\ $$$$\Rightarrow \\ $$$$\mathrm{area}\:\mathrm{of}\:\mathrm{the}\:\mathrm{left}\:\mathrm{square}\:\mathrm{is}\:\left(\mathrm{1}+\mathrm{8sin}^{\mathrm{2}} \:\alpha\right){A} \\ $$$$\mathrm{area}\:\mathrm{of}\:\mathrm{the}\:\mathrm{right}\:\mathrm{square}\:\mathrm{is}\:\left(\mathrm{1}+\mathrm{8cos}^{\mathrm{2}} \:\alpha\right){A} \\ $$
Commented by mr W last updated on 08/Feb/21

$${thanks}\:{sir}! \\ $$
