Question Number 131644 by Ahmed1hamouda last updated on 07/Feb/21

Commented by Ahmed1hamouda last updated on 07/Feb/21

$$ \\ $$$$\mathrm{solve}\:\mathrm{the}\:\mathrm{differential}\:\mathrm{equation} \\ $$
Answered by rs4089 last updated on 07/Feb/21
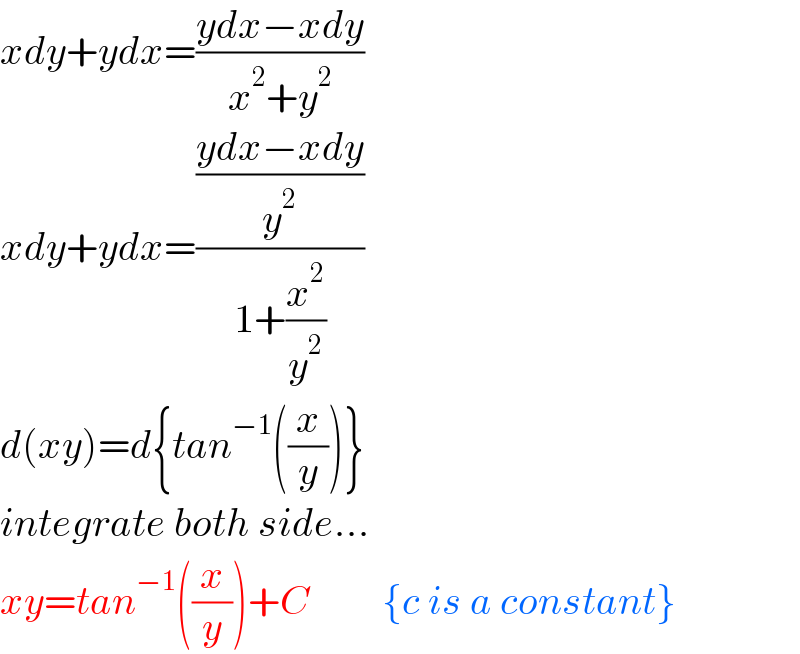
$${xdy}+{ydx}=\frac{{ydx}−{xdy}}{{x}^{\mathrm{2}} +{y}^{\mathrm{2}} } \\ $$$${xdy}+{ydx}=\frac{\frac{{ydx}−{xdy}}{{y}^{\mathrm{2}} }}{\mathrm{1}+\frac{{x}^{\mathrm{2}} }{{y}^{\mathrm{2}} }} \\ $$$${d}\left({xy}\right)={d}\left\{{tan}^{−\mathrm{1}} \left(\frac{{x}}{{y}}\right)\right\} \\ $$$${integrate}\:{both}\:{side}… \\ $$$${xy}={tan}^{−\mathrm{1}} \left(\frac{{x}}{{y}}\right)+{C}\:\:\:\:\:\:\:\:\:\left\{{c}\:{is}\:{a}\:{constant}\right\} \\ $$
