Question Number 133056 by mr W last updated on 19/Feb/21

Commented by mr W last updated on 18/Feb/21

Commented by liberty last updated on 18/Feb/21

Commented by liberty last updated on 18/Feb/21
Commented by mr W last updated on 18/Feb/21

Commented by liberty last updated on 18/Feb/21
Commented by liberty last updated on 18/Feb/21

Answered by mr W last updated on 19/Feb/21

Commented by EDWIN88 last updated on 20/Feb/21

Commented by mr W last updated on 20/Feb/21
![B(L+s cos α, s sin α) with s=ξL, ξ≥0 from A to B: t=((L+s cos α)/(u cos θ))=((L(1+ξ cos α))/(u cos θ)) s sin α=((L(1+ξ cos α))/(u cos θ))[u sin θ−(g/2)×((L(1+ξ cos α))/(u cos θ))] ξ sin α=(1+ξ cos α)[tan θ−((gL(1+ξ cos α)(1+tan^2 θ))/(2u^2 ))] let λ=((gL)/(2u^2 )) ξ sin α=(1+ξ cos α)[tan θ−λ(1+ξ cos α)(1+tan^2 θ)] λ(1+ξ cos α)tan^2 θ−tan θ+((ξ sin α+λ(1+ξ cos α)^2 )/((1+ξ cos α)))=0 ⇒tan θ=((1±(√(1−4λ[ξ sin α+λ(1+ξ cos α)^2 ])))/(2λ(1+ξ cos α))) at point B: (see Q132102 for more detail) U=coming speed V=leaving speed (→) U_x =u cos θ U_y =−u sin θ+g×((L(1+ξ cos α))/(u cos θ)) (↓) U_y =u[−sin θ+((2λ(1+ξ cos α))/(cos θ))] let e′=1+e V_x =(e′ sin^2 α−1)U_x +e′ sin α cos α U_y (←) V_x =u[−e′ sin α sin (θ−α)−cos θ+((λe′(1+ξ cos α) sin 2α)/(cos θ))] V_y =e′ sin α cos α U_x +(e′ cos^2 α−1)U_y (↑) V_y =u[−e′ cos α sin (θ−α)+sin θ+((2λ(e′ cos^2 α−1)(1+ξ cos α))/(cos θ))] from B to A: t=((L(1+ξ cos α))/(u[−e′ sin α sin (θ−α)−cos θ+((λe′(1+ξ cos α) sin 2α)/(cos θ))])) ((tu)/L)=η=((1+ξ cos α)/(−e′ sin α sin (θ−α)−cos θ+((λe′(1+ξ cos α) sin 2α)/(cos θ)))) s sin α=t{−u[−e′ cos α sin (θ−α)+sin θ+((2λ(e′ cos^2 α−1)(1+ξ cos α))/(cos θ))]+(1/2)gt} ξ sin α=((tu)/L)[e′ cos α sin (θ−α)−sin θ−((2λ(e′ cos^2 α−1)(1+ξ cos α))/(cos θ))+((gL)/(2u^2 ))×((tu)/L)] ⇒ξ sin α=η[e′ cos α sin (θ−α)−sin θ−((2λ(e′ cos^2 α−1)(1+ξ cos α))/(cos θ))+λη] ...(I) for a given λ we can find ξ through (I).](https://www.tinkutara.com/question/Q133132.png)
Commented by mr W last updated on 20/Feb/21

Commented by mr W last updated on 20/Feb/21

Commented by mr W last updated on 19/Feb/21

Commented by mr W last updated on 19/Feb/21

Commented by mr W last updated on 19/Feb/21

Commented by mr W last updated on 19/Feb/21
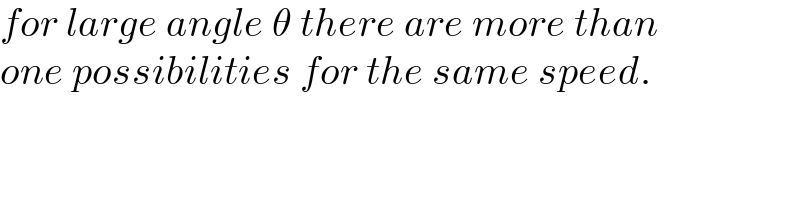
Commented by mr W last updated on 20/Feb/21

Commented by mr W last updated on 20/Feb/21

Commented by mr W last updated on 20/Feb/21

Commented by mr W last updated on 19/Feb/21

Commented by mr W last updated on 20/Feb/21

Commented by mr W last updated on 20/Feb/21

Commented by otchereabdullai@gmail.com last updated on 23/Feb/21

