Question-134678

Question Number 134678 by mr W last updated on 07/Mar/21

Commented by mr W last updated on 06/Mar/21

a t t e m p t t o s o l v e Q 134376

Commented by mr W last updated on 06/Mar/21

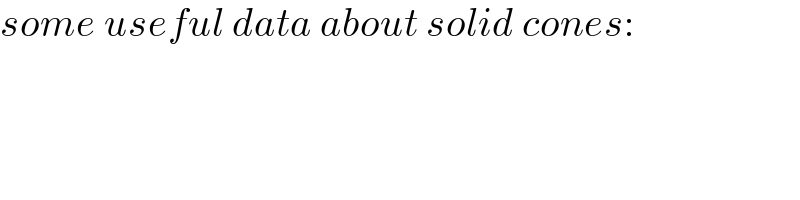
s o m e u s e f u l d a t a a b o u t s o l i d c o n e s :

Commented by mr W last updated on 06/Mar/21

Commented by mr W last updated on 07/Mar/21

Commented by mr W last updated on 07/Mar/21

O C = a x i s o f c o n e

O D = c o n t a c t l i n e o f c o n e o n p l a n e

G = c e n t e r o f m a s s o f c o n e

O C = h

O G = \frac{3}{4} h

O D = \sqrt{h^{2} + r^{2}}

\tan ρ = \frac{r}{h} \Rightarrow ρ = \tan^{- 1} \frac{r}{h}

G E ⊥ O D

G E = e = \frac{O G}{O D} \times r = \frac{3 h r}{4 \sqrt{h^{2} + r^{2}}}

O E = f = \frac{O G}{O D} \times h = \frac{3 h^{2}}{4 \sqrt{h^{2} + r^{2}}}

t h e p o s i t i o n o f t h e c o n e i s d e s c r i b e d

t h r o u g h θ w i t h - 90 ° ⩽ θ ⩽ 90 °, s i n c e

t h e c o n e i s r e l e a s e d f r o m r e s t a t

θ = - 90 ° .

s i n c e t h e s u r f a c e i s r o u g h e n o u g h

s u c h t h a t t h e c o n e c a n o n l y r o l l

o n t h e p l a n e w i t h o u t s l i p p i n g,

t h e c o n e r o t a t e s a b o u t i t s t i p p o i n t O

a l o n g t h e p l a n e . b e s i d e s i t r o t a t e s

a b o u t i t s o w n a x i s O C . s a y t h e

a n g u l a r s p e e d o f t h e m o t i o n a b o u t O

i s ω_{P} a n d t h a t a b o u t i t s a x i s i s ω_{a} .

ω_{P} = \frac{d θ}{d t} = ω

s i n c e t h e r e i s n o s l i p p i n g o n t h e

c o n t a c t,

O D \times ω_{P} = r \times ω_{a}

\Rightarrow ω_{a} = \frac{O D}{r} ω_{P} = \frac{ω}{\sin ρ}

ω_{P, s} = ω_{P} \cos ρ = ω \cos ρ

ω_{P, a} = - ω_{P} \sin ρ = - ω \sin ρ

w e s e e ω_{P, a} i s o p p o s i t e t o ω_{a}, t h e r e f o r e

t h e n e g a t i v e s i g n .

I_{a} = \frac{3 {m r}^{2}}{10}

I_{s} = \frac{3 m}{20} (r^{2} + 4 h^{2})

t h e k i n e t i c e n e r g y o f c o n e a t θ :

{K E}_{t} = \frac{1}{2} I_{a} {(ω_{a} + ω_{P, a})}^{2} + \frac{1}{2} I_{s} ω_{P, s}^{2}

{K E}_{t} = \frac{1}{2} \times \frac{3 {m r}^{2}}{10} \times {(\frac{1}{\sin ρ} - \sin ρ)}^{2} ω^{2} + \frac{1}{2} \times \frac{3 m}{20} (r^{2} + 4 h^{2}) \times \cos^{2} ρ \times ω^{2}

{K E}_{t} = \frac{3 m}{40} [(\frac{2}{\sin ρ} - \sin ρ) (\frac{1}{\sin ρ} - \sin ρ) r^{2} + 4 \cos^{2} ρ h^{2}] ω^{2}

{K E}_{t} = \frac{3 {m h}^{2}}{40} [(\frac{2}{\sin ρ} - \sin ρ) (\frac{1}{\sin ρ} - \sin ρ) \tan^{2} ρ + 4 \cos^{2} ρ] ω^{2}

{K E}_{t} = \frac{3 {m h}^{2}}{40} (1 + 5 \cos^{2} ρ) ω^{2}

l e t z_{O} = 0

a t t = 0 a n d θ = - 90 ° :

z_{E, 0} = 0

a t θ :

z_{E, t} = - O E \times \cos θ \times \sin ϕ = - f \cos θ \sin ϕ

{K E}_{t} = m g (z_{E, 0} - z_{E, t})

\frac{3 {m h}^{2}}{40} (1 + 5 \cos^{2} ρ) ω^{2} = m g f \cos θ \sin ϕ

(1 + 5 \cos^{2} ρ) ω^{2} = \frac{10 g}{\sqrt{h^{2} + r^{2}}} \times \cos θ \sin ϕ

ω^{2} = \frac{10 g \cos ρ}{h (1 + 5 \cos^{2} ρ)} \times \cos θ \sin ϕ

w i t h ξ = \sqrt{\frac{10 g \cos ρ}{h (1 + 5 \cos^{2} ρ)}}

\Rightarrow ω = ξ \sqrt{\sin ϕ \cos θ}

\frac{d θ}{d t} = ξ \sqrt{\sin ϕ \cos θ}

\int \frac{d θ}{\sqrt{\cos θ}} = ξ \sqrt{\sin ϕ} \int d t

l e t T = p e r i o d

\int_{0}^{\frac{π}{2}} \frac{d θ}{\sqrt{\cos θ}} = \frac{ξ \sqrt{\sin ϕ} T}{4}

\Rightarrow T = \frac{4}{ξ \sqrt{\sin ϕ}} \int_{0}^{\frac{π}{2}} \frac{d θ}{\sqrt{\cos θ}} = \frac{2 B (\frac{1}{4}, \frac{1}{2})}{ξ \sqrt{\sin ϕ}}

\Rightarrow T \approx \frac{10.48823}{ξ \sqrt{\sin ϕ}} = 3.31667 \sqrt{\frac{1 + 5 \cos^{2} ρ}{\cos ρ \sin ϕ}} \sqrt{\frac{h}{g}}

e x a m p l e :

ρ = 15 ° = \frac{π}{12}

ϕ = 30 ° = \frac{π}{6}

T \approx 11.359 \sqrt{\frac{h}{g}}

Commented by physicstutes last updated on 06/Mar/21

sir W please reference me to an e - book where

i can learn basic moment of inertia, especially things

like basic integral derivation of moment of inertia

for certain shapes (like rods, rectangles, rings etc) and

appling these knowledge to compound pendulum and swinging

masses . please i need help with those .

Commented by mr W last updated on 06/Mar/21

i r e a l l y {d o n}^{'} t k n o w s u c h a b o o k w h i c h

i c a n r e c o m m e n d . n o w a d a y s w h e n i

n e e d t o l o o k s o m e t h i n g, i j u s t g o o g l e .

Commented by I want to learn more last updated on 06/Mar/21

weldone sir .

Commented by physicstutes last updated on 06/Mar/21

i tried googling but could not find a suitable

pdf or even a book for the assesment .