Menu Close

Question-136043

Tinku Tara 0 Comments
Pin




Question Number 136043 by JulioCesar last updated on 18/Mar/21
Answered by rs4089 last updated on 18/Mar/21
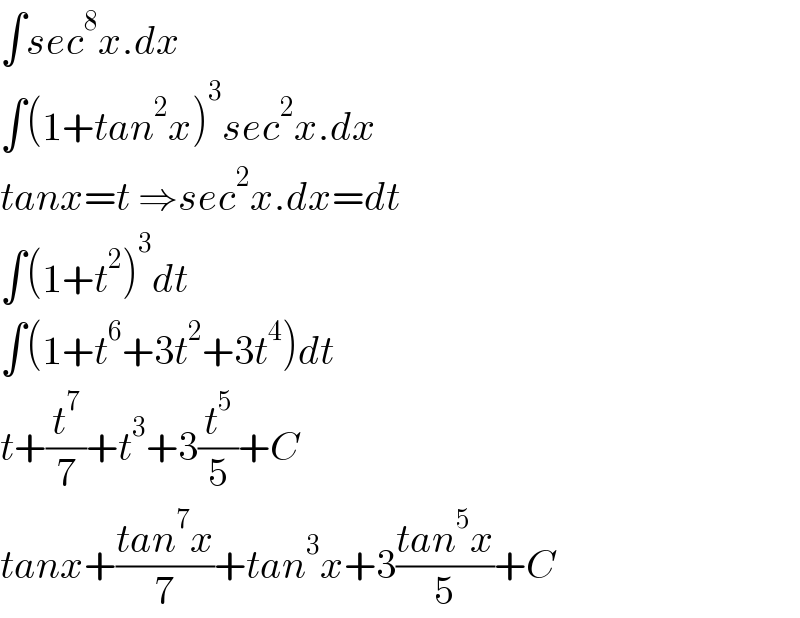
∫sec^8 x.dx ∫(1+tan^2 x)^3 sec^2 x.dx tanx=t ⇒sec^2 x.dx=dt ∫(1+t^2 )^3 dt ∫(1+t^6 +3t^2 +3t^4 )dt t+(t^7 /7)+t^3 +3(t^5 /5)+C tanx+((tan^7 x)/7)+tan^3 x+3((tan^5 x)/5)+C
$$\int{sec}^{\mathrm{8}} {x}.{dx} \\ $$$$\int\left(\mathrm{1}+{tan}^{\mathrm{2}} {x}\right)^{\mathrm{3}} {sec}^{\mathrm{2}} {x}.{dx} \\ $$$${tanx}={t}\:\Rightarrow{sec}^{\mathrm{2}} {x}.{dx}={dt} \\ $$$$\int\left(\mathrm{1}+{t}^{\mathrm{2}} \right)^{\mathrm{3}} {dt} \\ $$$$\int\left(\mathrm{1}+{t}^{\mathrm{6}} +\mathrm{3}{t}^{\mathrm{2}} +\mathrm{3}{t}^{\mathrm{4}} \right){dt} \\ $$$${t}+\frac{{t}^{\mathrm{7}} }{\mathrm{7}}+{t}^{\mathrm{3}} +\mathrm{3}\frac{{t}^{\mathrm{5}} }{\mathrm{5}}+{C} \\ $$$${tanx}+\frac{{tan}^{\mathrm{7}} {x}}{\mathrm{7}}+{tan}^{\mathrm{3}} {x}+\mathrm{3}\frac{{tan}^{\mathrm{5}} {x}}{\mathrm{5}}+{C} \\ $$
Answered by Olaf last updated on 18/Mar/21
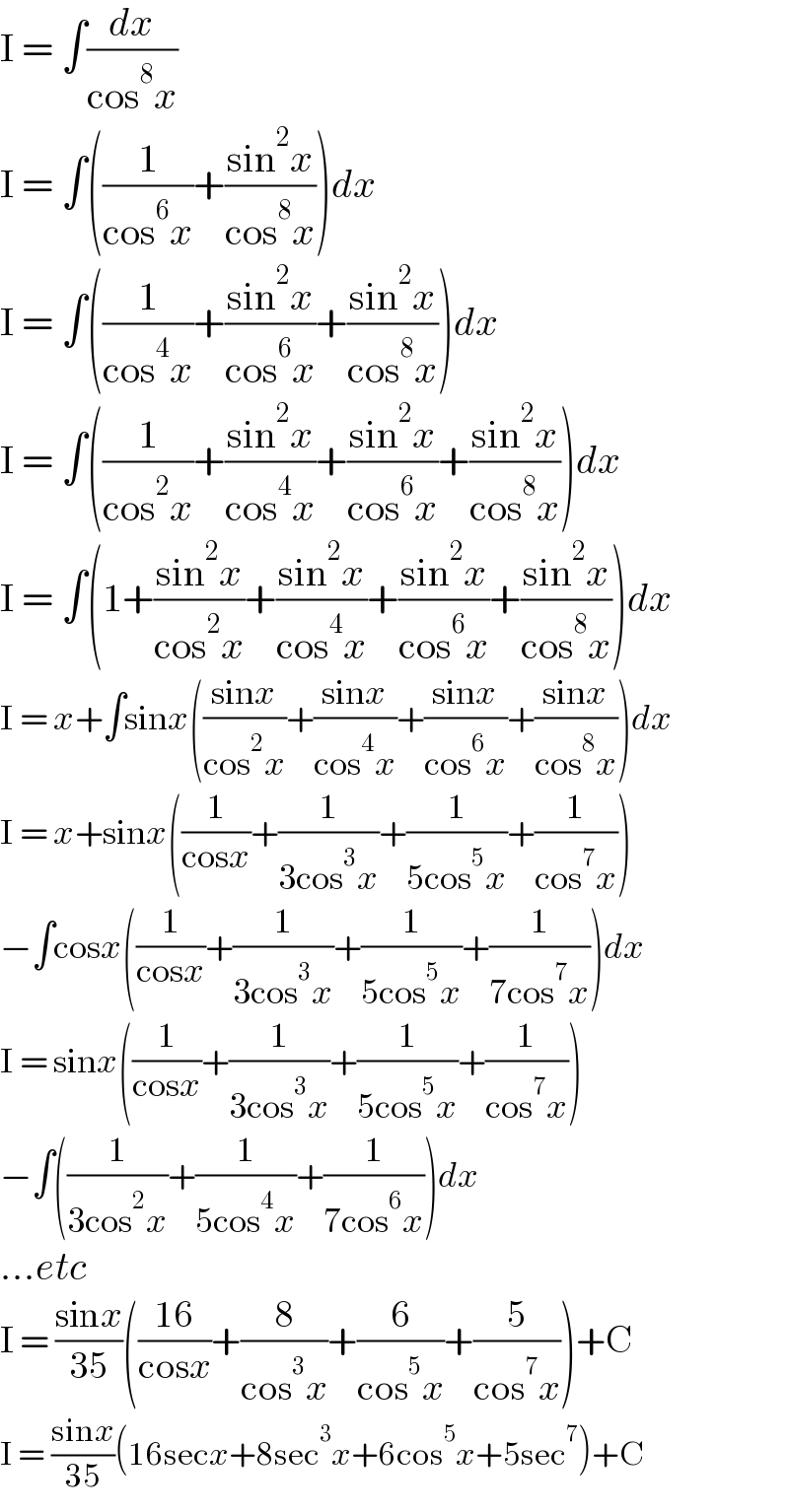
I = ∫(dx/(cos^8 x)) I = ∫((1/(cos^6 x))+((sin^2 x)/(cos^8 x)))dx I = ∫((1/(cos^4 x))+((sin^2 x)/(cos^6 x))+((sin^2 x)/(cos^8 x)))dx I = ∫((1/(cos^2 x))+((sin^2 x)/(cos^4 x))+((sin^2 x)/(cos^6 x))+((sin^2 x)/(cos^8 x)))dx I = ∫(1+((sin^2 x)/(cos^2 x))+((sin^2 x)/(cos^4 x))+((sin^2 x)/(cos^6 x))+((sin^2 x)/(cos^8 x)))dx I = x+∫sinx(((sinx)/(cos^2 x))+((sinx)/(cos^4 x))+((sinx)/(cos^6 x))+((sinx)/(cos^8 x)))dx I = x+sinx((1/(cosx))+(1/(3cos^3 x))+(1/(5cos^5 x))+(1/(cos^7 x))) −∫cosx((1/(cosx))+(1/(3cos^3 x))+(1/(5cos^5 x))+(1/(7cos^7 x)))dx I = sinx((1/(cosx))+(1/(3cos^3 x))+(1/(5cos^5 x))+(1/(cos^7 x))) −∫((1/(3cos^2 x))+(1/(5cos^4 x))+(1/(7cos^6 x)))dx ...etc I = ((sinx)/(35))(((16)/(cosx))+(8/(cos^3 x))+(6/(cos^5 x))+(5/(cos^7 x)))+C I = ((sinx)/(35))(16secx+8sec^3 x+6cos^5 x+5sec^7 )+C
$$\mathrm{I}\:=\:\int\frac{{dx}}{\mathrm{cos}^{\mathrm{8}} {x}} \\ $$$$\mathrm{I}\:=\:\int\left(\frac{\mathrm{1}}{\mathrm{cos}^{\mathrm{6}} {x}}+\frac{\mathrm{sin}^{\mathrm{2}} {x}}{\mathrm{cos}^{\mathrm{8}} {x}}\right){dx} \\ $$$$\mathrm{I}\:=\:\int\left(\frac{\mathrm{1}}{\mathrm{cos}^{\mathrm{4}} {x}}+\frac{\mathrm{sin}^{\mathrm{2}} {x}}{\mathrm{cos}^{\mathrm{6}} {x}}+\frac{\mathrm{sin}^{\mathrm{2}} {x}}{\mathrm{cos}^{\mathrm{8}} {x}}\right){dx} \\ $$$$\mathrm{I}\:=\:\int\left(\frac{\mathrm{1}}{\mathrm{cos}^{\mathrm{2}} {x}}+\frac{\mathrm{sin}^{\mathrm{2}} {x}}{\mathrm{cos}^{\mathrm{4}} {x}}+\frac{\mathrm{sin}^{\mathrm{2}} {x}}{\mathrm{cos}^{\mathrm{6}} {x}}+\frac{\mathrm{sin}^{\mathrm{2}} {x}}{\mathrm{cos}^{\mathrm{8}} {x}}\right){dx} \\ $$$$\mathrm{I}\:=\:\int\left(\mathrm{1}+\frac{\mathrm{sin}^{\mathrm{2}} {x}}{\mathrm{cos}^{\mathrm{2}} {x}}+\frac{\mathrm{sin}^{\mathrm{2}} {x}}{\mathrm{cos}^{\mathrm{4}} {x}}+\frac{\mathrm{sin}^{\mathrm{2}} {x}}{\mathrm{cos}^{\mathrm{6}} {x}}+\frac{\mathrm{sin}^{\mathrm{2}} {x}}{\mathrm{cos}^{\mathrm{8}} {x}}\right){dx} \\ $$$$\mathrm{I}\:=\:{x}+\int\mathrm{sin}{x}\left(\frac{\mathrm{sin}{x}}{\mathrm{cos}^{\mathrm{2}} {x}}+\frac{\mathrm{sin}{x}}{\mathrm{cos}^{\mathrm{4}} {x}}+\frac{\mathrm{sin}{x}}{\mathrm{cos}^{\mathrm{6}} {x}}+\frac{\mathrm{sin}{x}}{\mathrm{cos}^{\mathrm{8}} {x}}\right){dx} \\ $$$$\mathrm{I}\:=\:{x}+\mathrm{sin}{x}\left(\frac{\mathrm{1}}{\mathrm{cos}{x}}+\frac{\mathrm{1}}{\mathrm{3cos}^{\mathrm{3}} {x}}+\frac{\mathrm{1}}{\mathrm{5cos}^{\mathrm{5}} {x}}+\frac{\mathrm{1}}{\mathrm{cos}^{\mathrm{7}} {x}}\right) \\ $$$$−\int\mathrm{cos}{x}\left(\frac{\mathrm{1}}{\mathrm{cos}{x}}+\frac{\mathrm{1}}{\mathrm{3cos}^{\mathrm{3}} {x}}+\frac{\mathrm{1}}{\mathrm{5cos}^{\mathrm{5}} {x}}+\frac{\mathrm{1}}{\mathrm{7cos}^{\mathrm{7}} {x}}\right){dx} \\ $$$$\mathrm{I}\:=\:\mathrm{sin}{x}\left(\frac{\mathrm{1}}{\mathrm{cos}{x}}+\frac{\mathrm{1}}{\mathrm{3cos}^{\mathrm{3}} {x}}+\frac{\mathrm{1}}{\mathrm{5cos}^{\mathrm{5}} {x}}+\frac{\mathrm{1}}{\mathrm{cos}^{\mathrm{7}} {x}}\right) \\ $$$$−\int\left(\frac{\mathrm{1}}{\mathrm{3cos}^{\mathrm{2}} {x}}+\frac{\mathrm{1}}{\mathrm{5cos}^{\mathrm{4}} {x}}+\frac{\mathrm{1}}{\mathrm{7cos}^{\mathrm{6}} {x}}\right){dx} \\ $$$$…{etc} \\ $$$$\mathrm{I}\:=\:\frac{\mathrm{sin}{x}}{\mathrm{35}}\left(\frac{\mathrm{16}}{\mathrm{cos}{x}}+\frac{\mathrm{8}}{\mathrm{cos}^{\mathrm{3}} {x}}+\frac{\mathrm{6}}{\mathrm{cos}^{\mathrm{5}} {x}}+\frac{\mathrm{5}}{\mathrm{cos}^{\mathrm{7}} {x}}\right)+\mathrm{C} \\ $$$$\mathrm{I}\:=\:\frac{\mathrm{sin}{x}}{\mathrm{35}}\left(\mathrm{16sec}{x}+\mathrm{8sec}^{\mathrm{3}} {x}+\mathrm{6cos}^{\mathrm{5}} {x}+\mathrm{5sec}^{\mathrm{7}} \right)+\mathrm{C} \\ $$

Pin

Leave a Reply

Your email address will not be published. Required fields are marked *