Question Number 137173 by JulioCesar last updated on 30/Mar/21
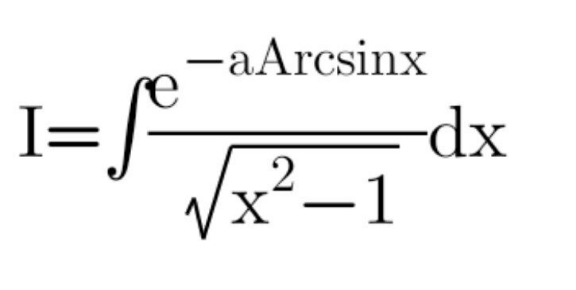
Commented by mathmax by abdo last updated on 31/Mar/21
![not defined arcsin is defined on [−1,1] but x^2 −1≤0 !](https://www.tinkutara.com/question/Q137200.png)
$$\mathrm{not}\:\mathrm{defined}\:\:\:\mathrm{arcsin}\:\mathrm{is}\:\mathrm{defined}\:\mathrm{on}\:\left[−\mathrm{1},\mathrm{1}\right]\:\:\mathrm{but}\:\mathrm{x}^{\mathrm{2}} −\mathrm{1}\leqslant\mathrm{0}\:! \\ $$
Answered by Ñï= last updated on 30/Mar/21
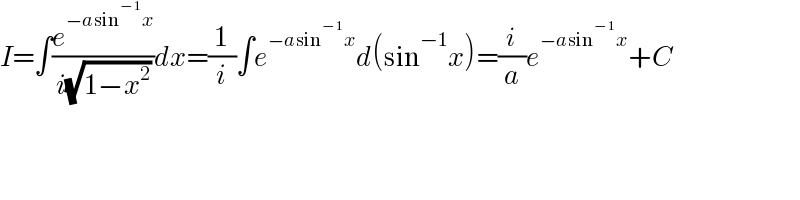
$${I}=\int\frac{{e}^{−{a}\mathrm{sin}^{−\mathrm{1}} {x}} }{{i}\sqrt{\mathrm{1}−{x}^{\mathrm{2}} }}{dx}=\frac{\mathrm{1}}{{i}}\int{e}^{−{a}\mathrm{sin}^{−\mathrm{1}} {x}} {d}\left(\mathrm{sin}^{−\mathrm{1}} {x}\right)=\frac{{i}}{{a}}{e}^{−{a}\mathrm{sin}^{−\mathrm{1}} {x}} +{C} \\ $$
