Question-138485

Question Number 138485 by SLVR last updated on 14/Apr/21

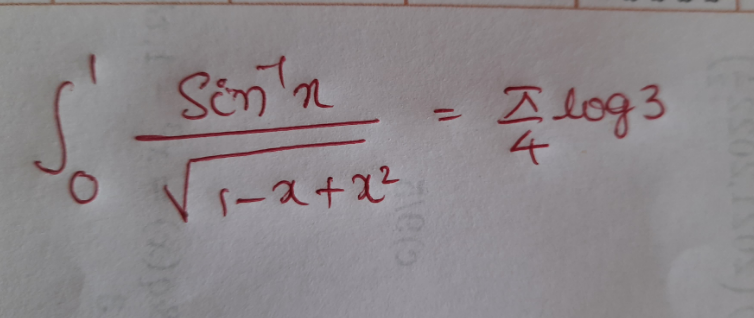
Commented by SLVR last updated on 14/Apr/21

G o o d m o r n i n g m r . W t h a n k s f o r

y o u r s u p p o r t . T h e a b o v e i s a l s o i

m i s s e d l o n g a g o . . a n d i c o u l d n o t

r e t r i v e f r o m t h e g r o u p . k i n d l y h e l p

Answered by phanphuoc last updated on 14/Apr/21

u = a r c x s i n x d v = 1 / \sqrt{1 - x + x^{2}} d x

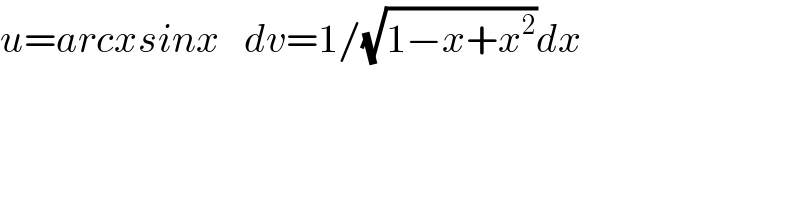
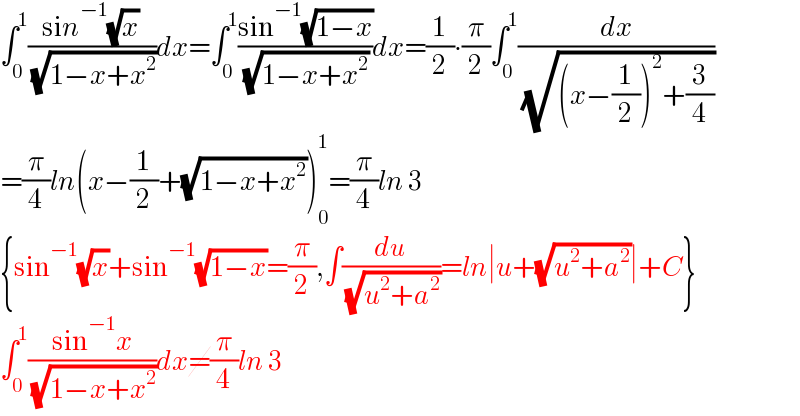
Answered by Ñï= last updated on 14/Apr/21

\int_{0}^{1} \frac{si n^{- 1} \sqrt{x}}{\sqrt{1 - x + x^{2}}} d x = \int_{0}^{1} \frac{\sin^{- 1} \sqrt{1 - x}}{\sqrt{1 - x + x^{2}}} d x = \frac{1}{2} \cdot \frac{π}{2} \int_{0}^{1} \frac{d x}{\sqrt{{(x - \frac{1}{2})}^{2} + \frac{3}{4}}}

= \frac{π}{4} l n {(x - \frac{1}{2} + \sqrt{1 - x + x^{2}})}_{0}^{1} = \frac{π}{4} l n 3

{\sin^{- 1} \sqrt{x} + \sin^{- 1} \sqrt{1 - x} = \frac{π}{2}, \int \frac{d u}{\sqrt{u^{2} + a^{2}}} = l n ∣ u + \sqrt{u^{2} + a^{2}} ∣ + C}

\int_{0}^{1} \frac{\sin^{- 1} x}{\sqrt{1 - x + x^{2}}} d x \cancel = \frac{π}{4} l n 3

Commented by SLVR last updated on 14/Apr/21

t h a n k s s i r \dots i a m w r o n g w r i t i n g

t h e q u e s t i o n .

Facebook Tweet Pin