Question Number 138621 by mnjuly1970 last updated on 15/Apr/21
Answered by Dwaipayan Shikari last updated on 15/Apr/21
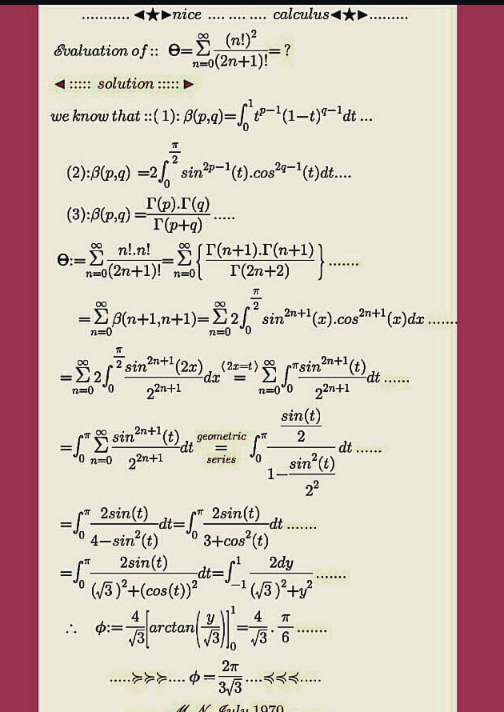
![Σ_(n=0) ^∞ ((Γ^2 (n+1))/(Γ(2n+2)))=∫_0 ^1 Σx^n (1−x)^n dx =∫_0 ^1 (1/(1−x+x^2 ))dx=∫_0 ^1 (1/((x−(1/2))^2 +(((√3)/2))^2 ))dx=(2/( (√3)))[tan^(−1) ((2x−1)/( (√3)))]_0 ^1 =(2/( (√3)))((π/6)+(π/6))=((2π)/( 3(√3)))](https://www.tinkutara.com/question/Q138623.png)
$$\underset{{n}=\mathrm{0}} {\overset{\infty} {\sum}}\frac{\Gamma^{\mathrm{2}} \left({n}+\mathrm{1}\right)}{\Gamma\left(\mathrm{2}{n}+\mathrm{2}\right)}=\int_{\mathrm{0}} ^{\mathrm{1}} \Sigma{x}^{{n}} \left(\mathrm{1}−{x}\right)^{{n}} {dx} \\ $$$$=\int_{\mathrm{0}} ^{\mathrm{1}} \frac{\mathrm{1}}{\mathrm{1}−{x}+{x}^{\mathrm{2}} }{dx}=\int_{\mathrm{0}} ^{\mathrm{1}} \frac{\mathrm{1}}{\left({x}−\frac{\mathrm{1}}{\mathrm{2}}\right)^{\mathrm{2}} +\left(\frac{\sqrt{\mathrm{3}}}{\mathrm{2}}\right)^{\mathrm{2}} }{dx}=\frac{\mathrm{2}}{\:\sqrt{\mathrm{3}}}\left[{tan}^{−\mathrm{1}} \frac{\mathrm{2}{x}−\mathrm{1}}{\:\sqrt{\mathrm{3}}}\right]_{\mathrm{0}} ^{\mathrm{1}} \\ $$$$=\frac{\mathrm{2}}{\:\sqrt{\mathrm{3}}}\left(\frac{\pi}{\mathrm{6}}+\frac{\pi}{\mathrm{6}}\right)=\frac{\mathrm{2}\pi}{\:\mathrm{3}\sqrt{\mathrm{3}}} \\ $$
Commented by mnjuly1970 last updated on 16/Apr/21
$$\:\:{thank}\:{you}\:{so}\:{much}… \\ $$
