Question Number 140738 by BHOOPENDRA last updated on 12/May/21

Commented by BHOOPENDRA last updated on 12/May/21

$${mr}.{W}\:{sir}? \\ $$
Commented by mr W last updated on 13/May/21
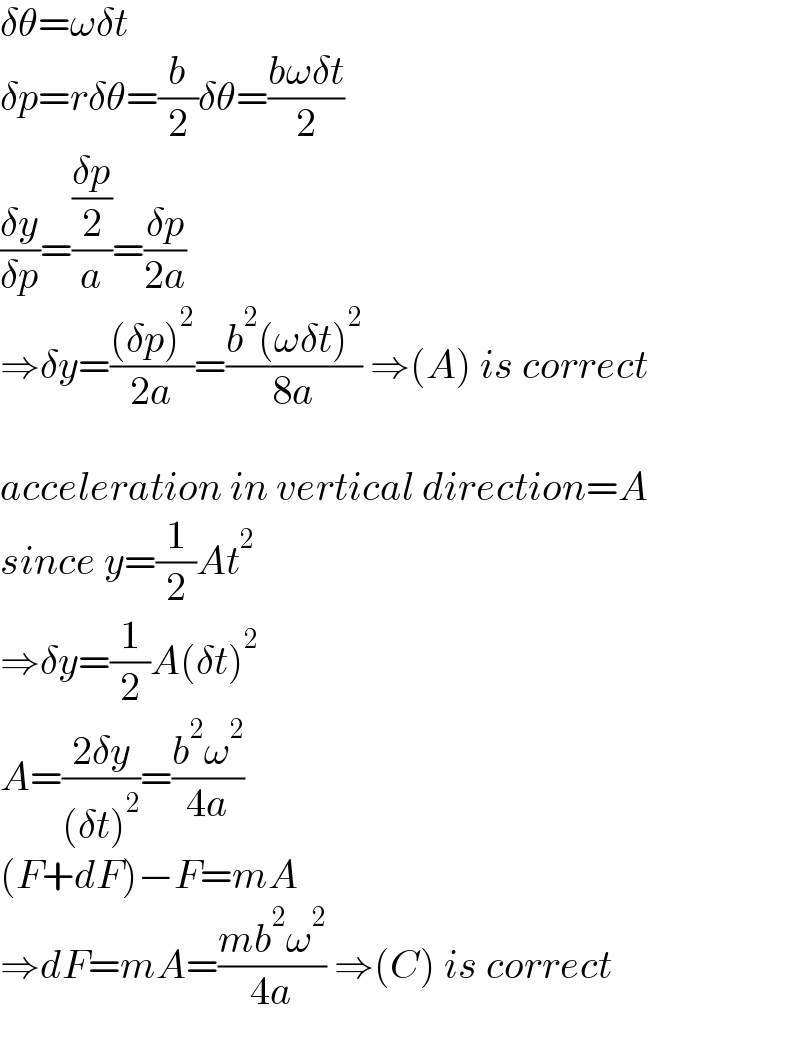
$$\delta\theta=\omega\delta{t} \\ $$$$\delta{p}={r}\delta\theta=\frac{{b}}{\mathrm{2}}\delta\theta=\frac{{b}\omega\delta{t}}{\mathrm{2}} \\ $$$$\frac{\delta{y}}{\delta{p}}=\frac{\frac{\delta{p}}{\mathrm{2}}}{{a}}=\frac{\delta{p}}{\mathrm{2}{a}} \\ $$$$\Rightarrow\delta{y}=\frac{\left(\delta{p}\right)^{\mathrm{2}} }{\mathrm{2}{a}}=\frac{{b}^{\mathrm{2}} \left(\omega\delta{t}\right)^{\mathrm{2}} }{\mathrm{8}{a}}\:\Rightarrow\left({A}\right)\:{is}\:{correct} \\ $$$$ \\ $$$${acceleration}\:{in}\:{vertical}\:{direction}={A} \\ $$$${since}\:{y}=\frac{\mathrm{1}}{\mathrm{2}}{At}^{\mathrm{2}} \\ $$$$\Rightarrow\delta{y}=\frac{\mathrm{1}}{\mathrm{2}}{A}\left(\delta{t}\right)^{\mathrm{2}} \\ $$$${A}=\frac{\mathrm{2}\delta{y}}{\left(\delta{t}\right)^{\mathrm{2}} }=\frac{{b}^{\mathrm{2}} \omega^{\mathrm{2}} }{\mathrm{4}{a}} \\ $$$$\left({F}+{dF}\right)−{F}={mA} \\ $$$$\Rightarrow{dF}={mA}=\frac{{mb}^{\mathrm{2}} \omega^{\mathrm{2}} }{\mathrm{4}{a}}\:\Rightarrow\left({C}\right)\:{is}\:{correct} \\ $$
Commented by BHOOPENDRA last updated on 13/May/21

$${option}\:{a}\:{and}\:{c}\:{will}\:{be}\:{correct}\: \\ $$$${and}\:{can}\:{you}\:{explain}\:{how}\:{you}\:{these}\:{equation}? \\ $$
Commented by mr W last updated on 13/May/21

