Question Number 141608 by cherokeesay last updated on 21/May/21

Commented by cherokeesay last updated on 21/May/21

$${the}\:{area}\:{hatched}\:? \\ $$
Answered by MJS_new last updated on 21/May/21
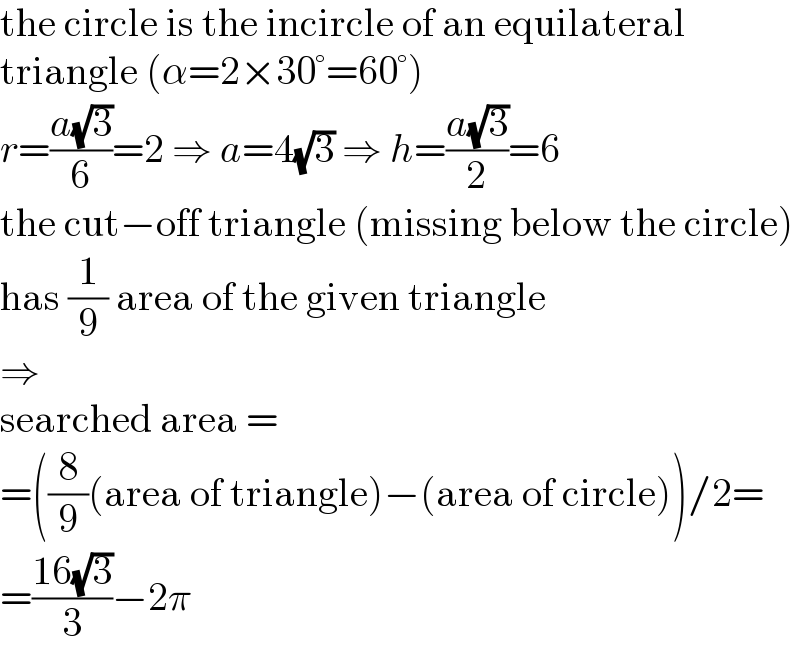
$$\mathrm{the}\:\mathrm{circle}\:\mathrm{is}\:\mathrm{the}\:\mathrm{incircle}\:\mathrm{of}\:\mathrm{an}\:\mathrm{equilateral} \\ $$$$\mathrm{triangle}\:\left(\alpha=\mathrm{2}×\mathrm{30}°=\mathrm{60}°\right) \\ $$$${r}=\frac{{a}\sqrt{\mathrm{3}}}{\mathrm{6}}=\mathrm{2}\:\Rightarrow\:{a}=\mathrm{4}\sqrt{\mathrm{3}}\:\Rightarrow\:{h}=\frac{{a}\sqrt{\mathrm{3}}}{\mathrm{2}}=\mathrm{6} \\ $$$$\mathrm{the}\:\mathrm{cut}−\mathrm{off}\:\mathrm{triangle}\:\left(\mathrm{missing}\:\mathrm{below}\:\mathrm{the}\:\mathrm{circle}\right) \\ $$$$\mathrm{has}\:\frac{\mathrm{1}}{\mathrm{9}}\:\mathrm{area}\:\mathrm{of}\:\mathrm{the}\:\mathrm{given}\:\mathrm{triangle} \\ $$$$\Rightarrow \\ $$$$\mathrm{searched}\:\mathrm{area}\:= \\ $$$$=\left(\frac{\mathrm{8}}{\mathrm{9}}\left(\mathrm{area}\:\mathrm{of}\:\mathrm{triangle}\right)−\left(\mathrm{area}\:\mathrm{of}\:\mathrm{circle}\right)\right)/\mathrm{2}= \\ $$$$=\frac{\mathrm{16}\sqrt{\mathrm{3}}}{\mathrm{3}}−\mathrm{2}\pi \\ $$
Commented by cherokeesay last updated on 21/May/21

$${very}\:{nice}\:! \\ $$$${thank}\:{you}\:! \\ $$
