Menu Close

Question-143367

Tinku Tara 0 Comments
Pin




Question Number 143367 by Sawadogo last updated on 13/Jun/21
Answered by TheHoneyCat last updated on 13/Jun/21
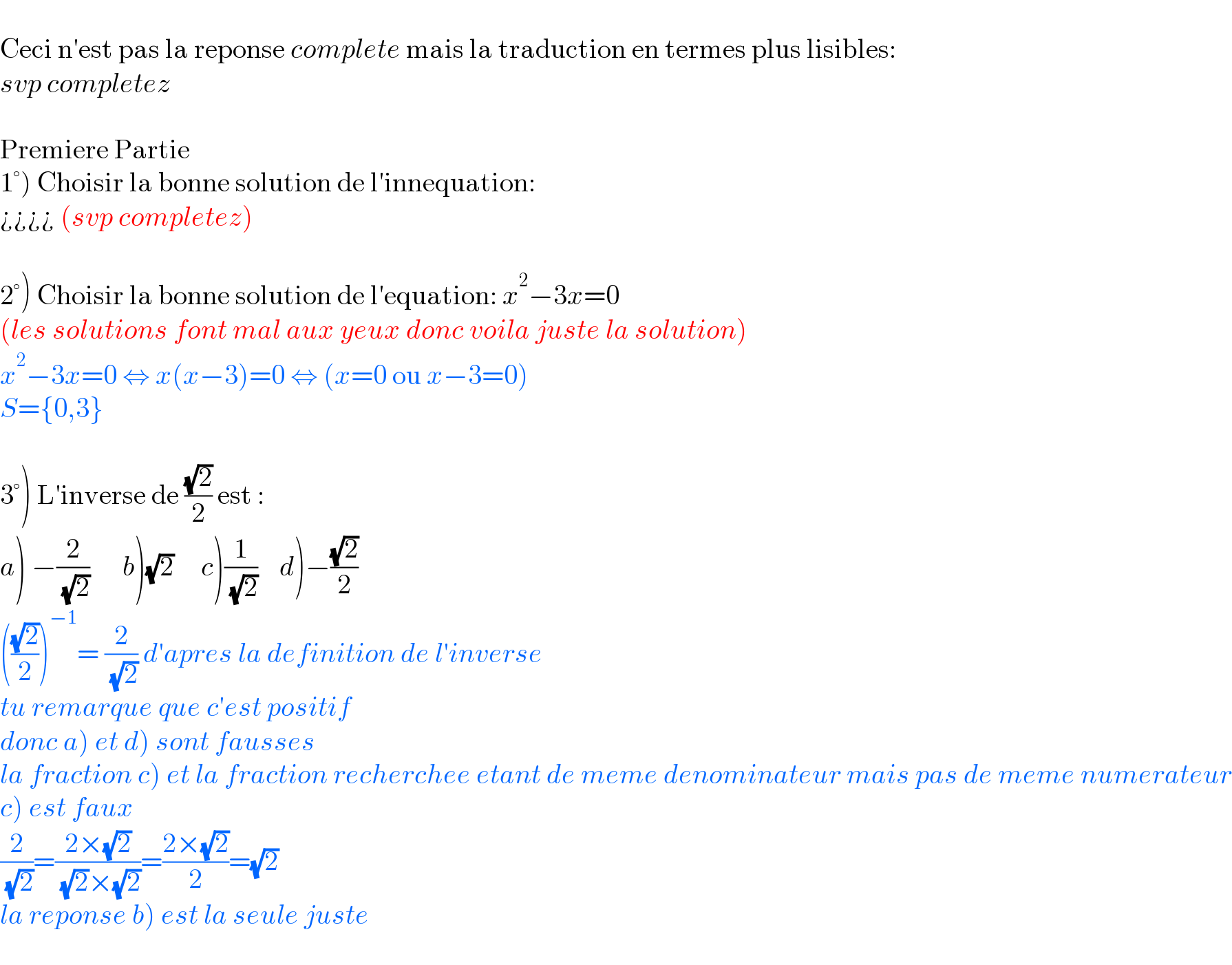
Ceci n′est pas la reponse complete mais la traduction en termes plus lisibles: svp completez Premiere Partie 1°) Choisir la bonne solution de l′innequation: ¿¿¿¿ (svp completez) 2°) Choisir la bonne solution de l′equation: x^2 −3x=0 (les solutions font mal aux yeux donc voila juste la solution) x^2 −3x=0 ⇔ x(x−3)=0 ⇔ (x=0 ou x−3=0) S={0,3} 3°) L′inverse de ((√2)/2) est : a) −(2/( (√2))) b)(√2) c)(1/( (√2))) d)−((√2)/2) (((√2)/2))^(−1) = (2/( (√2))) d′apres la definition de l′inverse tu remarque que c′est positif donc a) et d) sont fausses la fraction c) et la fraction recherchee etant de meme denominateur mais pas de meme numerateur c) est faux (2/( (√2)))=((2×(√2))/( (√2)×(√2)))=((2×(√2))/2)=(√2) la reponse b) est la seule juste
$$ \\ $$$$\mathrm{Ceci}\:\mathrm{n}'\mathrm{est}\:\mathrm{pas}\:\mathrm{la}\:\mathrm{reponse}\:{complete}\:\mathrm{mais}\:\mathrm{la}\:\mathrm{traduction}\:\mathrm{en}\:\mathrm{termes}\:\mathrm{plus}\:\mathrm{lisibles}: \\ $$$${svp}\:{completez} \\ $$$$ \\ $$$$\mathrm{Premiere}\:\mathrm{Partie} \\ $$$$\left.\mathrm{1}°\right)\:\mathrm{Choisir}\:\mathrm{la}\:\mathrm{bonne}\:\mathrm{solution}\:\mathrm{de}\:\mathrm{l}'\mathrm{innequation}: \\ $$$$¿¿¿¿\:\left({svp}\:{completez}\right) \\ $$$$ \\ $$$$\left.\mathrm{2}°\right)\:\mathrm{Choisir}\:\mathrm{la}\:\mathrm{bonne}\:\mathrm{solution}\:\mathrm{de}\:\mathrm{l}'\mathrm{equation}:\:{x}^{\mathrm{2}} −\mathrm{3}{x}=\mathrm{0} \\ $$$$\left({les}\:{solutions}\:{font}\:{mal}\:{aux}\:{yeux}\:{donc}\:{voila}\:{juste}\:{la}\:{solution}\right) \\ $$$${x}^{\mathrm{2}} −\mathrm{3}{x}=\mathrm{0}\:\Leftrightarrow\:{x}\left({x}−\mathrm{3}\right)=\mathrm{0}\:\Leftrightarrow\:\left({x}=\mathrm{0}\:\mathrm{ou}\:{x}−\mathrm{3}=\mathrm{0}\right) \\ $$$${S}=\left\{\mathrm{0},\mathrm{3}\right\} \\ $$$$ \\ $$$$\left.\mathrm{3}°\right)\:\mathrm{L}'\mathrm{inverse}\:\mathrm{de}\:\frac{\sqrt{\mathrm{2}}}{\mathrm{2}}\:\mathrm{est}\:: \\ $$$$\left.{a}\left.\right)\left.\:\left.−\frac{\mathrm{2}}{\:\sqrt{\mathrm{2}}}\:\:\:\:\:\:{b}\right)\sqrt{\mathrm{2}}\:\:\:\:\:{c}\right)\frac{\mathrm{1}}{\:\sqrt{\mathrm{2}}}\:\:\:\:{d}\right)−\frac{\sqrt{\mathrm{2}}}{\mathrm{2}} \\ $$$$\left(\frac{\sqrt{\mathrm{2}}}{\mathrm{2}}\right)^{−\mathrm{1}} =\:\frac{\mathrm{2}}{\:\sqrt{\mathrm{2}}}\:{d}'{apres}\:{la}\:{definition}\:{de}\:{l}'{inverse} \\ $$$${tu}\:{remarque}\:{que}\:{c}'{est}\:{positif} \\ $$$$\left.{d}\left.{onc}\:{a}\right)\:{et}\:{d}\right)\:{sont}\:{fausses} \\ $$$$\left.{la}\:{fraction}\:{c}\right)\:{et}\:{la}\:{fraction}\:{recherchee}\:{etant}\:{de}\:{meme}\:{denominateur}\:{mais}\:{pas}\:{de}\:{meme}\:{numerateur} \\ $$$$\left.{c}\right)\:{est}\:{faux} \\ $$$$\frac{\mathrm{2}}{\:\sqrt{\mathrm{2}}}=\frac{\mathrm{2}×\sqrt{\mathrm{2}}}{\:\sqrt{\mathrm{2}}×\sqrt{\mathrm{2}}}=\frac{\mathrm{2}×\sqrt{\mathrm{2}}}{\mathrm{2}}=\sqrt{\mathrm{2}} \\ $$$$\left.{la}\:{reponse}\:{b}\right)\:{est}\:{la}\:{seule}\:{juste} \\ $$$$ \\ $$
Answered by TheHoneyCat last updated on 13/Jun/21
4°) a=3(√5)−5(√3) signe: a=(√(3×3))(√5)−(√(5×5))(√3) =(√(3×3×5))−(√(3×5×5)) 0≤3<5 ⇔0≤ 3×3×5 <3×5×5⇔(√(3×3×5))<(√(3×5×5)) car (√.) est une gonction croissante a<0 a^2 =(3(√5))^2 −2×3(√5)×5(√3)+(5(√3))^2 =3×3×5−6×5×(√(15))+5×5×3 =15×(3+5)−30(√(15)) =120−30(√(15)) simplifier (√(95))−15(√(40))+3(√5) =(√(5×19))−3×5(√(4×2×5))+3(√5) =(√5)((√(19))−3(10(√2)+1)) je vois rien de mieux peut−t−etre ai−je mal lu l′ennonce 5°) { ((x+y=3,2)),((x−y=5,7)) :} ⇔ { ((2x=5,7 + 3,2)),((2y=3,2−5,7)) :} ⇔ { ((x=4,45)),((y=−1,25)) :}
$$\left.\mathrm{4}°\right)\:{a}=\mathrm{3}\sqrt{\mathrm{5}}−\mathrm{5}\sqrt{\mathrm{3}} \\ $$$${signe}: \\ $$$${a}=\sqrt{\mathrm{3}×\mathrm{3}}\sqrt{\mathrm{5}}−\sqrt{\mathrm{5}×\mathrm{5}}\sqrt{\mathrm{3}} \\ $$$$=\sqrt{\mathrm{3}×\mathrm{3}×\mathrm{5}}−\sqrt{\mathrm{3}×\mathrm{5}×\mathrm{5}} \\ $$$$\mathrm{0}\leqslant\mathrm{3}<\mathrm{5}\:\Leftrightarrow\mathrm{0}\leqslant\:\mathrm{3}×\mathrm{3}×\mathrm{5}\:<\mathrm{3}×\mathrm{5}×\mathrm{5}\Leftrightarrow\sqrt{\mathrm{3}×\mathrm{3}×\mathrm{5}}<\sqrt{\mathrm{3}×\mathrm{5}×\mathrm{5}}\:{car}\:\sqrt{.}\:{est}\:{une}\:{gonction}\:{croissante} \\ $$$${a}<\mathrm{0} \\ $$$$ \\ $$$${a}^{\mathrm{2}} =\left(\mathrm{3}\sqrt{\mathrm{5}}\right)^{\mathrm{2}} −\mathrm{2}×\mathrm{3}\sqrt{\mathrm{5}}×\mathrm{5}\sqrt{\mathrm{3}}+\left(\mathrm{5}\sqrt{\mathrm{3}}\right)^{\mathrm{2}} \\ $$$$=\mathrm{3}×\mathrm{3}×\mathrm{5}−\mathrm{6}×\mathrm{5}×\sqrt{\mathrm{15}}+\mathrm{5}×\mathrm{5}×\mathrm{3} \\ $$$$=\mathrm{15}×\left(\mathrm{3}+\mathrm{5}\right)−\mathrm{30}\sqrt{\mathrm{15}} \\ $$$$=\mathrm{120}−\mathrm{30}\sqrt{\mathrm{15}} \\ $$$$ \\ $$$${simplifier}\:\sqrt{\mathrm{95}}−\mathrm{15}\sqrt{\mathrm{40}}+\mathrm{3}\sqrt{\mathrm{5}} \\ $$$$=\sqrt{\mathrm{5}×\mathrm{19}}−\mathrm{3}×\mathrm{5}\sqrt{\mathrm{4}×\mathrm{2}×\mathrm{5}}+\mathrm{3}\sqrt{\mathrm{5}} \\ $$$$=\sqrt{\mathrm{5}}\left(\sqrt{\mathrm{19}}−\mathrm{3}\left(\mathrm{10}\sqrt{\mathrm{2}}+\mathrm{1}\right)\right) \\ $$$${je}\:{vois}\:{rien}\:{de}\:{mieux} \\ $$$${peut}−{t}−{etre}\:{ai}−{je}\:{mal}\:{lu}\:{l}'{ennonce} \\ $$$$ \\ $$$$\left.\mathrm{5}°\right)\:\begin{cases}{{x}+{y}=\mathrm{3},\mathrm{2}}\\{{x}−{y}=\mathrm{5},\mathrm{7}}\end{cases} \\ $$$$\Leftrightarrow\begin{cases}{\mathrm{2}{x}=\mathrm{5},\mathrm{7}\:+\:\mathrm{3},\mathrm{2}}\\{\mathrm{2}{y}=\mathrm{3},\mathrm{2}−\mathrm{5},\mathrm{7}}\end{cases} \\ $$$$\Leftrightarrow\begin{cases}{{x}=\mathrm{4},\mathrm{45}}\\{{y}=−\mathrm{1},\mathrm{25}}\end{cases} \\ $$

Pin

Leave a Reply

Your email address will not be published. Required fields are marked *