Question Number 143677 by bobhans last updated on 17/Jun/21

Answered by bemath last updated on 17/Jun/21
Answered by mathmax by abdo last updated on 17/Jun/21
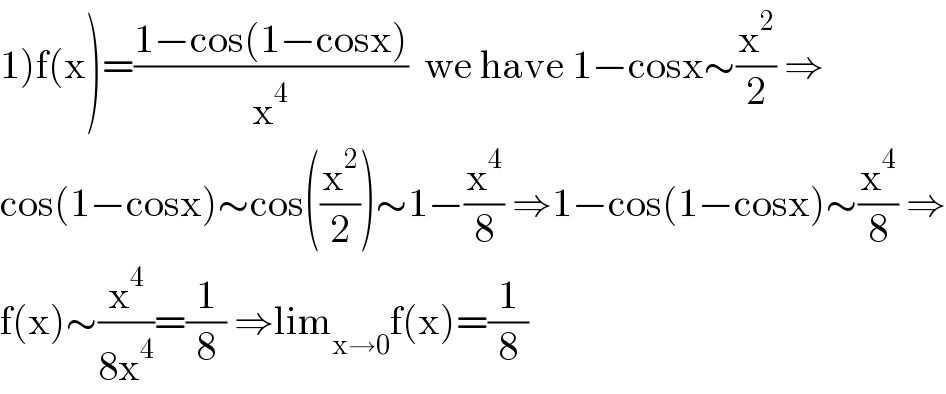
$$\left.\mathrm{1}\right)\mathrm{f}\left(\mathrm{x}\right)=\frac{\mathrm{1}−\mathrm{cos}\left(\mathrm{1}−\mathrm{cosx}\right)}{\mathrm{x}^{\mathrm{4}} }\:\:\mathrm{we}\:\mathrm{have}\:\mathrm{1}−\mathrm{cosx}\sim\frac{\mathrm{x}^{\mathrm{2}} }{\mathrm{2}}\:\Rightarrow \\ $$$$\mathrm{cos}\left(\mathrm{1}−\mathrm{cosx}\right)\sim\mathrm{cos}\left(\frac{\mathrm{x}^{\mathrm{2}} }{\mathrm{2}}\right)\sim\mathrm{1}−\frac{\mathrm{x}^{\mathrm{4}} }{\mathrm{8}}\:\Rightarrow\mathrm{1}−\mathrm{cos}\left(\mathrm{1}−\mathrm{cosx}\right)\sim\frac{\mathrm{x}^{\mathrm{4}} }{\mathrm{8}}\:\Rightarrow \\ $$$$\mathrm{f}\left(\mathrm{x}\right)\sim\frac{\mathrm{x}^{\mathrm{4}} }{\mathrm{8x}^{\mathrm{4}} }=\frac{\mathrm{1}}{\mathrm{8}}\:\Rightarrow\mathrm{lim}_{\mathrm{x}\rightarrow\mathrm{0}} \mathrm{f}\left(\mathrm{x}\right)=\frac{\mathrm{1}}{\mathrm{8}} \\ $$
Answered by mathmax by abdo last updated on 17/Jun/21
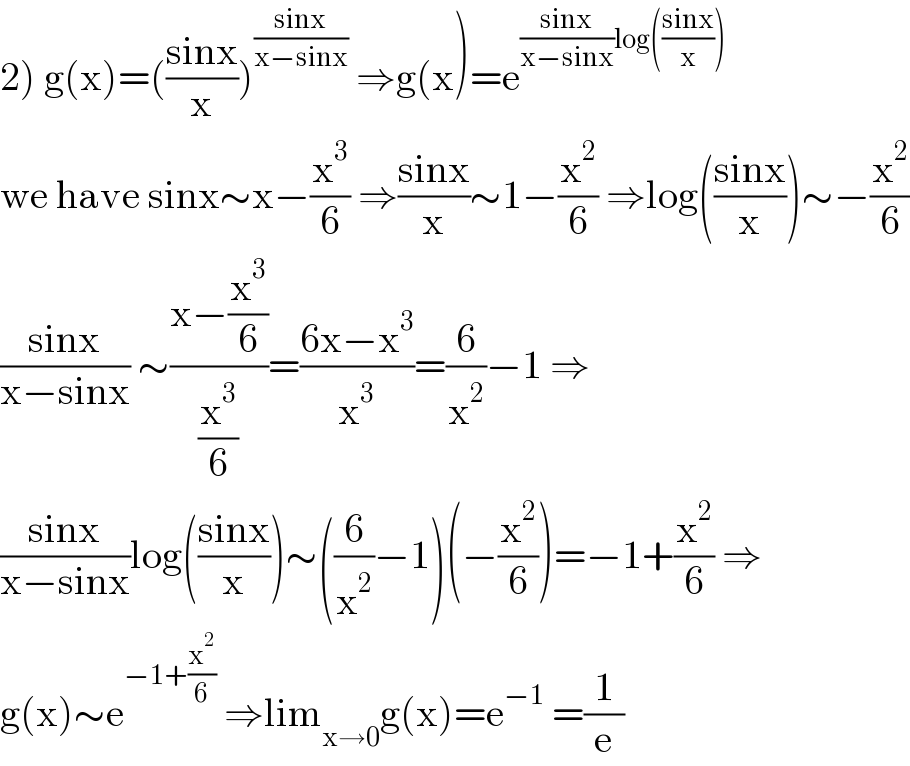
$$\left.\mathrm{2}\right)\:\mathrm{g}\left(\mathrm{x}\right)=\left(\frac{\mathrm{sinx}}{\mathrm{x}}\right)^{\frac{\mathrm{sinx}}{\mathrm{x}−\mathrm{sinx}}} \:\Rightarrow\mathrm{g}\left(\mathrm{x}\right)=\mathrm{e}^{\frac{\mathrm{sinx}}{\mathrm{x}−\mathrm{sinx}}\mathrm{log}\left(\frac{\mathrm{sinx}}{\mathrm{x}}\right)} \\ $$$$\mathrm{we}\:\mathrm{have}\:\mathrm{sinx}\sim\mathrm{x}−\frac{\mathrm{x}^{\mathrm{3}} }{\mathrm{6}}\:\Rightarrow\frac{\mathrm{sinx}}{\mathrm{x}}\sim\mathrm{1}−\frac{\mathrm{x}^{\mathrm{2}} }{\mathrm{6}}\:\Rightarrow\mathrm{log}\left(\frac{\mathrm{sinx}}{\mathrm{x}}\right)\sim−\frac{\mathrm{x}^{\mathrm{2}} }{\mathrm{6}} \\ $$$$\frac{\mathrm{sinx}}{\mathrm{x}−\mathrm{sinx}}\:\sim\frac{\mathrm{x}−\frac{\mathrm{x}^{\mathrm{3}} }{\mathrm{6}}}{\frac{\mathrm{x}^{\mathrm{3}} }{\mathrm{6}}}=\frac{\mathrm{6x}−\mathrm{x}^{\mathrm{3}} }{\mathrm{x}^{\mathrm{3}} }=\frac{\mathrm{6}}{\mathrm{x}^{\mathrm{2}} }−\mathrm{1}\:\Rightarrow \\ $$$$\frac{\mathrm{sinx}}{\mathrm{x}−\mathrm{sinx}}\mathrm{log}\left(\frac{\mathrm{sinx}}{\mathrm{x}}\right)\sim\left(\frac{\mathrm{6}}{\mathrm{x}^{\mathrm{2}} }−\mathrm{1}\right)\left(−\frac{\mathrm{x}^{\mathrm{2}} }{\mathrm{6}}\right)=−\mathrm{1}+\frac{\mathrm{x}^{\mathrm{2}} }{\mathrm{6}}\:\Rightarrow \\ $$$$\mathrm{g}\left(\mathrm{x}\right)\sim\mathrm{e}^{−\mathrm{1}+\frac{\mathrm{x}^{\mathrm{2}} }{\mathrm{6}}} \:\Rightarrow\mathrm{lim}_{\mathrm{x}\rightarrow\mathrm{0}} \mathrm{g}\left(\mathrm{x}\right)=\mathrm{e}^{−\mathrm{1}} \:=\frac{\mathrm{1}}{\mathrm{e}} \\ $$
