Question Number 5314 by sanusihammed last updated on 06/May/16

Commented by prakash jain last updated on 08/May/16
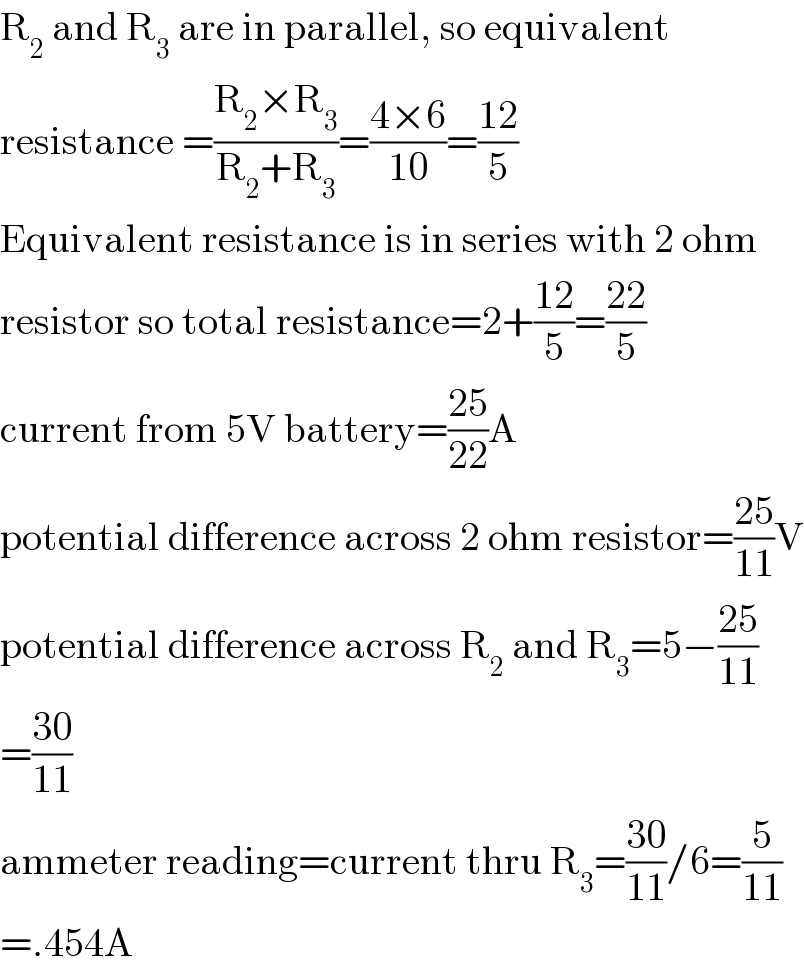
$$\mathrm{R}_{\mathrm{2}} \:\mathrm{and}\:\mathrm{R}_{\mathrm{3}} \:\mathrm{are}\:\mathrm{in}\:\mathrm{parallel},\:\mathrm{so}\:\mathrm{equivalent} \\ $$$$\mathrm{resistance}\:=\frac{\mathrm{R}_{\mathrm{2}} ×\mathrm{R}_{\mathrm{3}} }{\mathrm{R}_{\mathrm{2}} +\mathrm{R}_{\mathrm{3}} }=\frac{\mathrm{4}×\mathrm{6}}{\mathrm{10}}=\frac{\mathrm{12}}{\mathrm{5}} \\ $$$$\mathrm{Equivalent}\:\mathrm{resistance}\:\mathrm{is}\:\mathrm{in}\:\mathrm{series}\:\mathrm{with}\:\mathrm{2}\:\mathrm{ohm} \\ $$$$\mathrm{resistor}\:\mathrm{so}\:\mathrm{total}\:\mathrm{resistance}=\mathrm{2}+\frac{\mathrm{12}}{\mathrm{5}}=\frac{\mathrm{22}}{\mathrm{5}} \\ $$$$\mathrm{current}\:\mathrm{from}\:\mathrm{5V}\:\mathrm{battery}=\frac{\mathrm{25}}{\mathrm{22}}\mathrm{A} \\ $$$$\mathrm{potential}\:\mathrm{difference}\:\mathrm{across}\:\mathrm{2}\:\mathrm{ohm}\:\mathrm{resistor}=\frac{\mathrm{25}}{\mathrm{11}}\mathrm{V} \\ $$$$\mathrm{potential}\:\mathrm{difference}\:\mathrm{across}\:\mathrm{R}_{\mathrm{2}} \:\mathrm{and}\:\mathrm{R}_{\mathrm{3}} =\mathrm{5}−\frac{\mathrm{25}}{\mathrm{11}} \\ $$$$=\frac{\mathrm{30}}{\mathrm{11}} \\ $$$$\mathrm{ammeter}\:\mathrm{reading}=\mathrm{current}\:\mathrm{thru}\:\mathrm{R}_{\mathrm{3}} =\frac{\mathrm{30}}{\mathrm{11}}/\mathrm{6}=\frac{\mathrm{5}}{\mathrm{11}} \\ $$$$=.\mathrm{454A} \\ $$
