Menu Close

Question-5441

Tinku Tara 0 Comments
Pin




Question Number 5441 by Rasheed Soomro last updated on 15/May/16
Commented by Rasheed Soomro last updated on 15/May/16
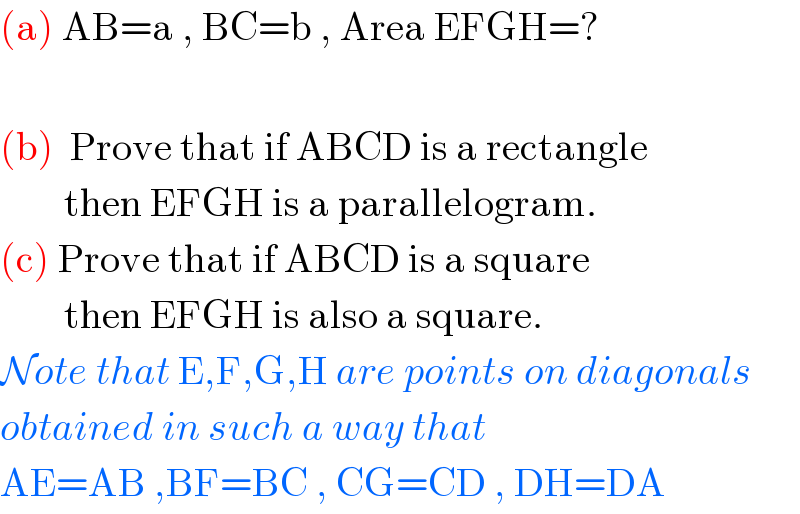
(a) AB=a , BC=b , Area EFGH=? (b) Prove that if ABCD is a rectangle then EFGH is a parallelogram. (c) Prove that if ABCD is a square then EFGH is also a square. Note that E,F,G,H are points on diagonals obtained in such a way that AE=AB ,BF=BC , CG=CD , DH=DA
$$\left(\mathrm{a}\right)\:\mathrm{AB}=\mathrm{a}\:,\:\mathrm{BC}=\mathrm{b}\:,\:\mathrm{Area}\:\mathrm{EFGH}=? \\ $$$$ \\ $$$$\left(\mathrm{b}\right)\:\:\mathrm{Prove}\:\mathrm{that}\:\mathrm{if}\:\mathrm{ABCD}\:\mathrm{is}\:\mathrm{a}\:\mathrm{rectangle}\: \\ $$$$\:\:\:\:\:\:\:\:\mathrm{then}\:\mathrm{EFGH}\:\mathrm{is}\:\mathrm{a}\:\mathrm{parallelogram}. \\ $$$$\left(\mathrm{c}\right)\:\mathrm{Prove}\:\mathrm{that}\:\mathrm{if}\:\mathrm{ABCD}\:\mathrm{is}\:\mathrm{a}\:\mathrm{square} \\ $$$$\:\:\:\:\:\:\:\:\mathrm{then}\:\mathrm{EFGH}\:\mathrm{is}\:\mathrm{also}\:\mathrm{a}\:\mathrm{square}. \\ $$$$\mathcal{N}{ote}\:{that}\:\mathrm{E},\mathrm{F},\mathrm{G},\mathrm{H}\:{are}\:{points}\:{on}\:{diagonals} \\ $$$${obtained}\:{in}\:{such}\:{a}\:{way}\:{that} \\ $$$$\mathrm{AE}=\mathrm{AB}\:,\mathrm{BF}=\mathrm{BC}\:,\:\mathrm{CG}=\mathrm{CD}\:,\:\mathrm{DH}=\mathrm{DA} \\ $$
Commented by Rasheed Soomro last updated on 15/May/16
^• What if ABCD would be a parallelogram, a quadrilateral ? ^• It is interesting to place some restrictions on ABCD and to see an effect on EFGH! For example ABCD may be non-convex,self intersecting, two sides parallel etc.
$$\:^{\bullet} \mathrm{What}\:\mathrm{if}\:\mathrm{ABCD}\:\:\mathrm{would}\:\mathrm{be}\:\mathrm{a}\:\mathrm{parallelogram},\:\mathrm{a}\:\mathrm{quadrilateral}\:? \\ $$$$\:^{\bullet} \mathrm{It}\:\mathrm{is}\:\mathrm{interesting}\:\mathrm{to}\:\mathrm{place}\:\mathrm{some}\:\mathrm{restrictions}\:\mathrm{on} \\ $$$$\mathrm{ABCD}\:\mathrm{and}\:\mathrm{to}\:\mathrm{see}\:\mathrm{an}\:\mathrm{effect}\:\mathrm{on}\:\mathrm{EFGH}!\:\mathrm{For}\:\mathrm{example} \\ $$$$\mathrm{ABCD}\:\mathrm{may}\:\mathrm{be}\:\mathrm{non}-\mathrm{convex},\mathrm{self}\:\mathrm{intersecting},\:\mathrm{two} \\ $$$$\mathrm{sides}\:\mathrm{parallel}\:\mathrm{etc}. \\ $$
Commented by Yozzii last updated on 15/May/16
I′ll try it soon. I′ve been busy.
$${I}'{ll}\:{try}\:{it}\:{soon}.\:{I}'{ve}\:{been}\:{busy}. \\ $$
Commented by Yozzii last updated on 17/May/16
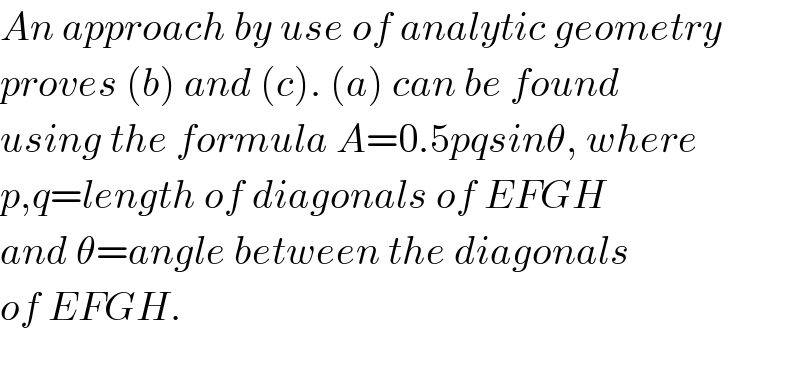
An approach by use of analytic geometry proves (b) and (c). (a) can be found using the formula A=0.5pqsinθ, where p,q=length of diagonals of EFGH and θ=angle between the diagonals of EFGH.
$${An}\:{approach}\:{by}\:{use}\:{of}\:{analytic}\:{geometry}\: \\ $$$${proves}\:\left({b}\right)\:{and}\:\left({c}\right).\:\left({a}\right)\:{can}\:{be}\:{found}\: \\ $$$${using}\:{the}\:{formula}\:{A}=\mathrm{0}.\mathrm{5}{pqsin}\theta,\:{where} \\ $$$${p},{q}={length}\:{of}\:{diagonals}\:{of}\:{EFGH}\: \\ $$$${and}\:\theta={angle}\:{between}\:{the}\:{diagonals} \\ $$$${of}\:{EFGH}. \\ $$$$ \\ $$

Pin

Leave a Reply

Your email address will not be published. Required fields are marked *