Menu Close

Question-69569

Tinku Tara 0 Comments
Pin




Question Number 69569 by Ajao yinka last updated on 25/Sep/19
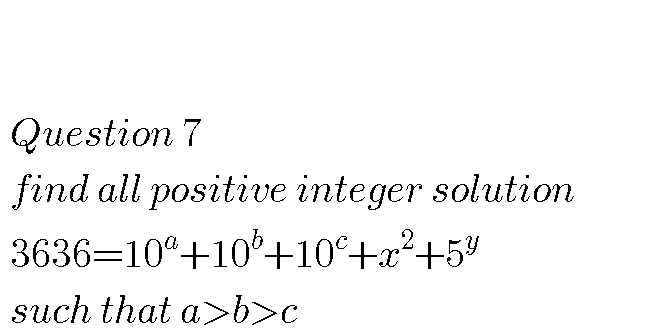
Answered by naka3546 last updated on 25/Sep/19
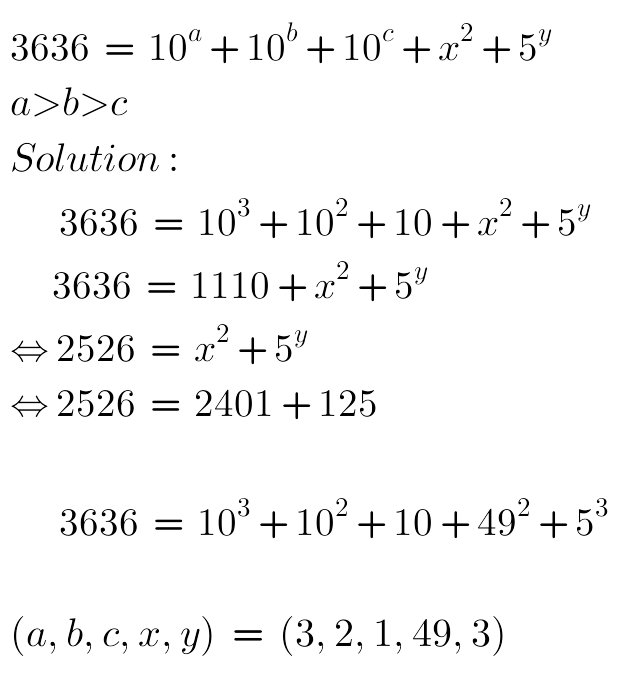
Commented by Rasheed.Sindhi last updated on 26/Sep/19
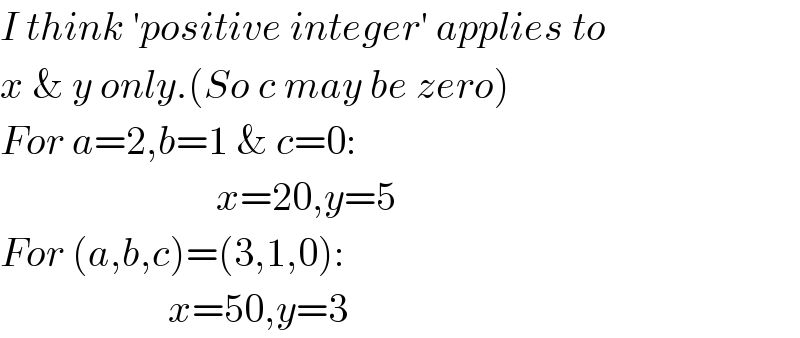
I think ′positive integer′ applies to x & y only.(So c may be zero) For a=2,b=1 & c=0: x=20,y=5 For (a,b,c)=(3,1,0): x=50,y=3
$${I}\:{think}\:'{positive}\:{integer}'\:{applies}\:{to} \\ $$$${x}\:\&\:{y}\:{only}.\left({So}\:{c}\:{may}\:{be}\:{zero}\right) \\ $$$${For}\:{a}=\mathrm{2},{b}=\mathrm{1}\:\&\:{c}=\mathrm{0}: \\ $$$$\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:{x}=\mathrm{20},{y}=\mathrm{5} \\ $$$${For}\:\left({a},{b},{c}\right)=\left(\mathrm{3},\mathrm{1},\mathrm{0}\right): \\ $$$$\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:{x}=\mathrm{50},{y}=\mathrm{3} \\ $$
Commented by Ajao yinka last updated on 26/Sep/19
cool
$${cool} \\ $$
Answered by Rasheed.Sindhi last updated on 27/Sep/19
10^a ≤3636⇒a<4 a>b>c: possibilities for (a,b,c) (3,2,1),(3,2,0),(3,1,0),(2,1,0) (a,b,c)=(3,2,1) _(−) 10^3 +10^2 +10^1 +x^2 +5^y =3636 x^2 +5^y =3636−1110=2526 x=(√(2526−5^y )) Testing for y=1,2,3,... x∈Z^+ only for y=3 ∴ for (a,b,c)=(3,2,1): y=3,x=49 (a,b,c)=(3,2,0) _(−) 10^3 +10^2 +10^0 +x^2 +5^y =3636 x^2 +5^y =3636−1101=2535 x=(√(2535−5^y )) x is positive integer for no value of y. ∴ No solution in (a,b,c)=(3,2,0). (a,b,c)=(3,1,0) _(−) 10^3 +10^1 +10^0 +x^2 +5^y =3636 x=(√(2625−5^y )) y=3⇒x=50 (only positive integers) ∴ for (a,b,c)=(3,1,0):y=3,x=50 (a,b,c)=(2,1,0) _(−) 10^2 +10^1 +10^1 +x^2 +5^y =3636 x=(√(3525−5^y )) y=5⇒x=20 (only positive integers) ∴for (a,b,c)=(2,1,0): y=5,x=20 { (((a,b,c)=(3,2,1):(x,y)=(49,3))),(((a,b,c)=(3,2,0):(x,y)=φ)),(((a,b,c)=(3,1,0):(x,y)=(50,3))),(((a,b,c)=(2,1,0):(x,y)=(20,5))) :}
$$\:\:\:\mathrm{10}^{{a}} \leqslant\mathrm{3636}\Rightarrow{a}<\mathrm{4} \\ $$$${a}>{b}>{c}: \\ $$$${possibilities}\:{for}\:\left({a},{b},{c}\right) \\ $$$$\left(\mathrm{3},\mathrm{2},\mathrm{1}\right),\left(\mathrm{3},\mathrm{2},\mathrm{0}\right),\left(\mathrm{3},\mathrm{1},\mathrm{0}\right),\left(\mathrm{2},\mathrm{1},\mathrm{0}\right) \\ $$$$\:\:\:\:\:\:\:\:\underset{−} {\:\:\:\:\:\:\left({a},{b},{c}\right)=\left(\mathrm{3},\mathrm{2},\mathrm{1}\right)\:\:\:\:\:} \\ $$$$\:\:\:\:\mathrm{10}^{\mathrm{3}} +\mathrm{10}^{\mathrm{2}} +\mathrm{10}^{\mathrm{1}} +{x}^{\mathrm{2}} +\mathrm{5}^{{y}} =\mathrm{3636} \\ $$$$\:\:\:\:\:{x}^{\mathrm{2}} +\mathrm{5}^{{y}} =\mathrm{3636}−\mathrm{1110}=\mathrm{2526} \\ $$$$\:\:\:\:\:\:\:{x}=\sqrt{\mathrm{2526}−\mathrm{5}^{{y}} } \\ $$$${Testing}\:{for}\:{y}=\mathrm{1},\mathrm{2},\mathrm{3},… \\ $$$${x}\in\mathbb{Z}^{+} \:{only}\:{for}\:{y}=\mathrm{3} \\ $$$$\therefore\:{for}\:\left({a},{b},{c}\right)=\left(\mathrm{3},\mathrm{2},\mathrm{1}\right):\:{y}=\mathrm{3},{x}=\mathrm{49} \\ $$$$\:\:\:\:\:\:\:\:\underset{−} {\:\:\:\:\:\:\left({a},{b},{c}\right)=\left(\mathrm{3},\mathrm{2},\mathrm{0}\right)\:\:\:\:\:} \\ $$$$\:\:\mathrm{10}^{\mathrm{3}} +\mathrm{10}^{\mathrm{2}} +\mathrm{10}^{\mathrm{0}} +{x}^{\mathrm{2}} +\mathrm{5}^{{y}} =\mathrm{3636} \\ $$$$\:\:\:\:\:{x}^{\mathrm{2}} +\mathrm{5}^{{y}} =\mathrm{3636}−\mathrm{1101}=\mathrm{2535} \\ $$$$\:\:\:\:\:\:\:{x}=\sqrt{\mathrm{2535}−\mathrm{5}^{{y}} } \\ $$$${x}\:{is}\:{positive}\:{integer}\:{for}\:{no}\:{value}\:{of}\:{y}. \\ $$$$\therefore\:{No}\:{solution}\:{in}\:\left({a},{b},{c}\right)=\left(\mathrm{3},\mathrm{2},\mathrm{0}\right). \\ $$$$\:\:\:\:\:\:\:\:\underset{−} {\:\:\:\:\:\:\left({a},{b},{c}\right)=\left(\mathrm{3},\mathrm{1},\mathrm{0}\right)\:\:\:\:\:} \\ $$$$\:\:\:\mathrm{10}^{\mathrm{3}} +\mathrm{10}^{\mathrm{1}} +\mathrm{10}^{\mathrm{0}} +{x}^{\mathrm{2}} +\mathrm{5}^{{y}} =\mathrm{3636} \\ $$$$\:\:\:\:\:\:\:{x}=\sqrt{\mathrm{2625}−\mathrm{5}^{{y}} } \\ $$$${y}=\mathrm{3}\Rightarrow{x}=\mathrm{50}\:\left({only}\:{positive}\:{integers}\right) \\ $$$$\therefore\:{for}\:\left({a},{b},{c}\right)=\left(\mathrm{3},\mathrm{1},\mathrm{0}\right):{y}=\mathrm{3},{x}=\mathrm{50} \\ $$$$\:\:\:\:\:\:\:\:\underset{−} {\:\:\:\:\:\:\left({a},{b},{c}\right)=\left(\mathrm{2},\mathrm{1},\mathrm{0}\right)\:\:\:\:\:} \\ $$$$\:\:\mathrm{10}^{\mathrm{2}} +\mathrm{10}^{\mathrm{1}} +\mathrm{10}^{\mathrm{1}} +{x}^{\mathrm{2}} +\mathrm{5}^{{y}} =\mathrm{3636} \\ $$$$\:\:\:\:\:\:\:{x}=\sqrt{\mathrm{3525}−\mathrm{5}^{{y}} } \\ $$$$\:\:{y}=\mathrm{5}\Rightarrow{x}=\mathrm{20}\:\left({only}\:{positive}\:{integers}\right)\: \\ $$$$\therefore{for}\:\left({a},{b},{c}\right)=\left(\mathrm{2},\mathrm{1},\mathrm{0}\right):\:{y}=\mathrm{5},{x}=\mathrm{20} \\ $$$$ \\ $$$$\begin{cases}{\left({a},{b},{c}\right)=\left(\mathrm{3},\mathrm{2},\mathrm{1}\right):\left({x},{y}\right)=\left(\mathrm{49},\mathrm{3}\right)}\\{\left({a},{b},{c}\right)=\left(\mathrm{3},\mathrm{2},\mathrm{0}\right):\left({x},{y}\right)=\phi}\\{\left({a},{b},{c}\right)=\left(\mathrm{3},\mathrm{1},\mathrm{0}\right):\left({x},{y}\right)=\left(\mathrm{50},\mathrm{3}\right)}\\{\left({a},{b},{c}\right)=\left(\mathrm{2},\mathrm{1},\mathrm{0}\right):\left({x},{y}\right)=\left(\mathrm{20},\mathrm{5}\right)}\end{cases} \\ $$$$ \\ $$
Commented by Ajao yinka last updated on 27/Sep/19
i said positive integer solution,zero is not a positive integer
$${i}\:{said}\:{positive}\:{integer}\:{solution},{zero}\:{is}\:{not}\:{a}\:{positive}\:{integer} \\ $$
Commented by Rasheed.Sindhi last updated on 27/Sep/19
a,b & c are arbitrary constants and x & y are variables. The condition “positive integersip” are aplicable to variables here.
$${a},{b}\:\&\:{c}\:{are}\:\boldsymbol{{arbitrary}}\:\boldsymbol{{constant}}{s}\:{and}\:{x}\:\&\:{y}\:{are} \\ $$$${variables}.\:{The}\:{condition}\:“{positive}\:{integersip}'' \\ $$$${are}\:{aplicable}\:{to}\:{variables}\:{here}. \\ $$

Pin

Leave a Reply

Your email address will not be published. Required fields are marked *