Question Number 69574 by Ajao yinka last updated on 25/Sep/19

Answered by MJS last updated on 25/Sep/19

$$\mathrm{36}_{\mathrm{7}} =\mathrm{27}_{\mathrm{10}} \\ $$$$\mathrm{27}_{\mathrm{10}} !=\mathrm{10}\:\mathrm{888}\:\mathrm{869}\:\mathrm{450}\:\mathrm{418}\:\mathrm{352}\:\mathrm{160}\:\mathrm{768}\:\mathrm{000}\:\mathrm{000}_{\mathrm{10}} = \\ $$$$\mathrm{36}_{\mathrm{7}} !=\mathrm{1}\:\mathrm{260}\:\mathrm{051}\:\mathrm{015}\:\mathrm{522}\:\mathrm{245}\:\mathrm{130}\:\mathrm{363}\:\mathrm{455}\:\mathrm{463}\:\mathrm{066}\:\mathrm{000}_{\mathrm{7}} \\ $$$$\Rightarrow\:\mathrm{the}\:\mathrm{last}\:\mathrm{five}\:\mathrm{digits}\:\mathrm{are}\:\mathrm{66000} \\ $$
Commented by Ajao yinka last updated on 26/Sep/19
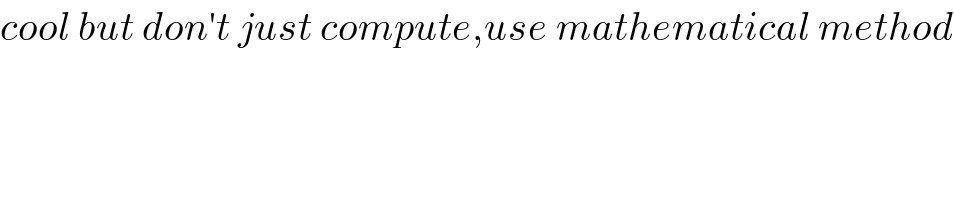
$${cool}\:{but}\:{don}'{t}\:{just}\:{compute},{use}\:{mathematical}\:{method} \\ $$
