Question Number 7063 by Tawakalitu. last updated on 08/Aug/16

Commented by Tawakalitu. last updated on 08/Aug/16
Commented by Yozzii last updated on 08/Aug/16
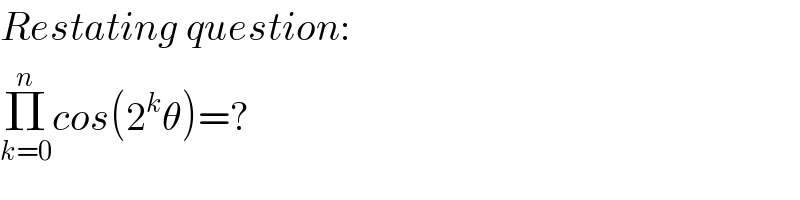
Commented by Yozzii last updated on 08/Aug/16
![u=cosθ cos2θ cos4θ cos8θ ×...× cos2^(n−1) θ cos2^n θ Let u(n)=Π_(k=0) ^n cos(2^k θ) Observing the result of u(n) for n=0,1,2,3 u(0)=cosθ=(1/2^0 )cosθ u(1)=cosθcos2θ=(1/2)(cos3θ+cosθ) u(2)=cosθcos2θcos4θ=0.5(0.5[cos7θ+cosθ+cos5θ+cos3θ])=(1/2^2 )[cosθ+cos3θ+cos5θ+cos7θ] u(3)=cosθcos2θcos4θcos8θ=(1/2^2 )[cos7θcos8θ+cos5θcos8θ+cos3θcos8θ+cosθcos8θ] u(3)=(1/2^3 )(cos15θ+cosθ+cos13θ+cos3θ+cos11θ+cos5θ+cos9θ+cos7θ) u(3)=(1/2^3 )(cosθ+cos3θ+cos5θ+cos7θ+cos9θ+cos11θ+cos13θ+cos15θ) I conject that u(n)=(1/2^n )Σ_(i=1) ^2^n cos[(2i−1)θ] u(n)sinθ=(1/2^n )Σ_(i=1) ^2^n sinθcos(2i−1)θ cos(2i−1)θsinθ=0.5{sin(2i)θ−sin(2i−2)θ} Let f(i)=sin2iθ 1≤i≤2^n i∈N ∴ u(n)sinθ=(1/2^(n+1) )Σ_(i=1) ^2^n {f(i)−f(i−1)} u(n)sinθ=(1/2^(n+1) )({f(1)−f(0)}+{f(2)−f(1)}+{f(3)−f(2)}+...+{f(2^n −2)−f(2^n −3)}+{f(2^n −1)−f(2^n −2)}+{f(2^n )−f(2^n −1)}) u(n)sinθ=(1/2^(n+1) )[f(2^n )−f(0)] u(n)=((sin2×2^n θ−sin(0×θ))/(2^(n+1) sinθ)) u(n)=((sin2^(n+1) θ)/(2^(n+1) sinθ)) ⇒Π_(k=0) ^n cos(2^k θ)=((sin2^(n+1) θ)/(2^(n+1) sinθ)) (θ≠mπ) n=0,1,2,3,... By induction, this formular is correct.](https://www.tinkutara.com/question/Q7069.png)
Commented by Tawakalitu. last updated on 09/Aug/16
Answered by Yozzii last updated on 08/Aug/16