Question Number 70847 by A8;15: last updated on 08/Oct/19
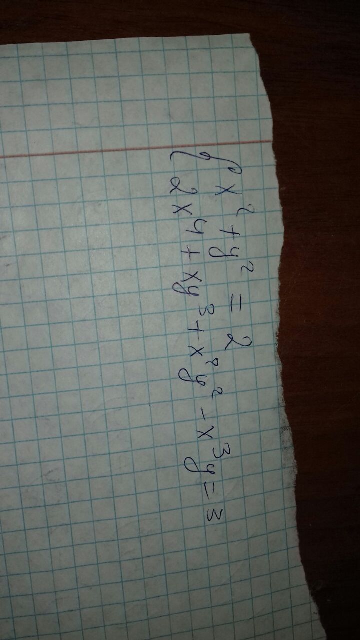
Answered by mind is power last updated on 08/Oct/19
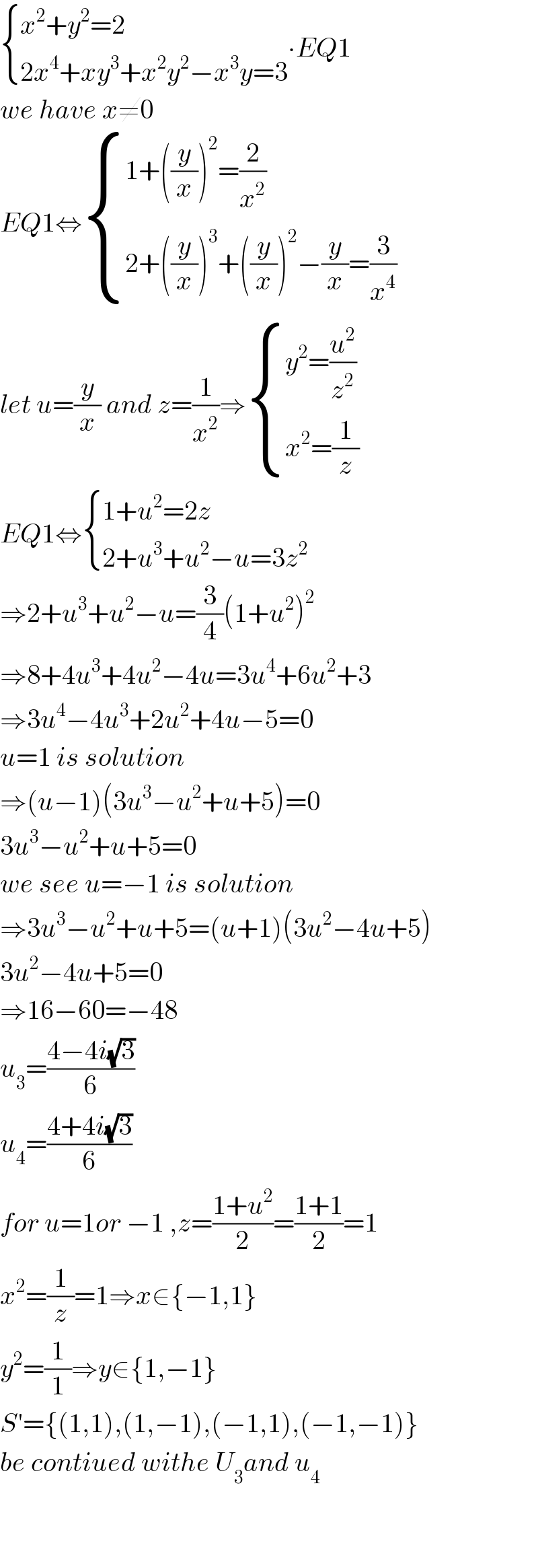
$$\begin{cases}{{x}^{\mathrm{2}} +{y}^{\mathrm{2}} =\mathrm{2}}\\{\mathrm{2}{x}^{\mathrm{4}} +{xy}^{\mathrm{3}} +{x}^{\mathrm{2}} {y}^{\mathrm{2}} −{x}^{\mathrm{3}} {y}=\mathrm{3}}\end{cases}\centerdot{EQ}\mathrm{1} \\ $$$${we}\:{have}\:{x}\neq\mathrm{0} \\ $$$${EQ}\mathrm{1}\Leftrightarrow\begin{cases}{\mathrm{1}+\left(\frac{{y}}{{x}}\right)^{\mathrm{2}} =\frac{\mathrm{2}}{{x}^{\mathrm{2}} }}\\{\mathrm{2}+\left(\frac{{y}}{{x}}\right)^{\mathrm{3}} +\left(\frac{{y}}{{x}}\right)^{\mathrm{2}} −\frac{{y}}{{x}}=\frac{\mathrm{3}}{{x}^{\mathrm{4}} }}\end{cases}_{} \\ $$$${let}\:{u}=\frac{{y}}{{x}}\:{and}\:{z}=\frac{\mathrm{1}}{{x}^{\mathrm{2}} }\Rightarrow\begin{cases}{{y}^{\mathrm{2}} =\frac{{u}^{\mathrm{2}} }{{z}^{\mathrm{2}} }}\\{{x}^{\mathrm{2}} =\frac{\mathrm{1}}{{z}}}\end{cases} \\ $$$${EQ}\mathrm{1}\Leftrightarrow\begin{cases}{\mathrm{1}+{u}^{\mathrm{2}} =\mathrm{2}{z}}\\{\mathrm{2}+{u}^{\mathrm{3}} +{u}^{\mathrm{2}} −{u}=\mathrm{3}{z}^{\mathrm{2}} }\end{cases} \\ $$$$\Rightarrow\mathrm{2}+{u}^{\mathrm{3}} +{u}^{\mathrm{2}} −{u}=\frac{\mathrm{3}}{\mathrm{4}}\left(\mathrm{1}+{u}^{\mathrm{2}} \right)^{\mathrm{2}} \\ $$$$\Rightarrow\mathrm{8}+\mathrm{4}{u}^{\mathrm{3}} +\mathrm{4}{u}^{\mathrm{2}} −\mathrm{4}{u}=\mathrm{3}{u}^{\mathrm{4}} +\mathrm{6}{u}^{\mathrm{2}} +\mathrm{3} \\ $$$$\Rightarrow\mathrm{3}{u}^{\mathrm{4}} −\mathrm{4}{u}^{\mathrm{3}} +\mathrm{2}{u}^{\mathrm{2}} +\mathrm{4}{u}−\mathrm{5}=\mathrm{0} \\ $$$${u}=\mathrm{1}\:{is}\:{solution} \\ $$$$\Rightarrow\left({u}−\mathrm{1}\right)\left(\mathrm{3}{u}^{\mathrm{3}} −{u}^{\mathrm{2}} +{u}+\mathrm{5}\right)=\mathrm{0} \\ $$$$\mathrm{3}{u}^{\mathrm{3}} −{u}^{\mathrm{2}} +{u}+\mathrm{5}=\mathrm{0} \\ $$$${we}\:{see}\:{u}=−\mathrm{1}\:{is}\:{solution} \\ $$$$\Rightarrow\mathrm{3}{u}^{\mathrm{3}} −{u}^{\mathrm{2}} +{u}+\mathrm{5}=\left({u}+\mathrm{1}\right)\left(\mathrm{3}{u}^{\mathrm{2}} −\mathrm{4}{u}+\mathrm{5}\right) \\ $$$$\mathrm{3}{u}^{\mathrm{2}} −\mathrm{4}{u}+\mathrm{5}=\mathrm{0} \\ $$$$\Rightarrow\mathrm{16}−\mathrm{60}=−\mathrm{48} \\ $$$${u}_{\mathrm{3}} =\frac{\mathrm{4}−\mathrm{4}{i}\sqrt{\mathrm{3}}}{\mathrm{6}} \\ $$$${u}_{\mathrm{4}} =\frac{\mathrm{4}+\mathrm{4}{i}\sqrt{\mathrm{3}}}{\mathrm{6}} \\ $$$${for}\:{u}=\mathrm{1}{or}\:−\mathrm{1}\:,{z}=\frac{\mathrm{1}+{u}^{\mathrm{2}} }{\mathrm{2}}=\frac{\mathrm{1}+\mathrm{1}}{\mathrm{2}}=\mathrm{1} \\ $$$${x}^{\mathrm{2}} =\frac{\mathrm{1}}{{z}}=\mathrm{1}\Rightarrow{x}\in\left\{−\mathrm{1},\mathrm{1}\right\} \\ $$$${y}^{\mathrm{2}} =\frac{\mathrm{1}}{\mathrm{1}}\Rightarrow{y}\in\left\{\mathrm{1},−\mathrm{1}\right\} \\ $$$${S}'=\left\{\left(\mathrm{1},\mathrm{1}\right),\left(\mathrm{1},−\mathrm{1}\right),\left(−\mathrm{1},\mathrm{1}\right),\left(−\mathrm{1},−\mathrm{1}\right)\right\} \\ $$$${be}\:{contiued}\:{withe}\:{U}_{\mathrm{3}} {and}\:{u}_{\mathrm{4}} \\ $$$$ \\ $$$$ \\ $$
Commented by MJS last updated on 08/Oct/19
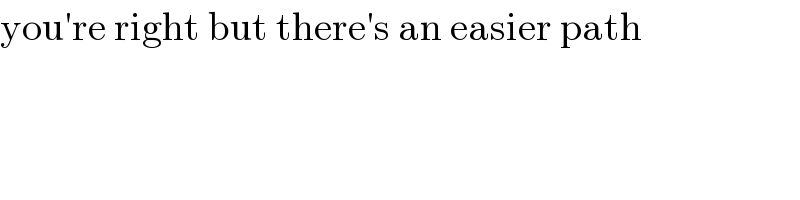
$$\mathrm{you}'\mathrm{re}\:\mathrm{right}\:\mathrm{but}\:\mathrm{there}'\mathrm{s}\:\mathrm{an}\:\mathrm{easier}\:\mathrm{path} \\ $$
Commented by mind is power last updated on 08/Oct/19

$${yeah}\:,{isee}\:{your}\:\:{solution}\:{Sir}\:\:{its}\:{better} \\ $$
Commented by MJS last updated on 08/Oct/19
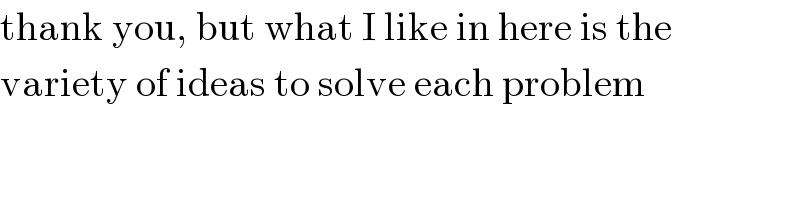
$$\mathrm{thank}\:\mathrm{you},\:\mathrm{but}\:\mathrm{what}\:\mathrm{I}\:\mathrm{like}\:\mathrm{in}\:\mathrm{here}\:\mathrm{is}\:\mathrm{the} \\ $$$$\mathrm{variety}\:\mathrm{of}\:\mathrm{ideas}\:\mathrm{to}\:\mathrm{solve}\:\mathrm{each}\:\mathrm{problem} \\ $$
Answered by MJS last updated on 08/Oct/19
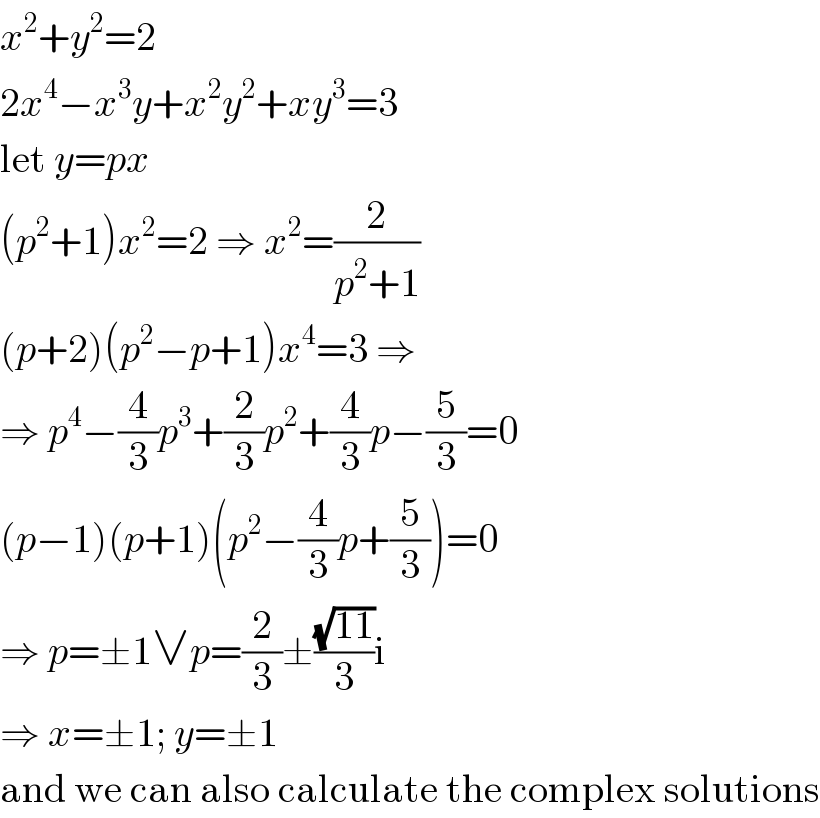
$${x}^{\mathrm{2}} +{y}^{\mathrm{2}} =\mathrm{2} \\ $$$$\mathrm{2}{x}^{\mathrm{4}} −{x}^{\mathrm{3}} {y}+{x}^{\mathrm{2}} {y}^{\mathrm{2}} +{xy}^{\mathrm{3}} =\mathrm{3} \\ $$$$\mathrm{let}\:{y}={px} \\ $$$$\left({p}^{\mathrm{2}} +\mathrm{1}\right){x}^{\mathrm{2}} =\mathrm{2}\:\Rightarrow\:{x}^{\mathrm{2}} =\frac{\mathrm{2}}{{p}^{\mathrm{2}} +\mathrm{1}} \\ $$$$\left({p}+\mathrm{2}\right)\left({p}^{\mathrm{2}} −{p}+\mathrm{1}\right){x}^{\mathrm{4}} =\mathrm{3}\:\Rightarrow \\ $$$$\Rightarrow\:{p}^{\mathrm{4}} −\frac{\mathrm{4}}{\mathrm{3}}{p}^{\mathrm{3}} +\frac{\mathrm{2}}{\mathrm{3}}{p}^{\mathrm{2}} +\frac{\mathrm{4}}{\mathrm{3}}{p}−\frac{\mathrm{5}}{\mathrm{3}}=\mathrm{0} \\ $$$$\left({p}−\mathrm{1}\right)\left({p}+\mathrm{1}\right)\left({p}^{\mathrm{2}} −\frac{\mathrm{4}}{\mathrm{3}}{p}+\frac{\mathrm{5}}{\mathrm{3}}\right)=\mathrm{0} \\ $$$$\Rightarrow\:{p}=\pm\mathrm{1}\vee{p}=\frac{\mathrm{2}}{\mathrm{3}}\pm\frac{\sqrt{\mathrm{11}}}{\mathrm{3}}\mathrm{i} \\ $$$$\Rightarrow\:{x}=\pm\mathrm{1};\:{y}=\pm\mathrm{1} \\ $$$$\mathrm{and}\:\mathrm{we}\:\mathrm{can}\:\mathrm{also}\:\mathrm{calculate}\:\mathrm{the}\:\mathrm{complex}\:\mathrm{solutions} \\ $$
