Question Number 71051 by jagannath19 last updated on 11/Oct/19
Commented by jagannath19 last updated on 11/Oct/19

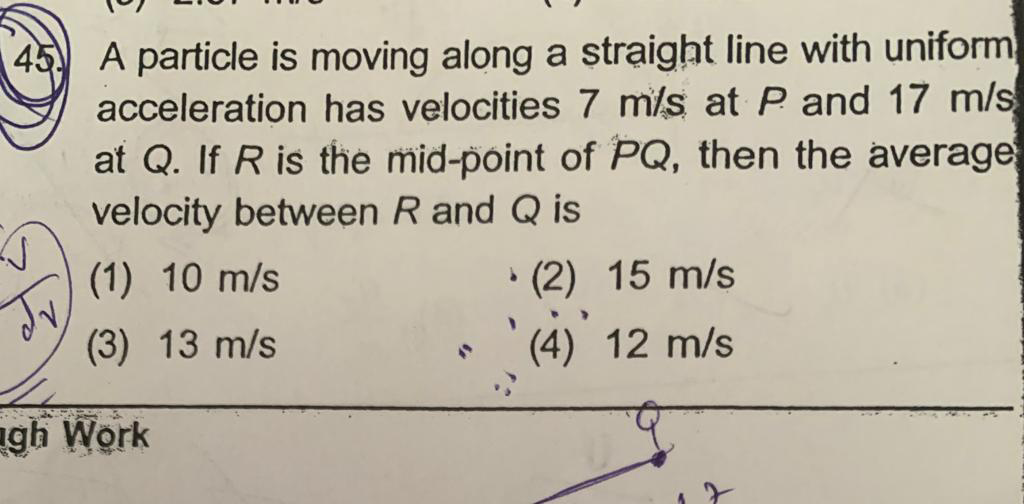
$${explain} \\ $$
Commented by mr W last updated on 11/Oct/19
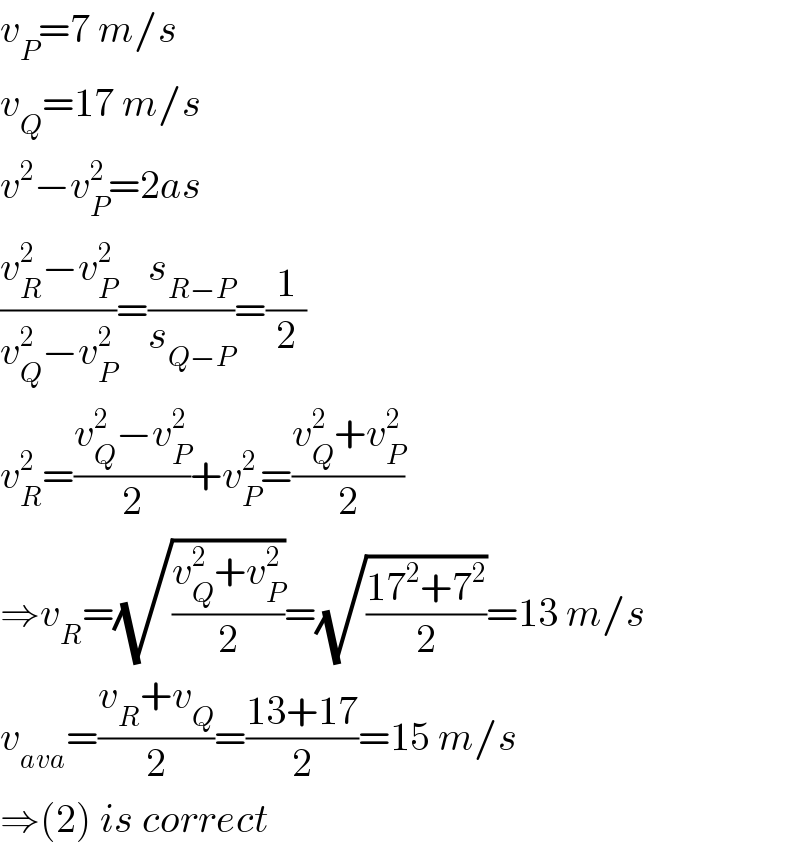
$${v}_{{P}} =\mathrm{7}\:{m}/{s} \\ $$$${v}_{{Q}} =\mathrm{17}\:{m}/{s} \\ $$$${v}^{\mathrm{2}} −{v}_{{P}} ^{\mathrm{2}} =\mathrm{2}{as} \\ $$$$\frac{{v}_{{R}} ^{\mathrm{2}} −{v}_{{P}} ^{\mathrm{2}} }{{v}_{{Q}} ^{\mathrm{2}} −{v}_{{P}} ^{\mathrm{2}} }=\frac{{s}_{{R}−{P}} }{{s}_{{Q}−{P}} }=\frac{\mathrm{1}}{\mathrm{2}} \\ $$$${v}_{{R}} ^{\mathrm{2}} =\frac{{v}_{{Q}} ^{\mathrm{2}} −{v}_{{P}} ^{\mathrm{2}} }{\mathrm{2}}+{v}_{{P}} ^{\mathrm{2}} =\frac{{v}_{{Q}} ^{\mathrm{2}} +{v}_{{P}} ^{\mathrm{2}} }{\mathrm{2}} \\ $$$$\Rightarrow{v}_{{R}} =\sqrt{\frac{{v}_{{Q}} ^{\mathrm{2}} +{v}_{{P}} ^{\mathrm{2}} }{\mathrm{2}}}=\sqrt{\frac{\mathrm{17}^{\mathrm{2}} +\mathrm{7}^{\mathrm{2}} }{\mathrm{2}}}=\mathrm{13}\:{m}/{s} \\ $$$${v}_{{ava}} =\frac{{v}_{{R}} +{v}_{{Q}} }{\mathrm{2}}=\frac{\mathrm{13}+\mathrm{17}}{\mathrm{2}}=\mathrm{15}\:{m}/{s} \\ $$$$\Rightarrow\left(\mathrm{2}\right)\:{is}\:{correct} \\ $$
