Question Number 73909 by Pk1167156@gmail.com last updated on 16/Nov/19

Commented by Pk1167156@gmail.com last updated on 16/Nov/19
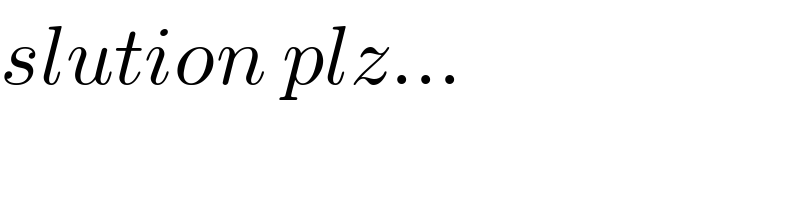
$${slution}\:{plz}… \\ $$
Answered by arkanmath7@gmail.com last updated on 16/Nov/19

$${it}'{s}\:{just}\:\infty\:{I}\:{think} \\ $$$${bcz}\:{ur}\:{qst}\:= \\ $$$$ \\ $$$$−\mathrm{4}^{\left(−\mathrm{4}\right)\left(−\mathrm{4}\right)\left(−\mathrm{4}\right)…\infty} \:=−\mathrm{4}^{\infty\:} =\:\infty \\ $$
Answered by mr W last updated on 17/Nov/19

$$−\mathrm{4}^{−\mathrm{4}^{−\mathrm{4}^{−\mathrm{4}^{…} } } } ={x} \\ $$$$\left(−\mathrm{4}\right)^{{x}} ={x}\:\in\:\mathbb{C} \\ $$$$\left(\mathrm{4}{e}^{{i}\pi} \right)^{{x}} ={x} \\ $$$${e}^{{x}\left(\mathrm{ln}\:\mathrm{4}+{i}\pi\right)} ={x} \\ $$$${xe}^{−{x}\left(\mathrm{ln}\:\mathrm{4}+{i}\pi\right)} =\mathrm{1} \\ $$$$−{x}\left(\mathrm{ln}\:\mathrm{4}+{i}\pi\right){e}^{−{x}\left(\mathrm{ln}\:\mathrm{4}+{i}\pi\right)} =−\left(\mathrm{ln}\:\mathrm{4}+{i}\pi\right) \\ $$$$−{x}\left(\mathrm{ln}\:\mathrm{4}+{i}\pi\right)={W}\left(−\mathrm{ln}\:\mathrm{4}−{i}\pi\right)\:\:\left({Lambert}\:{W}\:{function}\right) \\ $$$$\Rightarrow{x}=−\frac{{W}\left(−\mathrm{ln}\:\mathrm{4}−{i}\pi\right)}{\mathrm{ln}\:\mathrm{4}+{i}\pi} \\ $$
