Menu Close

Question-75110

Tinku Tara 0 Comments
Pin




Question Number 75110 by peter frank last updated on 07/Dec/19
Answered by mind is power last updated on 08/Dec/19
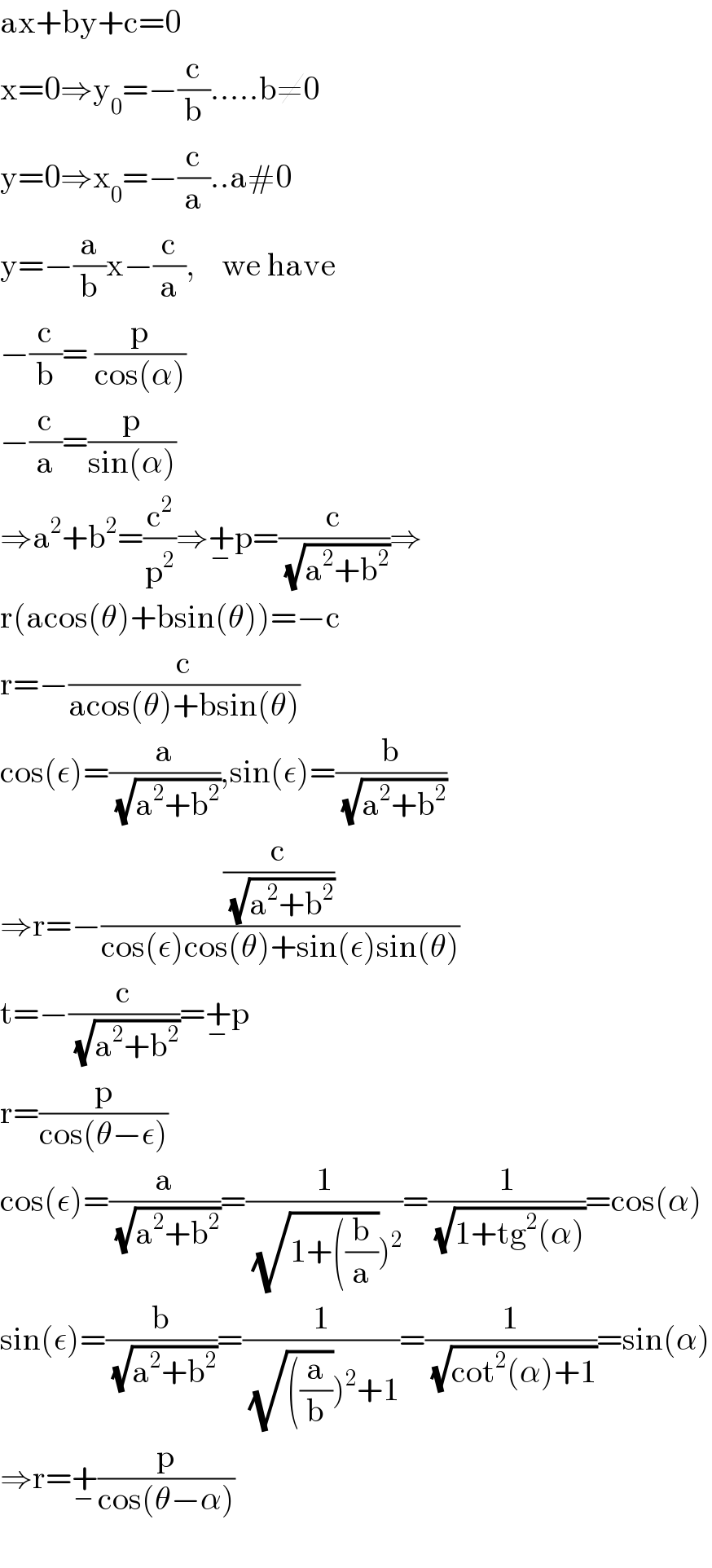
ax+by+c=0 x=0⇒y_0 =−(c/b).....b≠0 y=0⇒x_0 =−(c/a)..a#0 y=−(a/b)x−(c/a), we have −(c/b)= (p/(cos(α))) −(c/a)=(p/(sin(α))) ⇒a^2 +b^2 =(c^2 /p^2 )⇒+_− p=(c/( (√(a^2 +b^2 ))))⇒ r(acos(θ)+bsin(θ))=−c r=−(c/(acos(θ)+bsin(θ))) cos(ε)=(a/( (√(a^2 +b^2 )))),sin(ε)=(b/( (√(a^2 +b^2 )))) ⇒r=−((c/( (√(a^2 +b^2 ))))/(cos(ε)cos(θ)+sin(ε)sin(θ))) t=−(c/( (√(a^2 +b^2 ))))=+_− p r=(p/(cos(θ−ε))) cos(ε)=(a/( (√(a^2 +b^2 ))))=(1/( (√(1+((b/a))))^2 ))=(1/( (√(1+tg^2 (α)))))=cos(α) sin(ε)=(b/( (√(a^2 +b^2 ))))=(1/( (√(((a/b))))^2 +1))=(1/( (√(cot^2 (α)+1))))=sin(α) ⇒r=+_− (p/(cos(θ−α)))
$$\mathrm{ax}+\mathrm{by}+\mathrm{c}=\mathrm{0} \\ $$$$\mathrm{x}=\mathrm{0}\Rightarrow\mathrm{y}_{\mathrm{0}} =−\frac{\mathrm{c}}{\mathrm{b}}…..\mathrm{b}\neq\mathrm{0} \\ $$$$\mathrm{y}=\mathrm{0}\Rightarrow\mathrm{x}_{\mathrm{0}} =−\frac{\mathrm{c}}{\mathrm{a}}..\mathrm{a}#\mathrm{0} \\ $$$$\mathrm{y}=−\frac{\mathrm{a}}{\mathrm{b}}\mathrm{x}−\frac{\mathrm{c}}{\mathrm{a}},\:\:\:\:\mathrm{we}\:\mathrm{have}\: \\ $$$$−\frac{\mathrm{c}}{\mathrm{b}}=\:\frac{\mathrm{p}}{\mathrm{cos}\left(\alpha\right)} \\ $$$$−\frac{\mathrm{c}}{\mathrm{a}}=\frac{\mathrm{p}}{\mathrm{sin}\left(\alpha\right)} \\ $$$$\Rightarrow\mathrm{a}^{\mathrm{2}} +\mathrm{b}^{\mathrm{2}} =\frac{\mathrm{c}^{\mathrm{2}} }{\mathrm{p}^{\mathrm{2}} }\Rightarrow\underset{−} {+}\mathrm{p}=\frac{\mathrm{c}}{\:\sqrt{\mathrm{a}^{\mathrm{2}} +\mathrm{b}^{\mathrm{2}} }}\Rightarrow \\ $$$$\mathrm{r}\left(\mathrm{acos}\left(\theta\right)+\mathrm{bsin}\left(\theta\right)\right)=−\mathrm{c} \\ $$$$\mathrm{r}=−\frac{\mathrm{c}}{\mathrm{acos}\left(\theta\right)+\mathrm{bsin}\left(\theta\right)} \\ $$$$\mathrm{cos}\left(\epsilon\right)=\frac{\mathrm{a}}{\:\sqrt{\mathrm{a}^{\mathrm{2}} +\mathrm{b}^{\mathrm{2}} }},\mathrm{sin}\left(\epsilon\right)=\frac{\mathrm{b}}{\:\sqrt{\mathrm{a}^{\mathrm{2}} +\mathrm{b}^{\mathrm{2}} }} \\ $$$$\Rightarrow\mathrm{r}=−\frac{\frac{\mathrm{c}}{\:\sqrt{\mathrm{a}^{\mathrm{2}} +\mathrm{b}^{\mathrm{2}} }}}{\mathrm{cos}\left(\epsilon\right)\mathrm{cos}\left(\theta\right)+\mathrm{sin}\left(\epsilon\right)\mathrm{sin}\left(\theta\right)} \\ $$$$\mathrm{t}=−\frac{\mathrm{c}}{\:\sqrt{\mathrm{a}^{\mathrm{2}} +\mathrm{b}^{\mathrm{2}} }}=\underset{−} {+}\mathrm{p} \\ $$$$\mathrm{r}=\frac{\mathrm{p}}{\mathrm{cos}\left(\theta−\epsilon\right)} \\ $$$$\mathrm{cos}\left(\epsilon\right)=\frac{\mathrm{a}}{\:\sqrt{\mathrm{a}^{\mathrm{2}} +\mathrm{b}^{\mathrm{2}} }}=\frac{\mathrm{1}}{\left.\:\sqrt{\mathrm{1}+\left(\frac{\mathrm{b}}{\mathrm{a}}\right.}\right)^{\mathrm{2}} }=\frac{\mathrm{1}}{\:\sqrt{\mathrm{1}+\mathrm{tg}^{\mathrm{2}} \left(\alpha\right)}}=\mathrm{cos}\left(\alpha\right) \\ $$$$\mathrm{sin}\left(\epsilon\right)=\frac{\mathrm{b}}{\:\sqrt{\mathrm{a}^{\mathrm{2}} +\mathrm{b}^{\mathrm{2}} }}=\frac{\mathrm{1}}{\left.\:\sqrt{\left(\frac{\mathrm{a}}{\mathrm{b}}\right.}\right)^{\mathrm{2}} +\mathrm{1}}=\frac{\mathrm{1}}{\:\sqrt{\mathrm{cot}^{\mathrm{2}} \left(\alpha\right)+\mathrm{1}}}=\mathrm{sin}\left(\alpha\right) \\ $$$$\Rightarrow\mathrm{r}=\underset{−} {+}\frac{\mathrm{p}}{\mathrm{cos}\left(\theta−\alpha\right)} \\ $$$$ \\ $$
Commented by peter frank last updated on 09/Dec/19
thank you very much
$${thank}\:{you}\:{very}\:{much} \\ $$
Commented by peter frank last updated on 09/Dec/19
please sir can i get the diagram
$${please}\:{sir}\:{can}\:{i}\:{get}\:{the} \\ $$$${diagram} \\ $$

Pin

Leave a Reply

Your email address will not be published. Required fields are marked *