Menu Close

Question-75457

Tinku Tara 0 Comments
Pin




Question Number 75457 by Master last updated on 11/Dec/19
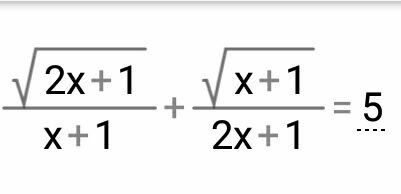
Commented by MJS last updated on 11/Dec/19
no exact solution possible try to approximate, there are 3 solutions, one real and two conjugated complex
$$\mathrm{no}\:\mathrm{exact}\:\mathrm{solution}\:\mathrm{possible} \\ $$$$\mathrm{try}\:\mathrm{to}\:\mathrm{approximate},\:\mathrm{there}\:\mathrm{are}\:\mathrm{3}\:\mathrm{solutions}, \\ $$$$\mathrm{one}\:\mathrm{real}\:\mathrm{and}\:\mathrm{two}\:\mathrm{conjugated}\:\mathrm{complex} \\ $$
Commented by behi83417@gmail.com last updated on 12/Dec/19
2x+1=t^2 ,x+1=s^2 ⇒x=s^2 −1,t^2 =2s^2 −1 ⇒(t/s^2 )+(s/t^2 )=5⇒t^3 +s^3 =5t^2 s^2 ⇒t^3 =s^2 (5t^2 −s)⇒t^6 =s^4 (25t^4 −10st^2 +s^2 ) ⇒(2s^2 −1)^3 =s^4 (25(2s^2 −1)^2 −10s(2s^2 −1)+s^2 ) ⇒8s^6 −12s^4 +6s^2 −1= =s^4 [100s^4 −100s^2 +25−20s^3 +10s+s^2 )= =100s^8 −20s^7 −99s^6 +10s^5 +25s^4 ⇒100s^8 −20s^7 −107s^6 +10s^5 +37s^4 −6s^2 +1=0 ⇒s=−0.661 ,0.753 ⇒[x=−0.563 ,−0.433]
$$\mathrm{2x}+\mathrm{1}=\mathrm{t}^{\mathrm{2}} ,\mathrm{x}+\mathrm{1}=\mathrm{s}^{\mathrm{2}} \Rightarrow\mathrm{x}=\mathrm{s}^{\mathrm{2}} −\mathrm{1},\mathrm{t}^{\mathrm{2}} =\mathrm{2s}^{\mathrm{2}} −\mathrm{1} \\ $$$$\Rightarrow\frac{\mathrm{t}}{\mathrm{s}^{\mathrm{2}} }+\frac{\mathrm{s}}{\mathrm{t}^{\mathrm{2}} }=\mathrm{5}\Rightarrow\mathrm{t}^{\mathrm{3}} +\mathrm{s}^{\mathrm{3}} =\mathrm{5t}^{\mathrm{2}} \mathrm{s}^{\mathrm{2}} \\ $$$$\Rightarrow\mathrm{t}^{\mathrm{3}} =\mathrm{s}^{\mathrm{2}} \left(\mathrm{5t}^{\mathrm{2}} −\mathrm{s}\right)\Rightarrow\mathrm{t}^{\mathrm{6}} =\mathrm{s}^{\mathrm{4}} \left(\mathrm{25t}^{\mathrm{4}} −\mathrm{10st}^{\mathrm{2}} +\mathrm{s}^{\mathrm{2}} \right) \\ $$$$\Rightarrow\left(\mathrm{2s}^{\mathrm{2}} −\mathrm{1}\right)^{\mathrm{3}} =\mathrm{s}^{\mathrm{4}} \left(\mathrm{25}\left(\mathrm{2s}^{\mathrm{2}} −\mathrm{1}\right)^{\mathrm{2}} −\mathrm{10s}\left(\mathrm{2s}^{\mathrm{2}} −\mathrm{1}\right)+\mathrm{s}^{\mathrm{2}} \right) \\ $$$$\Rightarrow\mathrm{8s}^{\mathrm{6}} −\mathrm{12s}^{\mathrm{4}} +\mathrm{6s}^{\mathrm{2}} −\mathrm{1}= \\ $$$$=\mathrm{s}^{\mathrm{4}} \left[\mathrm{100s}^{\mathrm{4}} −\mathrm{100s}^{\mathrm{2}} +\mathrm{25}−\mathrm{20s}^{\mathrm{3}} +\mathrm{10s}+\mathrm{s}^{\mathrm{2}} \right)= \\ $$$$=\mathrm{100s}^{\mathrm{8}} −\mathrm{20s}^{\mathrm{7}} −\mathrm{99s}^{\mathrm{6}} +\mathrm{10s}^{\mathrm{5}} +\mathrm{25s}^{\mathrm{4}} \\ $$$$\Rightarrow\mathrm{100s}^{\mathrm{8}} −\mathrm{20s}^{\mathrm{7}} −\mathrm{107s}^{\mathrm{6}} +\mathrm{10s}^{\mathrm{5}} +\mathrm{37s}^{\mathrm{4}} −\mathrm{6s}^{\mathrm{2}} +\mathrm{1}=\mathrm{0} \\ $$$$\Rightarrow\boldsymbol{\mathrm{s}}=−\mathrm{0}.\mathrm{661}\:\:,\mathrm{0}.\mathrm{753} \\ $$$$\Rightarrow\left[\mathrm{x}=−\mathrm{0}.\mathrm{563}\:\:,−\mathrm{0}.\mathrm{433}\right] \\ $$
Commented by MJS last updated on 12/Dec/19
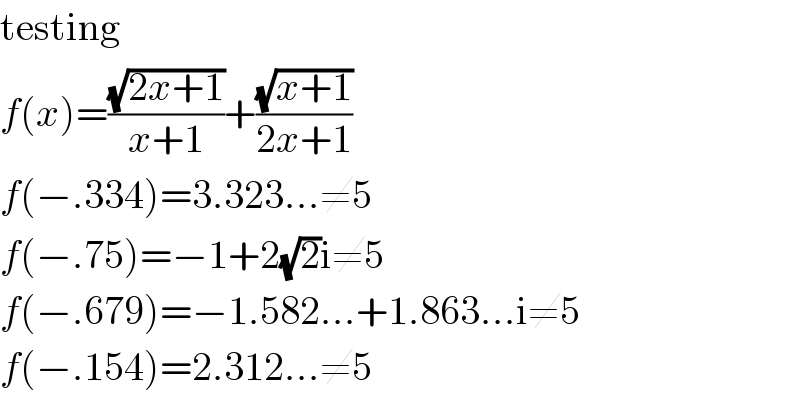
testing f(x)=((√(2x+1))/(x+1))+((√(x+1))/(2x+1)) f(−.334)=3.323...≠5 f(−.75)=−1+2(√2)i≠5 f(−.679)=−1.582...+1.863...i≠5 f(−.154)=2.312...≠5
$$\mathrm{testing} \\ $$$${f}\left({x}\right)=\frac{\sqrt{\mathrm{2}{x}+\mathrm{1}}}{{x}+\mathrm{1}}+\frac{\sqrt{{x}+\mathrm{1}}}{\mathrm{2}{x}+\mathrm{1}} \\ $$$${f}\left(−.\mathrm{334}\right)=\mathrm{3}.\mathrm{323}…\neq\mathrm{5} \\ $$$${f}\left(−.\mathrm{75}\right)=−\mathrm{1}+\mathrm{2}\sqrt{\mathrm{2}}\mathrm{i}\neq\mathrm{5} \\ $$$${f}\left(−.\mathrm{679}\right)=−\mathrm{1}.\mathrm{582}…+\mathrm{1}.\mathrm{863}…\mathrm{i}\neq\mathrm{5} \\ $$$${f}\left(−.\mathrm{154}\right)=\mathrm{2}.\mathrm{312}…\neq\mathrm{5} \\ $$
Commented by MJS last updated on 12/Dec/19
error in line 3 from end it must be 37s^4 instead of 31s^4 ⇒ you get the same solutions as me
$$\mathrm{error}\:\mathrm{in}\:\mathrm{line}\:\mathrm{3}\:\mathrm{from}\:\mathrm{end} \\ $$$$\mathrm{it}\:\mathrm{must}\:\mathrm{be}\:\mathrm{37}{s}^{\mathrm{4}} \:\mathrm{instead}\:\mathrm{of}\:\mathrm{31}{s}^{\mathrm{4}} \\ $$$$\Rightarrow \\ $$$$\mathrm{you}\:\mathrm{get}\:\mathrm{the}\:\mathrm{same}\:\mathrm{solutions}\:\mathrm{as}\:\mathrm{me} \\ $$
Commented by MJS last updated on 12/Dec/19
100s^8 −20s^7 −107s^6 +10s^5 +37s^4 −6s^2 +1=0 real solutions are s_1 ≈.752784 ⇒ x_1 ≈−.433316 s_2 ≈.767899 ⇒ x_2 ≈−.410330 but the first one doesn′t solve the given equation because of squaring along the path
$$\mathrm{100}{s}^{\mathrm{8}} −\mathrm{20}{s}^{\mathrm{7}} −\mathrm{107}{s}^{\mathrm{6}} +\mathrm{10}{s}^{\mathrm{5}} +\mathrm{37}{s}^{\mathrm{4}} −\mathrm{6}{s}^{\mathrm{2}} +\mathrm{1}=\mathrm{0} \\ $$$$\mathrm{real}\:\mathrm{solutions}\:\mathrm{are} \\ $$$${s}_{\mathrm{1}} \approx.\mathrm{752784}\:\Rightarrow\:{x}_{\mathrm{1}} \approx−.\mathrm{433316} \\ $$$${s}_{\mathrm{2}} \approx.\mathrm{767899}\:\Rightarrow\:{x}_{\mathrm{2}} \approx−.\mathrm{410330} \\ $$$$\mathrm{but}\:\mathrm{the}\:\mathrm{first}\:\mathrm{one}\:\mathrm{doesn}'\mathrm{t}\:\mathrm{solve}\:\mathrm{the}\:\mathrm{given}\:\mathrm{equation} \\ $$$$\mathrm{because}\:\mathrm{of}\:\mathrm{squaring}\:\mathrm{along}\:\mathrm{the}\:\mathrm{path} \\ $$
Answered by peter frank last updated on 11/Dec/19
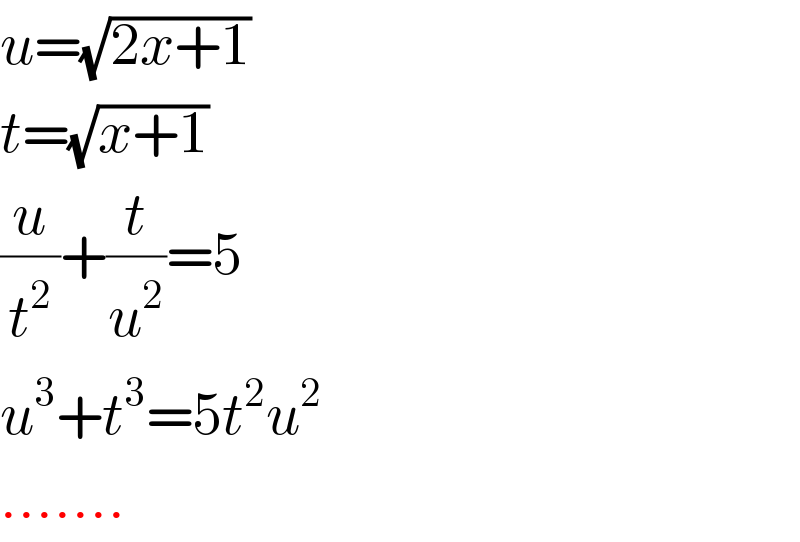
u=(√(2x+1)) t=(√(x+1)) (u/t^2 )+(t/u^2 )=5 u^3 +t^3 =5t^2 u^2 .......
$${u}=\sqrt{\mathrm{2}{x}+\mathrm{1}} \\ $$$${t}=\sqrt{{x}+\mathrm{1}} \\ $$$$\frac{{u}}{{t}^{\mathrm{2}} }+\frac{{t}}{{u}^{\mathrm{2}} }=\mathrm{5} \\ $$$${u}^{\mathrm{3}} +{t}^{\mathrm{3}} =\mathrm{5}{t}^{\mathrm{2}} {u}^{\mathrm{2}} \\ $$$$……. \\ $$
Answered by MJS last updated on 12/Dec/19
squaring, transforming, squaring again leads to x^8 +((291)/(50))x^7 +((146649)/(10000))x^6 +((52119)/(2500))x^5 +((91249)/(5000))x^4 +((50277)/(5000))x^3 +((34009)/(10000))x^2 +((129)/(200))x+((21)/(400))=0 but we have to test all solutions because squaring leads to false solutions the approximated roots are: x_1 ≈−.433316 wrong x_2 ≈−.410330 right x_(3, 4) ≈−.973872±.209039i wrong x_(5, 6) ≈−.950789±.181535i right x_(7, 8) ≈−.563516±.00951046i wrong ⇒ the solutions are x≈−.410330∨x≈−.950789±.181535i
$$\mathrm{squaring},\:\mathrm{transforming},\:\mathrm{squaring}\:\mathrm{again}\:\mathrm{leads}\:\mathrm{to} \\ $$$${x}^{\mathrm{8}} +\frac{\mathrm{291}}{\mathrm{50}}{x}^{\mathrm{7}} +\frac{\mathrm{146649}}{\mathrm{10000}}{x}^{\mathrm{6}} +\frac{\mathrm{52119}}{\mathrm{2500}}{x}^{\mathrm{5}} +\frac{\mathrm{91249}}{\mathrm{5000}}{x}^{\mathrm{4}} +\frac{\mathrm{50277}}{\mathrm{5000}}{x}^{\mathrm{3}} +\frac{\mathrm{34009}}{\mathrm{10000}}{x}^{\mathrm{2}} +\frac{\mathrm{129}}{\mathrm{200}}{x}+\frac{\mathrm{21}}{\mathrm{400}}=\mathrm{0} \\ $$$$\mathrm{but}\:\mathrm{we}\:\mathrm{have}\:\mathrm{to}\:\mathrm{test}\:\mathrm{all}\:\mathrm{solutions}\:\mathrm{because} \\ $$$$\mathrm{squaring}\:\mathrm{leads}\:\mathrm{to}\:\mathrm{false}\:\mathrm{solutions} \\ $$$$\mathrm{the}\:\mathrm{approximated}\:\mathrm{roots}\:\mathrm{are}: \\ $$$${x}_{\mathrm{1}} \approx−.\mathrm{433316}\:\mathrm{wrong} \\ $$$${x}_{\mathrm{2}} \approx−.\mathrm{410330}\:\mathrm{right} \\ $$$${x}_{\mathrm{3},\:\mathrm{4}} \approx−.\mathrm{973872}\pm.\mathrm{209039i}\:\mathrm{wrong} \\ $$$${x}_{\mathrm{5},\:\mathrm{6}} \approx−.\mathrm{950789}\pm.\mathrm{181535i}\:\mathrm{right} \\ $$$${x}_{\mathrm{7},\:\mathrm{8}} \approx−.\mathrm{563516}\pm.\mathrm{00951046i}\:\mathrm{wrong} \\ $$$$\Rightarrow \\ $$$$\mathrm{the}\:\mathrm{solutions}\:\mathrm{are} \\ $$$${x}\approx−.\mathrm{410330}\vee{x}\approx−.\mathrm{950789}\pm.\mathrm{181535i} \\ $$
Commented by behi83417@gmail.com last updated on 12/Dec/19
thank you very much sir proph: MJS. I fixed the error,but answers not much with yours.
$$\mathrm{thank}\:\mathrm{you}\:\mathrm{very}\:\mathrm{much}\:\mathrm{sir}\:\mathrm{proph}:\:\mathrm{MJS}. \\ $$$$\mathrm{I}\:\mathrm{fixed}\:\mathrm{the}\:\mathrm{error},\mathrm{but}\:\mathrm{answers}\:\mathrm{not}\:\mathrm{much} \\ $$$$\mathrm{with}\:\mathrm{yours}. \\ $$

Pin

Leave a Reply

Your email address will not be published. Required fields are marked *