Question Number 75597 by aliesam last updated on 13/Dec/19
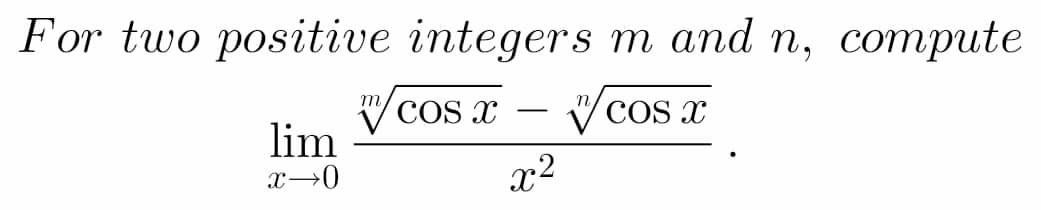
Commented by mathmax by abdo last updated on 13/Dec/19
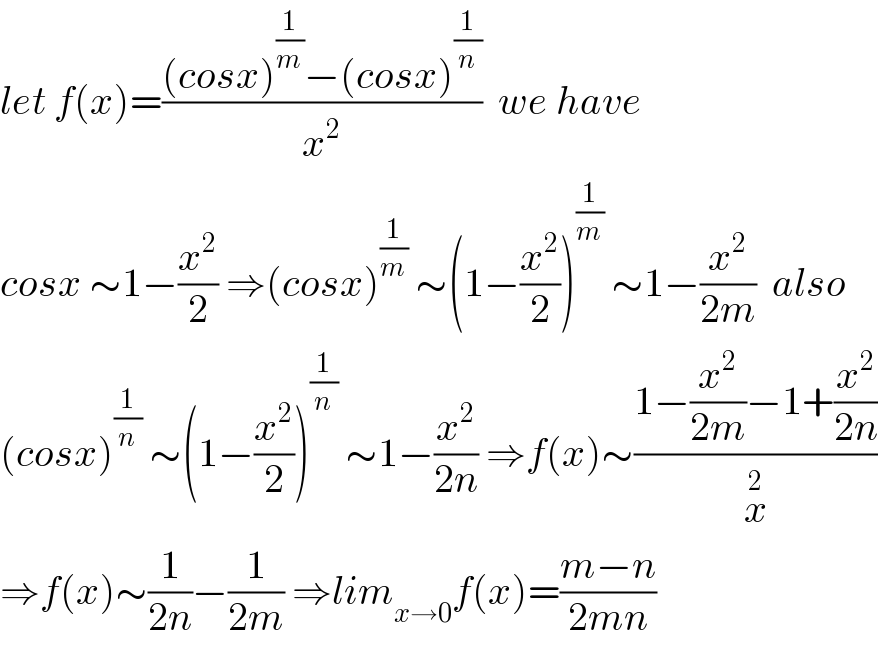
$${let}\:{f}\left({x}\right)=\frac{\left({cosx}\right)^{\frac{\mathrm{1}}{{m}}} −\left({cosx}\right)^{\frac{\mathrm{1}}{{n}}} }{{x}^{\mathrm{2}} }\:\:{we}\:{have} \\ $$$${cosx}\:\sim\mathrm{1}−\frac{{x}^{\mathrm{2}} }{\mathrm{2}}\:\Rightarrow\left({cosx}\right)^{\frac{\mathrm{1}}{{m}}} \:\sim\left(\mathrm{1}−\frac{{x}^{\mathrm{2}} }{\mathrm{2}}\right)^{\frac{\mathrm{1}}{{m}}} \:\sim\mathrm{1}−\frac{{x}^{\mathrm{2}} }{\mathrm{2}{m}}\:\:{also} \\ $$$$\left({cosx}\right)^{\frac{\mathrm{1}}{{n}}} \:\sim\left(\mathrm{1}−\frac{{x}^{\mathrm{2}} }{\mathrm{2}}\right)^{\frac{\mathrm{1}}{{n}}} \:\sim\mathrm{1}−\frac{{x}^{\mathrm{2}} }{\mathrm{2}{n}}\:\Rightarrow{f}\left({x}\right)\sim\frac{\mathrm{1}−\frac{{x}^{\mathrm{2}} }{\mathrm{2}{m}}−\mathrm{1}+\frac{{x}^{\mathrm{2}} }{\mathrm{2}{n}}}{\overset{\mathrm{2}} {{x}}} \\ $$$$\Rightarrow{f}\left({x}\right)\sim\frac{\mathrm{1}}{\mathrm{2}{n}}−\frac{\mathrm{1}}{\mathrm{2}{m}}\:\Rightarrow{lim}_{{x}\rightarrow\mathrm{0}} {f}\left({x}\right)=\frac{{m}−{n}}{\mathrm{2}{mn}} \\ $$
