Question-75684

Question Number 75684 by mr W last updated on 15/Dec/19

Commented by mr W last updated on 15/Dec/19

if the side length of square is 1. find the radius of the small green circle.

$${if}\:{the}\:{side}\:{length}\:{of}\:{square}\:{is}\:\mathrm{1}. \\ $$$${find}\:{the}\:{radius}\:{of}\:{the}\:{small}\:{green} \\ $$$${circle}. \\ $$

Commented by vishalbhardwaj last updated on 15/Dec/19

$$\mathrm{sir}\:\mathrm{please}\:\mathrm{give}\:\mathrm{some}\:\mathrm{hint}\:\mathrm{to}\:\mathrm{solve} \\ $$

Answered by MJS last updated on 15/Dec/19

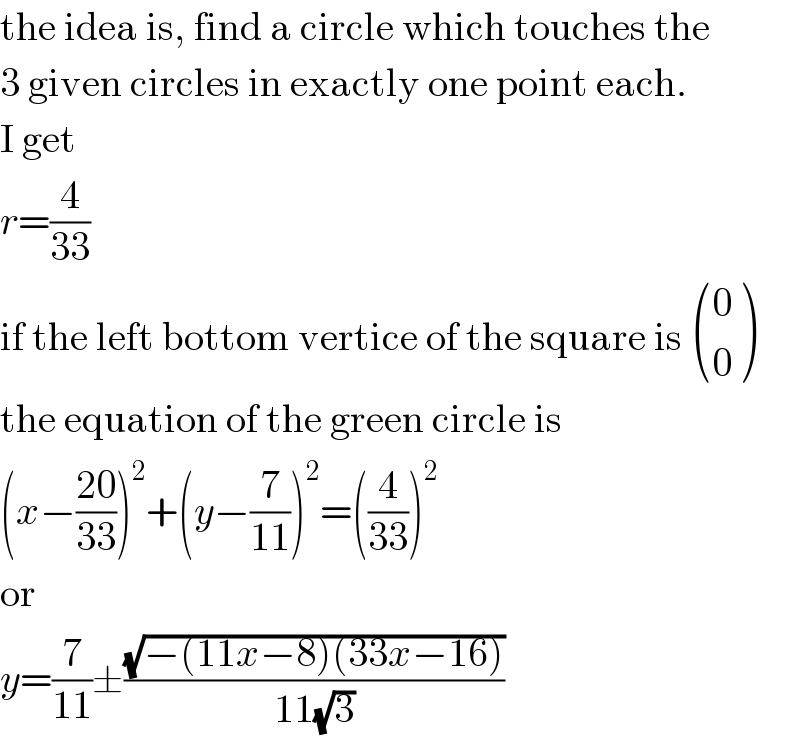
the idea is, find a circle which touches the 3 given circles in exactly one point each. I get r=(4/(33)) if the left bottom vertice of the square is ((0),(0) ) the equation of the green circle is (x−((20)/(33)))^2 +(y−(7/(11)))^2 =((4/(33)))^2 or y=(7/(11))±((√(−(11x−8)(33x−16)))/(11(√3)))

$$\mathrm{the}\:\mathrm{idea}\:\mathrm{is},\:\mathrm{find}\:\mathrm{a}\:\mathrm{circle}\:\mathrm{which}\:\mathrm{touches}\:\mathrm{the} \\ $$$$\mathrm{3}\:\mathrm{given}\:\mathrm{circles}\:\mathrm{in}\:\mathrm{exactly}\:\mathrm{one}\:\mathrm{point}\:\mathrm{each}. \\ $$$$\mathrm{I}\:\mathrm{get} \\ $$$${r}=\frac{\mathrm{4}}{\mathrm{33}} \\ $$$$\mathrm{if}\:\mathrm{the}\:\mathrm{left}\:\mathrm{bottom}\:\mathrm{vertice}\:\mathrm{of}\:\mathrm{the}\:\mathrm{square}\:\mathrm{is}\:\begin{pmatrix}{\mathrm{0}}\\{\mathrm{0}}\end{pmatrix} \\ $$$$\mathrm{the}\:\mathrm{equation}\:\mathrm{of}\:\mathrm{the}\:\mathrm{green}\:\mathrm{circle}\:\mathrm{is} \\ $$$$\left({x}−\frac{\mathrm{20}}{\mathrm{33}}\right)^{\mathrm{2}} +\left({y}−\frac{\mathrm{7}}{\mathrm{11}}\right)^{\mathrm{2}} =\left(\frac{\mathrm{4}}{\mathrm{33}}\right)^{\mathrm{2}} \\ $$$$\mathrm{or} \\ $$$${y}=\frac{\mathrm{7}}{\mathrm{11}}\pm\frac{\sqrt{−\left(\mathrm{11}{x}−\mathrm{8}\right)\left(\mathrm{33}{x}−\mathrm{16}\right)}}{\mathrm{11}\sqrt{\mathrm{3}}} \\ $$

Commented by mr W last updated on 15/Dec/19

$${thanks}\:{sir}!\:{i}'{ll}\:{try}\:{to}\:{see}\:{if}\:{i}\:{could} \\ $$$${get}\:{the}\:{same}\:{result}. \\ $$

Answered by mr W last updated on 15/Dec/19

Commented by mr W last updated on 15/Dec/19

A(0,0) C(x,y) x^2 +y^2 =(1−r)^2 ...(i) x^2 +(y−(1/2))^2 =((1/2)+r)^2 ...(ii) (x−(1/2))^2 +(1−y)^2 =((1/2)−r)^2 ...(iii) (i)−(ii): (1/2)(2y−(1/2))=(3/2)((1/2)−2r) ⇒y=1−3r (i)−(iii): (1/2)(2x−(1/2))+(2y−1)=(1/2)((3/2)−2r) x+2y=2−r x+2(1−3r)=2−r ⇒x=5r (5r)^2 +(1−3r)^2 =(1−r)^2 33r^2 =4r ⇒r=(4/(33)) ⇒y=1−((3×4)/(33))=(7/(11)) ⇒x=5×(4/(33))=((20)/(33)) ⇒C(((20)/(33)),(7/(11)))

$${A}\left(\mathrm{0},\mathrm{0}\right) \\ $$$${C}\left({x},{y}\right) \\ $$$${x}^{\mathrm{2}} +{y}^{\mathrm{2}} =\left(\mathrm{1}−{r}\right)^{\mathrm{2}} \:\:\:…\left({i}\right) \\ $$$${x}^{\mathrm{2}} +\left({y}−\frac{\mathrm{1}}{\mathrm{2}}\right)^{\mathrm{2}} =\left(\frac{\mathrm{1}}{\mathrm{2}}+{r}\right)^{\mathrm{2}} \:\:\:…\left({ii}\right) \\ $$$$\left({x}−\frac{\mathrm{1}}{\mathrm{2}}\right)^{\mathrm{2}} +\left(\mathrm{1}−{y}\right)^{\mathrm{2}} =\left(\frac{\mathrm{1}}{\mathrm{2}}−{r}\right)^{\mathrm{2}} \:\:\:…\left({iii}\right) \\ $$$$\left({i}\right)−\left({ii}\right): \\ $$$$\frac{\mathrm{1}}{\mathrm{2}}\left(\mathrm{2}{y}−\frac{\mathrm{1}}{\mathrm{2}}\right)=\frac{\mathrm{3}}{\mathrm{2}}\left(\frac{\mathrm{1}}{\mathrm{2}}−\mathrm{2}{r}\right) \\ $$$$\Rightarrow{y}=\mathrm{1}−\mathrm{3}{r} \\ $$$$\left({i}\right)−\left({iii}\right): \\ $$$$\frac{\mathrm{1}}{\mathrm{2}}\left(\mathrm{2}{x}−\frac{\mathrm{1}}{\mathrm{2}}\right)+\left(\mathrm{2}{y}−\mathrm{1}\right)=\frac{\mathrm{1}}{\mathrm{2}}\left(\frac{\mathrm{3}}{\mathrm{2}}−\mathrm{2}{r}\right) \\ $$$${x}+\mathrm{2}{y}=\mathrm{2}−{r} \\ $$$${x}+\mathrm{2}\left(\mathrm{1}−\mathrm{3}{r}\right)=\mathrm{2}−{r} \\ $$$$\Rightarrow{x}=\mathrm{5}{r} \\ $$$$\left(\mathrm{5}{r}\right)^{\mathrm{2}} +\left(\mathrm{1}−\mathrm{3}{r}\right)^{\mathrm{2}} =\left(\mathrm{1}−{r}\right)^{\mathrm{2}} \\ $$$$\mathrm{33}{r}^{\mathrm{2}} =\mathrm{4}{r} \\ $$$$\Rightarrow{r}=\frac{\mathrm{4}}{\mathrm{33}} \\ $$$$\Rightarrow{y}=\mathrm{1}−\frac{\mathrm{3}×\mathrm{4}}{\mathrm{33}}=\frac{\mathrm{7}}{\mathrm{11}} \\ $$$$\Rightarrow{x}=\mathrm{5}×\frac{\mathrm{4}}{\mathrm{33}}=\frac{\mathrm{20}}{\mathrm{33}} \\ $$$$\Rightarrow{C}\left(\frac{\mathrm{20}}{\mathrm{33}},\frac{\mathrm{7}}{\mathrm{11}}\right) \\ $$

Facebook Tweet Pin

Leave a Reply Cancel reply