Question Number 75809 by aliesam last updated on 17/Dec/19

Commented by kaivan.ahmadi last updated on 19/Dec/19
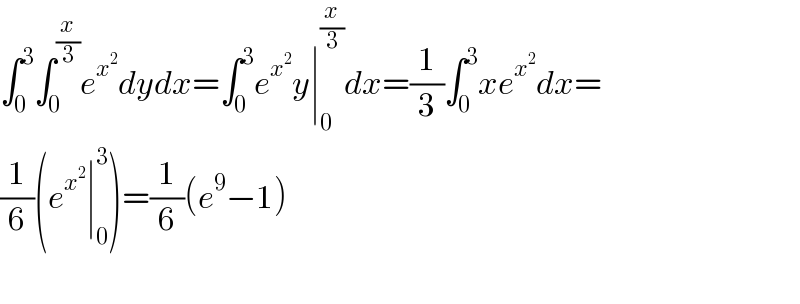
$$\int_{\mathrm{0}} ^{\mathrm{3}} \int_{\mathrm{0}} ^{\frac{{x}}{\mathrm{3}}} {e}^{{x}^{\mathrm{2}} } {dydx}=\int_{\mathrm{0}} ^{\mathrm{3}} {e}^{{x}^{\mathrm{2}} } {y}\mid_{\mathrm{0}} ^{\frac{{x}}{\mathrm{3}}} {dx}=\frac{\mathrm{1}}{\mathrm{3}}\int_{\mathrm{0}} ^{\mathrm{3}} {xe}^{{x}^{\mathrm{2}} } {dx}= \\ $$$$\frac{\mathrm{1}}{\mathrm{6}}\left({e}^{{x}^{\mathrm{2}} } \mid_{\mathrm{0}} ^{\mathrm{3}} \right)=\frac{\mathrm{1}}{\mathrm{6}}\left({e}^{\mathrm{9}} −\mathrm{1}\right) \\ $$$$ \\ $$
Commented by kaivan.ahmadi last updated on 19/Dec/19

