Menu Close

Question-76586

Tinku Tara 0 Comments
Pin




Question Number 76586 by Master last updated on 28/Dec/19
Commented by Master last updated on 28/Dec/19
solve please
$$\boldsymbol{\mathrm{solve}}\:\boldsymbol{\mathrm{please}} \\ $$
Commented by Tony Lin last updated on 28/Dec/19
(5) ∫_0 ^(π/2) sin^n xcos^n xdx =(1/2^n )∫_0 ^(π/2) sin^n (2x)dx let 2x=u, du=2dx (1/2^(n−1) )∫_0 ^π sin^n udu =(1/2^(n−2) )∫_0 ^(π/2) sin^n xdx =(1/2^(n−2) )× { (((((n−1)(n−3)∙∙∙1)/(n(n−2)∙∙∙2))×(π/2), if n is even)),(((((n−1)(n−3)∙∙∙2)/(n(n−2)∙∙∙1)), if n is odd)) :}
$$\left(\mathrm{5}\right) \\ $$$$\int_{\mathrm{0}} ^{\frac{\pi}{\mathrm{2}}} {sin}^{{n}} {xcos}^{{n}} {xdx} \\ $$$$=\frac{\mathrm{1}}{\mathrm{2}^{{n}} }\int_{\mathrm{0}} ^{\frac{\pi}{\mathrm{2}}} {sin}^{{n}} \left(\mathrm{2}{x}\right){dx} \\ $$$${let}\:\mathrm{2}{x}={u},\:{du}=\mathrm{2}{dx} \\ $$$$\frac{\mathrm{1}}{\mathrm{2}^{{n}−\mathrm{1}} }\int_{\mathrm{0}} ^{\pi} {sin}^{{n}} {udu} \\ $$$$=\frac{\mathrm{1}}{\mathrm{2}^{{n}−\mathrm{2}} }\int_{\mathrm{0}} ^{\frac{\pi}{\mathrm{2}}} {sin}^{{n}} {xdx} \\ $$$$=\frac{\mathrm{1}}{\mathrm{2}^{{n}−\mathrm{2}} }×\begin{cases}{\frac{\left({n}−\mathrm{1}\right)\left({n}−\mathrm{3}\right)\centerdot\centerdot\centerdot\mathrm{1}}{{n}\left({n}−\mathrm{2}\right)\centerdot\centerdot\centerdot\mathrm{2}}×\frac{\pi}{\mathrm{2}},\:{if}\:{n}\:{is}\:{even}}\\{\frac{\left({n}−\mathrm{1}\right)\left({n}−\mathrm{3}\right)\centerdot\centerdot\centerdot\mathrm{2}}{{n}\left({n}−\mathrm{2}\right)\centerdot\centerdot\centerdot\mathrm{1}},\:{if}\:{n}\:{is}\:{odd}}\end{cases} \\ $$
Commented by john santu last updated on 28/Dec/19
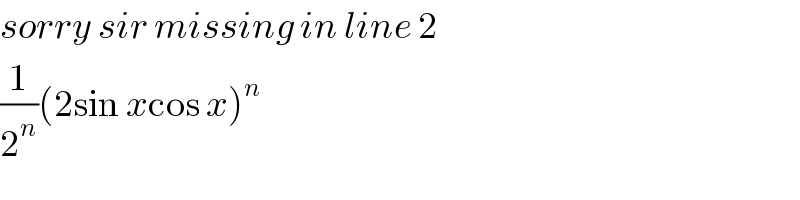
sorry sir missing in line 2 (1/2^n )(2sin xcos x)^n
$${sorry}\:{sir}\:{missing}\:{in}\:{line}\:\mathrm{2} \\ $$$$\frac{\mathrm{1}}{\mathrm{2}^{{n}} }\left(\mathrm{2sin}\:{x}\mathrm{cos}\:{x}\right)^{{n}} \\ $$
Commented by Tony Lin last updated on 28/Dec/19
(1) a_(n+2) =(1/a_(n+1) )+a_n ⇒a_(n+2) a_(n+1) =a_(n+1) a_n +1 ⇒a_(2019) a_(2018) =a_(2018) a_(2017) +1 =a_(2017) a_(2016) +2 =a_1 a_2 +2017 =2018 ⇒a_(n+1) a_n =n (a_(2019) /a_(2017) )=((2018)/(2017)) (a_(2017) /a_(2015) )=((2016)/(2015)) (a_5 /a_3 )=(4/3) (a_3 /a_1 )=(2/1) ⇒a_(2019) =a_1 ×((2×4×∙∙∙×2018)/(1×3×∙∙∙×2017)) =((2018!!)/(2017!!))
$$\left(\mathrm{1}\right) \\ $$$${a}_{{n}+\mathrm{2}} =\frac{\mathrm{1}}{{a}_{{n}+\mathrm{1}} }+{a}_{{n}} \\ $$$$\Rightarrow{a}_{{n}+\mathrm{2}} {a}_{{n}+\mathrm{1}} ={a}_{{n}+\mathrm{1}} {a}_{{n}} +\mathrm{1} \\ $$$$\Rightarrow{a}_{\mathrm{2019}} {a}_{\mathrm{2018}} \\ $$$$={a}_{\mathrm{2018}} {a}_{\mathrm{2017}} +\mathrm{1} \\ $$$$={a}_{\mathrm{2017}} {a}_{\mathrm{2016}} +\mathrm{2} \\ $$$$={a}_{\mathrm{1}} {a}_{\mathrm{2}} +\mathrm{2017} \\ $$$$=\mathrm{2018} \\ $$$$\Rightarrow{a}_{{n}+\mathrm{1}} {a}_{{n}} ={n} \\ $$$$\frac{{a}_{\mathrm{2019}} }{{a}_{\mathrm{2017}} }=\frac{\mathrm{2018}}{\mathrm{2017}} \\ $$$$\frac{{a}_{\mathrm{2017}} }{{a}_{\mathrm{2015}} }=\frac{\mathrm{2016}}{\mathrm{2015}} \\ $$$$\frac{{a}_{\mathrm{5}} }{{a}_{\mathrm{3}} }=\frac{\mathrm{4}}{\mathrm{3}} \\ $$$$\frac{{a}_{\mathrm{3}} }{{a}_{\mathrm{1}} }=\frac{\mathrm{2}}{\mathrm{1}} \\ $$$$\Rightarrow{a}_{\mathrm{2019}} ={a}_{\mathrm{1}} ×\frac{\mathrm{2}×\mathrm{4}×\centerdot\centerdot\centerdot×\mathrm{2018}}{\mathrm{1}×\mathrm{3}×\centerdot\centerdot\centerdot×\mathrm{2017}} \\ $$$$=\frac{\mathrm{2018}!!}{\mathrm{2017}!!} \\ $$
Commented by Master last updated on 28/Dec/19
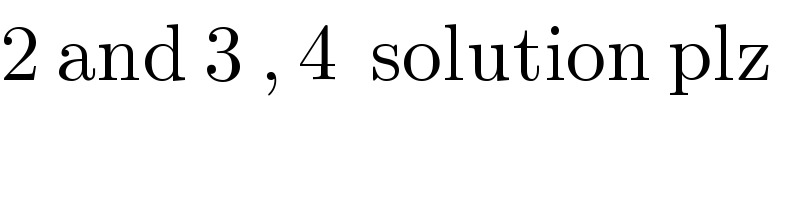
2 and 3 , 4 solution plz
$$\mathrm{2}\:\mathrm{and}\:\mathrm{3}\:,\:\mathrm{4}\:\:\mathrm{solution}\:\mathrm{plz} \\ $$
Commented by Master last updated on 28/Dec/19
thanks
$$\mathrm{thanks} \\ $$
Commented by MJS last updated on 28/Dec/19
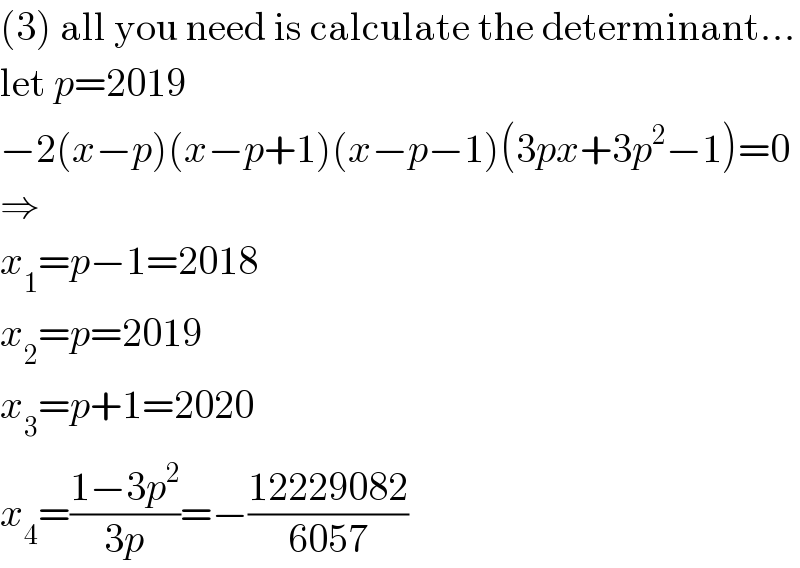
(3) all you need is calculate the determinant... let p=2019 −2(x−p)(x−p+1)(x−p−1)(3px+3p^2 −1)=0 ⇒ x_1 =p−1=2018 x_2 =p=2019 x_3 =p+1=2020 x_4 =((1−3p^2 )/(3p))=−((12229082)/(6057))
$$\left(\mathrm{3}\right)\:\mathrm{all}\:\mathrm{you}\:\mathrm{need}\:\mathrm{is}\:\mathrm{calculate}\:\mathrm{the}\:\mathrm{determinant}… \\ $$$$\mathrm{let}\:{p}=\mathrm{2019} \\ $$$$−\mathrm{2}\left({x}−{p}\right)\left({x}−{p}+\mathrm{1}\right)\left({x}−{p}−\mathrm{1}\right)\left(\mathrm{3}{px}+\mathrm{3}{p}^{\mathrm{2}} −\mathrm{1}\right)=\mathrm{0} \\ $$$$\Rightarrow \\ $$$${x}_{\mathrm{1}} ={p}−\mathrm{1}=\mathrm{2018} \\ $$$${x}_{\mathrm{2}} ={p}=\mathrm{2019} \\ $$$${x}_{\mathrm{3}} ={p}+\mathrm{1}=\mathrm{2020} \\ $$$${x}_{\mathrm{4}} =\frac{\mathrm{1}−\mathrm{3}{p}^{\mathrm{2}} }{\mathrm{3}{p}}=−\frac{\mathrm{12229082}}{\mathrm{6057}} \\ $$
Answered by benjo 1/2 santuyy last updated on 29/Dec/19
(4) f(0)=4 →c=4 0≤f(x)≤4 for 0≤x≤3 → ax^2 +bx+4≤4 , x(ax+b)≤0 , b must be negatif so we has 0≤x≤−(b/a) . we getting −(b/a)=3 → b =−3a , a >0 ■
$$\left(\mathrm{4}\right)\:{f}\left(\mathrm{0}\right)=\mathrm{4}\:\rightarrow{c}=\mathrm{4} \\ $$$$\mathrm{0}\leqslant{f}\left({x}\right)\leqslant\mathrm{4}\:{for}\:\mathrm{0}\leqslant{x}\leqslant\mathrm{3}\:\rightarrow \\ $$$${ax}^{\mathrm{2}} \:+{bx}+\mathrm{4}\leqslant\mathrm{4}\:,\: \\ $$$${x}\left({ax}+{b}\right)\leqslant\mathrm{0}\:,\:{b}\:{must}\:{be}\:{negatif} \\ $$$${so}\:{we}\:{has}\:\mathrm{0}\leqslant{x}\leqslant−\left({b}/{a}\right)\:.\: \\ $$$${we}\:{getting}\:−\left({b}/{a}\right)=\mathrm{3}\:\rightarrow\:{b}\:=−\mathrm{3}{a}\:,\:{a}\:>\mathrm{0}\:\blacksquare \\ $$$$ \\ $$

Pin

Leave a Reply

Your email address will not be published. Required fields are marked *