Question Number 77725 by BK last updated on 09/Jan/20

Commented by mr W last updated on 09/Jan/20
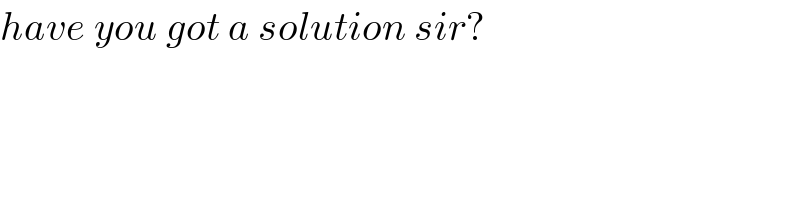
$${have}\:{you}\:{got}\:{a}\:{solution}\:{sir}? \\ $$
Commented by abdomathmax last updated on 09/Jan/20
![let A=ln(e+ln(e+ln(e+...)) ⇒ e^A =e +ln(e+ln(e+...) =e +A ⇒ e^A −A−e=0 let f(x)=e^x −x−e with x>0 we have f(0)=1−e and lim_(x→+∞) f(x)=+∞ f^′ (x)=e^x −1>0 for x>0 ⇒f is increasing on ]0,+∞[ varistion of f(x) x 0 +∞ f^′ (x) + f(x) 1−e inc. +∞ f(0)<0 ⇒ ∃ x_0 ∈]0,+∞ [ / f(x_0 )=0 f(1)=−1 and f(2)=e^2 −2−e >0 ⇒x_0 ∈]1,2[ we can find x_0 by iteration (newton method) u_0 =(3/2) and u_(n+1) =u_n −((f(u_n ))/(f^′ (u_n )))](https://www.tinkutara.com/question/Q77728.png)
$${let}\:{A}={ln}\left({e}+{ln}\left({e}+{ln}\left({e}+…\right)\right)\:\Rightarrow\right. \\ $$$${e}^{{A}} ={e}\:+{ln}\left({e}+{ln}\left({e}+…\right)\:={e}\:+{A}\:\Rightarrow\right. \\ $$$${e}^{{A}} −{A}−{e}=\mathrm{0}\:{let}\:\:{f}\left({x}\right)={e}^{{x}} −{x}−{e}\:\:\:{with}\:{x}>\mathrm{0} \\ $$$${we}\:{have}\:{f}\left(\mathrm{0}\right)=\mathrm{1}−{e}\:\:{and}\:{lim}_{{x}\rightarrow+\infty} {f}\left({x}\right)=+\infty \\ $$$${f}^{'} \left({x}\right)={e}^{{x}} −\mathrm{1}>\mathrm{0}\:{for}\:{x}>\mathrm{0}\:\Rightarrow{f}\:{is}\:{increasing}\:{on} \\ $$$$\left.\right]\mathrm{0},+\infty\left[\:\:\:{varistion}\:{of}\:{f}\left({x}\right)\right. \\ $$$${x}\:\:\:\:\:\:\:\:\mathrm{0}\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:+\infty \\ $$$${f}^{'} \left({x}\right)\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:+ \\ $$$${f}\left({x}\right)\:\:\:\:\mathrm{1}−{e}\:\:\:\:\:\:\:\:{inc}.\:\:+\infty \\ $$$$\left.{f}\left(\mathrm{0}\right)<\mathrm{0}\:\Rightarrow\:\exists\:{x}_{\mathrm{0}} \in\right]\mathrm{0},+\infty\:\left[\:\:\:\:/\:{f}\left({x}_{\mathrm{0}} \right)=\mathrm{0}\right. \\ $$$$\left.{f}\left(\mathrm{1}\right)=−\mathrm{1}\:\:{and}\:{f}\left(\mathrm{2}\right)={e}^{\mathrm{2}} −\mathrm{2}−{e}\:>\mathrm{0}\:\Rightarrow{x}_{\mathrm{0}} \:\in\right]\mathrm{1},\mathrm{2}\left[\right. \\ $$$${we}\:{can}\:{find}\:{x}_{\mathrm{0}} \:{by}\:{iteration}\:\left({newton}\:{method}\right) \\ $$$${u}_{\mathrm{0}} =\frac{\mathrm{3}}{\mathrm{2}}\:{and}\:\:{u}_{{n}+\mathrm{1}} ={u}_{{n}} −\frac{{f}\left({u}_{{n}} \right)}{{f}^{'} \left({u}_{{n}} \right)} \\ $$
Commented by BK last updated on 09/Jan/20

$$\mathrm{no}\:\mathrm{answer} \\ $$
Commented by msup trace by abdo last updated on 09/Jan/20

$${study}\:{the}\:{numerical}\:{analysis} \\ $$$${first}\:{instead}\:{of}\:{saying}\:{no}\:{answer} \\ $$$$… \\ $$
Answered by MJS last updated on 09/Jan/20

$${x}\approx−\mathrm{2}.\mathrm{64745}\vee{x}\approx\mathrm{1}.\mathrm{42037} \\ $$
Answered by mr W last updated on 09/Jan/20
![x=ln (e+ln (e+ln (e+...))) x=ln (e+x) e^x =x+e e^x e^e =(x+e)e^e e^(x+e) =(x+e)e^e (x+e)e^(−(x+e)) e^e =1 −(x+e)e^(−(x+e)) =−(1/e^e ) −(x+e)=W(−(1/e^e )) ⇒x=−[e+W(−(1/e^e ))]≈−[e+ { ((−4.138651946)),((−0.070831586)) :}]= { ((1.42037)),((−2.64745)) :}](https://www.tinkutara.com/question/Q77735.png)
$${x}=\mathrm{ln}\:\left({e}+\mathrm{ln}\:\left({e}+\mathrm{ln}\:\left({e}+…\right)\right)\right) \\ $$$${x}=\mathrm{ln}\:\left({e}+{x}\right) \\ $$$${e}^{{x}} ={x}+{e} \\ $$$${e}^{{x}} {e}^{{e}} =\left({x}+{e}\right){e}^{{e}} \\ $$$${e}^{{x}+{e}} =\left({x}+{e}\right){e}^{{e}} \\ $$$$\left({x}+{e}\right){e}^{−\left({x}+{e}\right)} {e}^{{e}} =\mathrm{1} \\ $$$$−\left({x}+{e}\right){e}^{−\left({x}+{e}\right)} =−\frac{\mathrm{1}}{{e}^{{e}} } \\ $$$$−\left({x}+{e}\right)=\mathbb{W}\left(−\frac{\mathrm{1}}{{e}^{{e}} }\right) \\ $$$$\Rightarrow{x}=−\left[{e}+\mathbb{W}\left(−\frac{\mathrm{1}}{{e}^{{e}} }\right)\right]\approx−\left[{e}+\begin{cases}{−\mathrm{4}.\mathrm{138651946}}\\{−\mathrm{0}.\mathrm{070831586}}\end{cases}\right]=\begin{cases}{\mathrm{1}.\mathrm{42037}}\\{−\mathrm{2}.\mathrm{64745}}\end{cases} \\ $$
Commented by BK last updated on 09/Jan/20

$$\mathrm{thanks} \\ $$
Commented by MJS last updated on 09/Jan/20
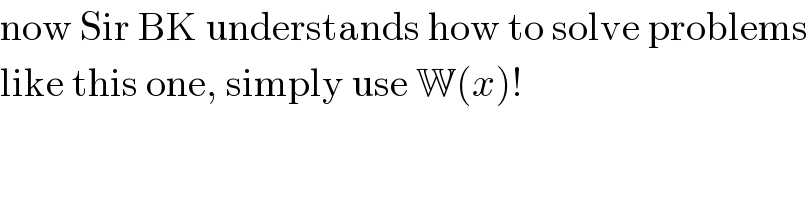
$$\mathrm{now}\:\mathrm{Sir}\:\mathrm{BK}\:\mathrm{understands}\:\mathrm{how}\:\mathrm{to}\:\mathrm{solve}\:\mathrm{problems} \\ $$$$\mathrm{like}\:\mathrm{this}\:\mathrm{one},\:\mathrm{simply}\:\mathrm{use}\:\mathbb{W}\left({x}\right)! \\ $$
