Menu Close

Question-7945

Tinku Tara 0 Comments
Pin




Question Number 7945 by Rasheed Soomro last updated on 25/Sep/16
Commented by Rasheed Soomro last updated on 25/Sep/16
In above figure AF ,a diameter of the circle with centre G has been trisected at B & D (i-e AB=BD=DF) Is there any point H on the circle for which ∠AHB=∠BHD=∠DHF I-E β=γ=δ ? If yes then what is ∠HGF?
$${In}\:{above}\:{figure}\:{AF}\:,{a}\:{diameter}\:{of}\:{the} \\ $$$${circle}\:{with}\:{centre}\:{G}\:{has}\:{been}\:{trisected} \\ $$$${at}\:{B}\:\&\:{D}\:\left({i}-{e}\:{AB}={BD}={DF}\right) \\ $$$${Is}\:{there}\:{any}\:{point}\:{H}\:{on}\:{the}\:{circle}\:{for} \\ $$$${which}\: \\ $$$$\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\angle{AHB}=\angle{BHD}=\angle{DHF} \\ $$$${I}-\mathcal{E}\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\beta=\gamma=\delta\:\:\:\:\:? \\ $$$${If}\:\:\:{yes}\:{then}\:{what}\:{is}\:\angle{HGF}? \\ $$
Commented by prakash jain last updated on 27/Sep/16
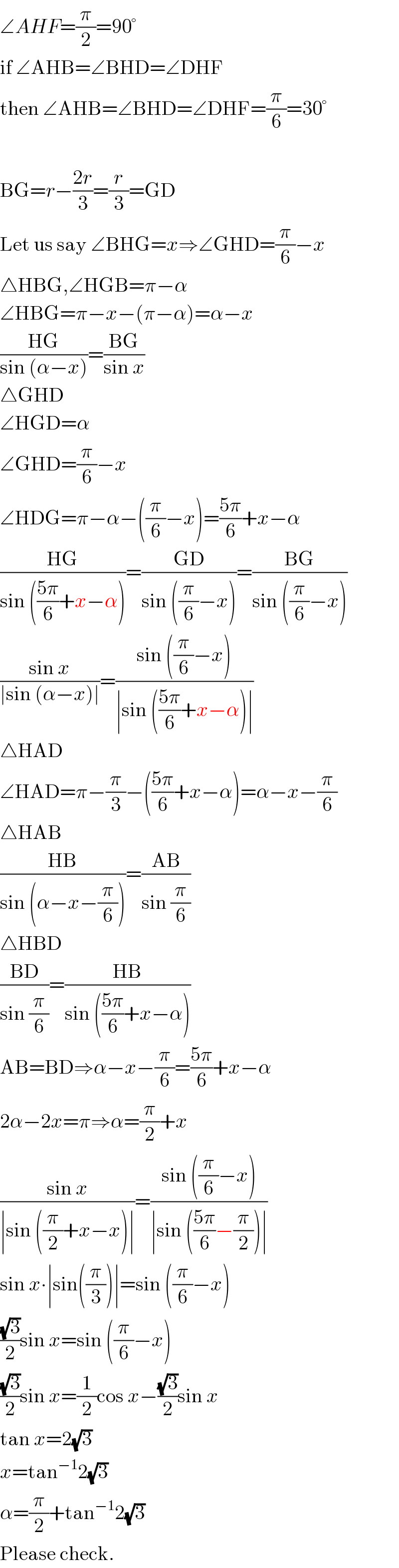
∠AHF=(π/2)=90° if ∠AHB=∠BHD=∠DHF then ∠AHB=∠BHD=∠DHF=(π/6)=30° BG=r−((2r)/3)=(r/3)=GD Let us say ∠BHG=x⇒∠GHD=(π/6)−x △HBG,∠HGB=π−α ∠HBG=π−x−(π−α)=α−x ((HG)/(sin (α−x)))=((BG)/(sin x)) △GHD ∠HGD=α ∠GHD=(π/6)−x ∠HDG=π−α−((π/6)−x)=((5π)/6)+x−α ((HG)/(sin (((5π)/6)+x−α)))=((GD)/(sin ((π/6)−x)))=((BG)/(sin ((π/6)−x))) ((sin x)/(∣sin (α−x)∣))=((sin ((π/6)−x))/(∣sin (((5π)/6)+x−α)∣)) △HAD ∠HAD=π−(π/3)−(((5π)/6)+x−α)=α−x−(π/6) △HAB ((HB)/(sin (α−x−(π/6))))=((AB)/(sin (π/6))) △HBD ((BD)/(sin (π/6)))=((HB)/(sin (((5π)/6)+x−α))) AB=BD⇒α−x−(π/6)=((5π)/6)+x−α 2α−2x=π⇒α=(π/2)+x ((sin x)/(∣sin ((π/2)+x−x)∣))=((sin ((π/6)−x))/(∣sin (((5π)/6)−(π/2))∣)) sin x∙∣sin((π/3))∣=sin ((π/6)−x) ((√3)/2)sin x=sin ((π/6)−x) ((√3)/2)sin x=(1/2)cos x−((√3)/2)sin x tan x=2(√3) x=tan^(−1) 2(√3) α=(π/2)+tan^(−1) 2(√3) Please check.
$$\angle{AHF}=\frac{\pi}{\mathrm{2}}=\mathrm{90}° \\ $$$$\mathrm{if}\:\angle\mathrm{AHB}=\angle\mathrm{BHD}=\angle\mathrm{DHF} \\ $$$$\mathrm{then}\:\angle\mathrm{AHB}=\angle\mathrm{BHD}=\angle\mathrm{DHF}=\frac{\pi}{\mathrm{6}}=\mathrm{30}° \\ $$$$ \\ $$$$\mathrm{BG}={r}−\frac{\mathrm{2}{r}}{\mathrm{3}}=\frac{{r}}{\mathrm{3}}=\mathrm{GD} \\ $$$$\mathrm{Let}\:\mathrm{us}\:\mathrm{say}\:\angle\mathrm{BHG}={x}\Rightarrow\angle\mathrm{GHD}=\frac{\pi}{\mathrm{6}}−{x} \\ $$$$\bigtriangleup\mathrm{HBG},\angle\mathrm{HGB}=\pi−\alpha \\ $$$$\angle\mathrm{HBG}=\pi−{x}−\left(\pi−\alpha\right)=\alpha−{x} \\ $$$$\frac{\mathrm{HG}}{\mathrm{sin}\:\left(\alpha−{x}\right)}=\frac{\mathrm{BG}}{\mathrm{sin}\:{x}} \\ $$$$\bigtriangleup\mathrm{GHD} \\ $$$$\angle\mathrm{HGD}=\alpha \\ $$$$\angle\mathrm{GHD}=\frac{\pi}{\mathrm{6}}−{x} \\ $$$$\angle\mathrm{HDG}=\pi−\alpha−\left(\frac{\pi}{\mathrm{6}}−{x}\right)=\frac{\mathrm{5}\pi}{\mathrm{6}}+{x}−\alpha \\ $$$$\frac{\mathrm{HG}}{\mathrm{sin}\:\left(\frac{\mathrm{5}\pi}{\mathrm{6}}+{x}−\alpha\right)}=\frac{\mathrm{GD}}{\mathrm{sin}\:\left(\frac{\pi}{\mathrm{6}}−{x}\right)}=\frac{\mathrm{BG}}{\mathrm{sin}\:\left(\frac{\pi}{\mathrm{6}}−{x}\right)} \\ $$$$\frac{\mathrm{sin}\:{x}}{\mid\mathrm{sin}\:\left(\alpha−{x}\right)\mid}=\frac{\mathrm{sin}\:\left(\frac{\pi}{\mathrm{6}}−{x}\right)}{\mid\mathrm{sin}\:\left(\frac{\mathrm{5}\pi}{\mathrm{6}}+{x}−\alpha\right)\mid} \\ $$$$\bigtriangleup\mathrm{HAD} \\ $$$$\angle\mathrm{HAD}=\pi−\frac{\pi}{\mathrm{3}}−\left(\frac{\mathrm{5}\pi}{\mathrm{6}}+{x}−\alpha\right)=\alpha−{x}−\frac{\pi}{\mathrm{6}} \\ $$$$\bigtriangleup\mathrm{HAB} \\ $$$$\frac{\mathrm{HB}}{\mathrm{sin}\:\left(\alpha−{x}−\frac{\pi}{\mathrm{6}}\right)}=\frac{\mathrm{AB}}{\mathrm{sin}\:\frac{\pi}{\mathrm{6}}} \\ $$$$\bigtriangleup\mathrm{HBD} \\ $$$$\frac{\mathrm{BD}}{\mathrm{sin}\:\frac{\pi}{\mathrm{6}}}=\frac{\mathrm{HB}}{\mathrm{sin}\:\left(\frac{\mathrm{5}\pi}{\mathrm{6}}+{x}−\alpha\right)} \\ $$$$\mathrm{AB}=\mathrm{BD}\Rightarrow\alpha−{x}−\frac{\pi}{\mathrm{6}}=\frac{\mathrm{5}\pi}{\mathrm{6}}+{x}−\alpha \\ $$$$\mathrm{2}\alpha−\mathrm{2}{x}=\pi\Rightarrow\alpha=\frac{\pi}{\mathrm{2}}+{x} \\ $$$$\frac{\mathrm{sin}\:{x}}{\mid\mathrm{sin}\:\left(\frac{\pi}{\mathrm{2}}+{x}−{x}\right)\mid}=\frac{\mathrm{sin}\:\left(\frac{\pi}{\mathrm{6}}−{x}\right)}{\mid\mathrm{sin}\:\left(\frac{\mathrm{5}\pi}{\mathrm{6}}−\frac{\pi}{\mathrm{2}}\right)\mid} \\ $$$$\mathrm{sin}\:{x}\centerdot\mid\mathrm{sin}\left(\frac{\pi}{\mathrm{3}}\right)\mid=\mathrm{sin}\:\left(\frac{\pi}{\mathrm{6}}−{x}\right) \\ $$$$\frac{\sqrt{\mathrm{3}}}{\mathrm{2}}\mathrm{sin}\:{x}=\mathrm{sin}\:\left(\frac{\pi}{\mathrm{6}}−{x}\right) \\ $$$$\frac{\sqrt{\mathrm{3}}}{\mathrm{2}}\mathrm{sin}\:{x}=\frac{\mathrm{1}}{\mathrm{2}}\mathrm{cos}\:{x}−\frac{\sqrt{\mathrm{3}}}{\mathrm{2}}\mathrm{sin}\:{x} \\ $$$$\mathrm{tan}\:{x}=\mathrm{2}\sqrt{\mathrm{3}} \\ $$$${x}=\mathrm{tan}^{−\mathrm{1}} \mathrm{2}\sqrt{\mathrm{3}} \\ $$$$\alpha=\frac{\pi}{\mathrm{2}}+\mathrm{tan}^{−\mathrm{1}} \mathrm{2}\sqrt{\mathrm{3}} \\ $$$$\mathrm{Please}\:\mathrm{check}. \\ $$
Commented by Rasheed Soomro last updated on 27/Sep/16
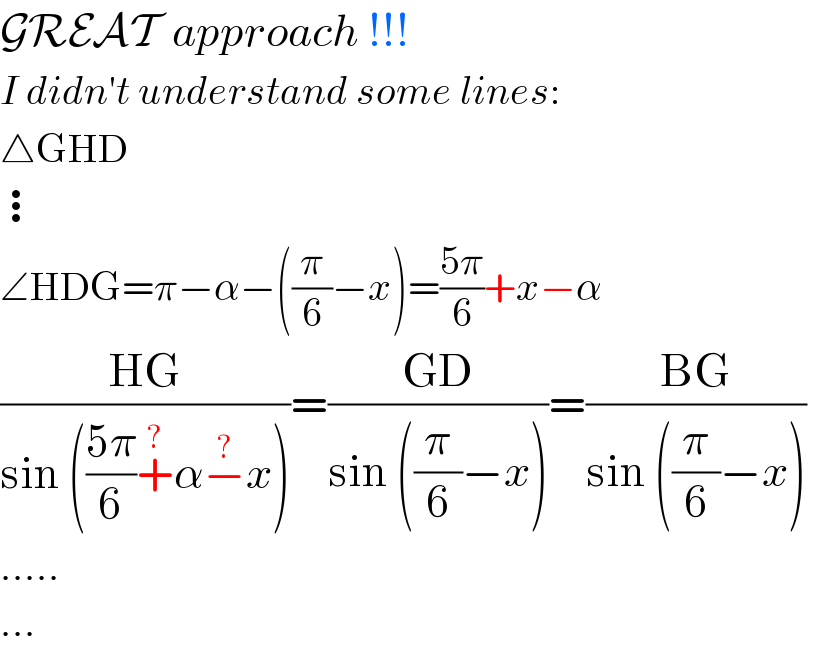
GREAT approach !!! I didn′t understand some lines: △GHD ⋮ ∠HDG=π−α−((π/6)−x)=((5π)/6)+x−α ((HG)/(sin (((5π)/6)+^(?) α−^(?) x)))=((GD)/(sin ((π/6)−x)))=((BG)/(sin ((π/6)−x))) ..... ...
$$\mathcal{GREAT}\:\:{approach}\:!!! \\ $$$${I}\:{didn}'{t}\:{understand}\:{some}\:{lines}: \\ $$$$\bigtriangleup\mathrm{GHD} \\ $$$$\vdots \\ $$$$\angle\mathrm{HDG}=\pi−\alpha−\left(\frac{\pi}{\mathrm{6}}−{x}\right)=\frac{\mathrm{5}\pi}{\mathrm{6}}+{x}−\alpha \\ $$$$\frac{\mathrm{HG}}{\mathrm{sin}\:\left(\frac{\mathrm{5}\pi}{\mathrm{6}}\overset{?} {+}\alpha\overset{?} {−}{x}\right)}=\frac{\mathrm{GD}}{\mathrm{sin}\:\left(\frac{\pi}{\mathrm{6}}−{x}\right)}=\frac{\mathrm{BG}}{\mathrm{sin}\:\left(\frac{\pi}{\mathrm{6}}−{x}\right)}\:\: \\ $$$$….. \\ $$$$… \\ $$
Commented by prakash jain last updated on 27/Sep/16
Yes. My mistake. I will correct.
$$\mathrm{Yes}.\:\mathrm{My}\:\mathrm{mistake}.\:\mathrm{I}\:\mathrm{will}\:\mathrm{correct}. \\ $$
Answered by prakash jain last updated on 02/Oct/16
In comments.
$$\mathrm{In}\:\mathrm{comments}. \\ $$

Pin

Leave a Reply

Your email address will not be published. Required fields are marked *