Question-9085

Question Number 9085 by tawakalitu last updated on 17/Nov/16

Answered by mrW last updated on 17/Nov/16

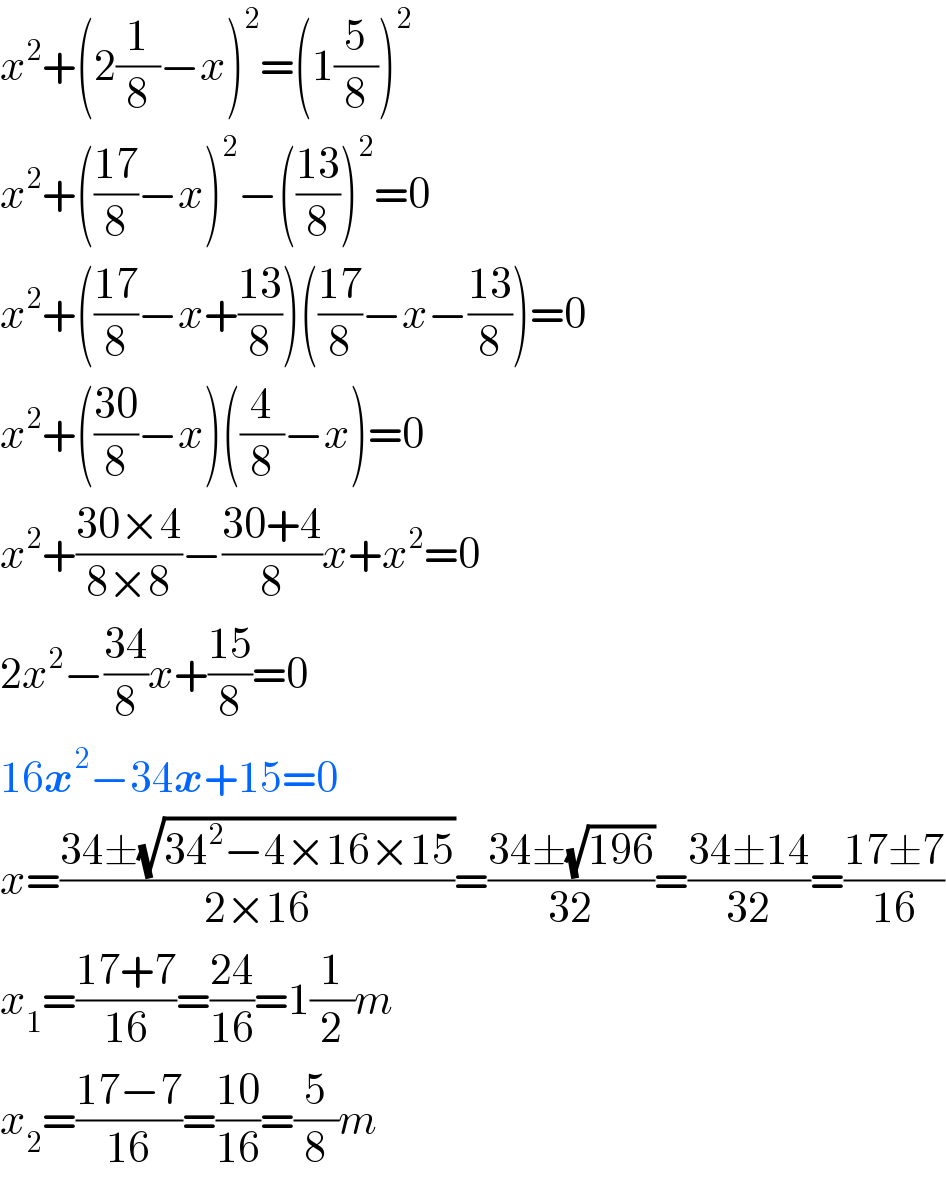
x^2 +(2(1/8)−x)^2 =(1(5/8))^2 x^2 +(((17)/8)−x)^2 −(((13)/8))^2 =0 x^2 +(((17)/8)−x+((13)/8))(((17)/8)−x−((13)/8))=0 x^2 +(((30)/8)−x)((4/8)−x)=0 x^2 +((30×4)/(8×8))−((30+4)/8)x+x^2 =0 2x^2 −((34)/8)x+((15)/8)=0 16x^2 −34x+15=0 x=((34±(√(34^2 −4×16×15)))/(2×16))=((34±(√(196)))/(32))=((34±14)/(32))=((17±7)/(16)) x_1 =((17+7)/(16))=((24)/(16))=1(1/2)m x_2 =((17−7)/(16))=((10)/(16))=(5/8)m

$${x}^{\mathrm{2}} +\left(\mathrm{2}\frac{\mathrm{1}}{\mathrm{8}}−{x}\right)^{\mathrm{2}} =\left(\mathrm{1}\frac{\mathrm{5}}{\mathrm{8}}\right)^{\mathrm{2}} \\ $$$${x}^{\mathrm{2}} +\left(\frac{\mathrm{17}}{\mathrm{8}}−{x}\right)^{\mathrm{2}} −\left(\frac{\mathrm{13}}{\mathrm{8}}\right)^{\mathrm{2}} =\mathrm{0} \\ $$$${x}^{\mathrm{2}} +\left(\frac{\mathrm{17}}{\mathrm{8}}−{x}+\frac{\mathrm{13}}{\mathrm{8}}\right)\left(\frac{\mathrm{17}}{\mathrm{8}}−{x}−\frac{\mathrm{13}}{\mathrm{8}}\right)=\mathrm{0} \\ $$$${x}^{\mathrm{2}} +\left(\frac{\mathrm{30}}{\mathrm{8}}−{x}\right)\left(\frac{\mathrm{4}}{\mathrm{8}}−{x}\right)=\mathrm{0} \\ $$$${x}^{\mathrm{2}} +\frac{\mathrm{30}×\mathrm{4}}{\mathrm{8}×\mathrm{8}}−\frac{\mathrm{30}+\mathrm{4}}{\mathrm{8}}{x}+{x}^{\mathrm{2}} =\mathrm{0} \\ $$$$\mathrm{2}{x}^{\mathrm{2}} −\frac{\mathrm{34}}{\mathrm{8}}{x}+\frac{\mathrm{15}}{\mathrm{8}}=\mathrm{0} \\ $$$$\mathrm{16}\boldsymbol{{x}}^{\mathrm{2}} −\mathrm{34}\boldsymbol{{x}}+\mathrm{15}=\mathrm{0} \\ $$$${x}=\frac{\mathrm{34}\pm\sqrt{\mathrm{34}^{\mathrm{2}} −\mathrm{4}×\mathrm{16}×\mathrm{15}}}{\mathrm{2}×\mathrm{16}}=\frac{\mathrm{34}\pm\sqrt{\mathrm{196}}}{\mathrm{32}}=\frac{\mathrm{34}\pm\mathrm{14}}{\mathrm{32}}=\frac{\mathrm{17}\pm\mathrm{7}}{\mathrm{16}} \\ $$$${x}_{\mathrm{1}} =\frac{\mathrm{17}+\mathrm{7}}{\mathrm{16}}=\frac{\mathrm{24}}{\mathrm{16}}=\mathrm{1}\frac{\mathrm{1}}{\mathrm{2}}{m} \\ $$$${x}_{\mathrm{2}} =\frac{\mathrm{17}−\mathrm{7}}{\mathrm{16}}=\frac{\mathrm{10}}{\mathrm{16}}=\frac{\mathrm{5}}{\mathrm{8}}{m} \\ $$

Commented by tawakalitu last updated on 17/Nov/16

Thank you sir. i really appreciate. God bless you.

$$\mathrm{Thank}\:\mathrm{you}\:\mathrm{sir}.\:\mathrm{i}\:\mathrm{really}\:\mathrm{appreciate}.\:\mathrm{God} \\ $$$$\mathrm{bless}\:\mathrm{you}. \\ $$

Facebook Tweet Pin

Leave a Reply Cancel reply